Quiz Questions On Algebra 2 Honors
Algebra is a fun to learn mathematical concept that most students fear to dive into. If you are taking your algebra two honors the quiz below is designed to help you pass your assessment tests with ease. Give it a try and see just how prepared you are for the finals. All the best!
- 1.
Liam is has a rectangular sandbox that he is building for his younger brother. The length of the sandbox is 3 times the width. If he builds a grassy area 3 feet out on every side, what would the area of the new rectangle be (including the grassy area) in terms of w, the width?
- A.
3w2 + 12w + 9
- B.
3w2 + 24w + 36
- C.
3w2 - 12w + 9
- D.
3w2 - 24w + 36
- E.
None of the above
Correct Answer
B. 3w2 + 24w + 36Explanation
(3w + 6)(w + 6)Rate this question:
-
- 2.
Victoria is building a rectangular pen using an existing side of her house (so her pen only needs three sides of fencing.) If she has 160 feet of fencing, what would be dimensions that would yield the maximum area? Use x to represent how far out from the house her pen extends.
- A.
30 feet out from house, 100 feet along the house
- B.
53.3 feet out from house, 53.3 feet along the house
- C.
60 feet out from house, 40 feet along the house
- D.
40 feet out from house, 80 feet along the house
- E.
20 feet out from house, 120 feet along the house
- F.
75 feet out from house, 30 feet along the house
Correct Answer
D. 40 feet out from house, 80 feet along the houseExplanation
A = x(160 - 2x) = -2x^2 + 160x. Vertex occurs at x = 40 feet, which means 160-2x = 80 feet. 40*80 = 3200 sq feet.Rate this question:
-
- 3.
Factor completely:4x2 - 81y2
Correct Answer
(2x + 9y)(2x - 9y)
(2x+9y)(2x-9y)
(2x-9y)(2x+9y)
(2x - 9y)(2x + 9y)Explanation
difference of squaresRate this question:
- 4.
Factor completely9a2 + 12ab + 4b2
Correct Answer
(3a + 2b)^2
(3a + 2b)(3a + 2b)
(3a+2b)(3a+2b)
(3a+2b)^2Explanation
The given expression, 9a^2 + 12ab + 4b^2, can be factored as (3a + 2b)^2. This is because the expression follows the pattern of a perfect square trinomial, where the first and last terms are perfect squares and the middle term is twice the product of the square roots of the first and last terms. In this case, the first term is (3a)^2 = 9a^2, the last term is (2b)^2 = 4b^2, and the middle term is 2(3a)(2b) = 12ab. Therefore, the expression can be factored as (3a + 2b)^2.Rate this question:
- 5.
Factor completely:125m3 + 27n3Use the ^ key to indicate a power.
Correct Answer
(5m + 3n)(25m^2 - 15mn + 9n^2)
(5m+3n)(25m^2-15mn+9n^2)Explanation
Sum of cubesRate this question:
- 6.
Factor completely:x4 - 625y4
Correct Answer
(x^2+25y^2)(x+5y)(x-5y)
(x^2 + 25y^2)(x + 5y)(x - 5y)
(x^2+25y^2)(x-5y)(x+5y)
(x^2 + 25y^2)(x - 5y)(x + 5y)Explanation
The given expression is a difference of squares, where x^4 is the square of (x^2) and 625y^4 is the square of (25y^2). Therefore, we can factor it using the formula for the difference of squares, which is (a^2 - b^2) = (a + b)(a - b). Applying this formula, we get (x^2 + 25y^2)(x + 5y)(x - 5y).Rate this question:
- 7.
Factor completely:10x3 - 12x2 - 16x
Correct Answer
2x(5x+4)(x-2)
2x(5x + 4)(x - 2)
2x(x-2)(5x+4)
2x(x - 2)(5x + 4)Explanation
The given expression can be factored completely as 2x(5x+4)(x-2). This can be done by finding the greatest common factor of the terms, which is 2x. Then, using the distributive property, we can factor out 2x from each term. This leaves us with (5x+4)(x-2), which cannot be factored any further. Therefore, the correct answer is 2x(5x+4)(x-2).Rate this question:
- 8.
Solve the following quadratic equation:5x(x - 2) - 2(x + 1) = x - 8Write answers as x = ____ and x = ______
Correct Answer
x = 3/5 and x = 2
x = 0.6 and x = 2
x = 2 and x = 0.6
x = 2 and x = 3/5Explanation
To solve the quadratic equation, we first need to simplify the equation by expanding and combining like terms.
Expanding the equation, we get:
5x^2 - 10x - 2x - 2 = x - 8
Combining like terms, we get:
5x^2 - 12x - 2 = x - 8
Next, we move all terms to one side of the equation to set it equal to zero:
5x^2 - 12x - x + 6 = 0
Combining like terms again, we get:
5x^2 - 13x + 6 = 0
Now, we can factorize the quadratic equation:
(5x - 2)(x - 3) = 0
Setting each factor equal to zero, we get two possible solutions:
5x - 2 = 0 --> x = 2/5
x - 3 = 0 --> x = 3
Therefore, the correct answers are x = 3/5 and x = 2.Rate this question:
- 9.
The path of a ball is given by the equation h(t) = -16t2 + 64t, where t is the time in seconds after the ball is hit in the air and h(t) is the height of the ball in feet at a given time, t.After how many seconds does the ball attain a maximum height?
Correct Answer
2
2 secondsExplanation
The ball attains its maximum height when its vertical velocity becomes zero. In this case, the equation h(t) = -16t^2 + 64t represents a parabolic path, where the coefficient of the t^2 term is negative. This means that the parabola opens downward and has a maximum point. To find the time at which the ball reaches its maximum height, we need to find the vertex of the parabola. The vertex of a parabola in the form h(t) = at^2 + bt + c is given by the formula t = -b/2a. In this case, a = -16 and b = 64, so t = -64/(2*(-16)) = 2 seconds. Therefore, after 2 seconds, the ball attains its maximum height.Rate this question:
- 10.
Write the following parabola in vertex form:h(x) = 0.5x2 + 5x + 1Use decimals to enter your answer and ^ for the exponent.
Correct Answer
h(x) = 0.5(x + 5)^2 - 11.5
h(x)=0.5(x+5)^2-11.5Explanation
The given answer is correct because it represents the parabola in vertex form. The vertex form of a parabola is given by h(x) = a(x - h)^2 + k, where (h, k) represents the coordinates of the vertex. In this case, the vertex form is h(x) = 0.5(x + 5)^2 - 11.5, which indicates that the vertex is at (-5, -11.5). The answer also correctly includes the coefficient of 0.5, which determines the shape of the parabola.Rate this question:
- 11.
Write the following parabola in vertex form:y = -6x2 - 36x - 10
- A.
Y = -6(x + 6)^2 + 26
- B.
Y = -6(x - 6)^2 + 26
- C.
Y = -6(x - 3)^2 + 44
- D.
Y = -6(x + 3)^2 - 1
- E.
Y = -6(x - 3)^2 - 1
Correct Answer
C. Y = -6(x - 3)^2 + 44Explanation
The given equation is in the form y = ax^2 + bx + c. To rewrite it in vertex form, we need to complete the square. We can do this by factoring out the common factor of -6 from the terms involving x, which gives us y = -6(x^2 + 6x) - 10. Then, we can add and subtract the square of half the coefficient of x (which is 3) inside the parentheses, giving us y = -6(x^2 + 6x + 9 - 9) - 10. Simplifying further, we have y = -6((x + 3)^2 - 9) - 10. Expanding the square, we get y = -6(x + 3)^2 + 54 - 10. Combining like terms, we have y = -6(x + 3)^2 + 44, which matches the given answer.Rate this question:
-
- 12.
Solve the following quadratic equation. Give the answer in simplest radical form.4x2 + 8x + 1 = 0enter answer as (_____ +- sqrt____)/_____
Correct Answer
(-2+-sqrt3)/2
(-2 +- sqrt3)/2
(-2 +- sqrt(3))/2Explanation
The given quadratic equation is 4x^2 + 8x + 1 = 0. To solve this equation, we can use the quadratic formula which states that for an equation ax^2 + bx + c = 0, the solutions are given by x = (-b +- sqrt(b^2 - 4ac))/(2a). In this case, a = 4, b = 8, and c = 1. Plugging these values into the quadratic formula, we get x = (-8 +- sqrt(8^2 - 4(4)(1)))/(2(4)). Simplifying further, we have x = (-2 +- sqrt(3))/2. Therefore, the answer is (-2+-sqrt3)/2, (-2 +- sqrt3)/2, and (-2 +- sqrt(3))/2.Rate this question:
- 13.
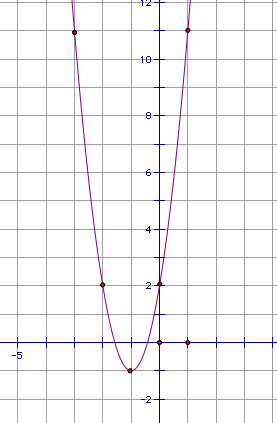
Write the equation of the following parabola:
- A.
Y = 3(x + 1)^2 - 1
- B.
Y = (x + 1)^2 - 1
- C.
Y = (x - 1)^2 + 1
- D.
Y = 3(x - 1)^2 - 1
- E.
Y = -3(x + 1)^2 - 1
Correct Answer
A. Y = 3(x + 1)^2 - 1Explanation
vertex = (-1, -1) y = a(x + 1)^2 - 1. Use another point to find a (for example, the point (0, 2)).Rate this question:
-
Quiz Review Timeline +
Our quizzes are rigorously reviewed, monitored and continuously updated by our expert board to maintain accuracy, relevance, and timeliness.
-
Current Version
-
Mar 21, 2023Quiz Edited by
ProProfs Editorial Team -
Oct 19, 2010Quiz Created by
Annacabral
- Calculus Quizzes
- Data Handling Quizzes
- Decimal Quizzes
- Discrete Mathematics Quizzes
- Function Quizzes
- Geometry Quizzes
- Graph Quizzes
- Measurement Quizzes
- Multiplication Quizzes
- Number Quizzes
- Percentage Quizzes
- Place Value Quizzes
- Probability Quizzes
- Problem Solving Quizzes
- Ratio Quizzes
- Shape Quizzes
- Statistics Quizzes
- Trigonometry Quizzes
 Back to top
Back to top


