Calculus Quiz Questions And Answers
-
What is the derivative of x² + 1 with respect to x?
-
2x
-
X
-
X+1
-
2x+1
-
About This Quiz
This Calculus Quiz is designed to challenge your grasp of key concepts, theories, and problem-solving techniques in differential and integral calculus. Each question is crafted to assess your analytical thinking and mathematical reasoning, helping you identify areas of strength and improvement.
This calculus test ensures a focus on fundamental principles without unnecessary complexity. It is a great way See moreto prepare for exams, improve your skills, or simply challenge yourself in a structured way. Sharpen your calculus abilities and gain confidence in your mathematical journey. Let's see how well you score on this essential calculus knowledge test!
.webp "Calculus Quiz Questions And Answers - Quiz")
Quiz Preview
- 2.
What is the derivative of the function f(x) = 1963?
-
0
-
1963
-
−∞
-
None of the above
Correct Answer
A. 0Explanation
The derivative of a constant function is always 0. In this case, the function f(x) = 1963 is a constant because it does not depend on x. The derivative of a constant function is 0, as there is no change in the value of the function as x changes. The derivative measures the rate of change, and since there is no change in the function, the rate of change is zero. Thus, the derivative of f(x) = 1963 with respect to x is 0.Rate this question:
-
- 3.
What is the integral of 1 with respect to x?
-
0.5x
-
X + C
-
1.25x
-
2x
Correct Answer
A. X + CExplanation
The integral of 1 with respect to x is x + C, where C is the constant of integration. The integral of a constant, such as 1, with respect to a variable, in this case, x, is simply the constant multiplied by x. Therefore, the integral of 1 with respect to x results in x. The constant C represents an arbitrary constant that can be added to the result because there are infinitely many antiderivatives of a function. This is the fundamental concept in indefinite integration.Rate this question:
-
- 4.
State whether the given statement is true or false. The second fundamental theorem of calculus states that if F(x) = ∫ ax f(t) dt then F '(x) = f(x).
-
True
-
False
Correct Answer
A. TrueExplanation
The second fundamental theorem of calculus states that if F(x) is defined as the integral of a function f(t) with respect to t from a constant a to a variable x, then the derivative of F(x) with respect to x is equal to the integrand f(x). This theorem establishes a connection between differentiation and integration. It shows that the derivative of the accumulated area under the curve described by f(t) from a to x is simply the function value at x, making differentiation and integration inverse operations.Rate this question:
-
- 5.
What is the derivative of x² + 1 with respect to y?
-
2x
-
Xy +1
-
0
-
(x+1)y
Correct Answer
A. 0Explanation
The expression x² + 1 does not contain the variable y. When differentiating with respect to a variable that does not appear in the expression, such as y, the result is always 0. The derivative represents the rate of change of the function with respect to the variable, and if the function does not depend on that variable, the rate of change is zero. Therefore, the derivative of x² + 1 with respect to y is 0, as there is no change in the function with respect to y.Rate this question:
-
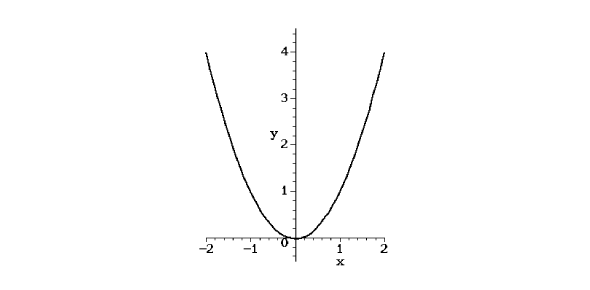
- 6.
Which function, when integrated, produces the graph shown?
-
-x+1
-
X-1
-
(x+1)
-
2x
Correct Answer
A. 2xExplanation
The graph described is a straight line with a positive slope, passing through the origin. The function that produces such a graph when integrated is 2x. When you integrate 2x with respect to x, the result is x², which is a parabolic graph. A linear function with a positive slope like 2x produces a graph where the value of the function increases steadily as x increases. Thus, integrating 2x produces a graph that matches the description of a straight line with a positive slope.Rate this question:
-
- 7.
Who are the two men credited with the discovery of calculus?
-
Newton
-
Gottfried Wilhelm Leibniz
-
Thomas Edison
-
Georg Cantor
Correct Answer(s)
A. Newton
A. Gottfried Wilhelm LeibnizExplanation
Isaac Newton and Gottfried Wilhelm Leibniz are both credited with independently developing the field of calculus. Newton, an English mathematician and physicist, developed calculus in the context of his work on physics and motion. Leibniz, a German mathematician, developed calculus using a different notation, which is still in use today. Though their discoveries were separate, both men’s contributions were crucial to the development of calculus. The dispute over who invented calculus first led to significant debates, but both Newton and Leibniz's work remains fundamental in mathematics.Rate this question:
-
- 8.
What is the derivative of f(x) = 3x² + 4x - 1 with respect to x?
-
6x + 4
-
3x^2 + 4x
-
6x + 4x - 1
-
6x - 4
Correct Answer
A. 6x + 4Explanation
To find the derivative of f(x) = 3x² + 4x - 1, apply the power rule. The derivative of 3x² is 6x (because 2 * 3 = 6). The derivative of 4x is 4 (since the derivative of x is 1). The constant term -1 has a derivative of 0. Combining these, the derivative of the function is 6x + 4. This represents the rate of change of the function f(x) = 3x² + 4x - 1 with respect to x, indicating how the function behaves as x changes.Rate this question:
-
- 9.
What is the limit of 1/(x - 1) as x approaches infinity?
-
Infinity
-
0
-
-1
-
Negative infinity
Correct Answer
A. 0Explanation
As x approaches infinity, the expression 1/(x - 1) behaves in such a way that the denominator becomes very large, making the entire fraction approach zero. As x increases, the term (x - 1) grows larger, and since the numerator remains constant at 1, the fraction becomes smaller and smaller. Therefore, the limit of 1/(x - 1) as x approaches infinity is 0. This is a standard result for rational functions where the degree of the denominator exceeds that of the numerator.Rate this question:
-
- 10.
Does the limit of the expression 'x - 1' exist as x approaches zero?
-
True
-
False
Correct Answer
A. TrueExplanation
The limit of the expression 'x - 1' as x approaches zero does exist. As x gets closer to zero, the expression x - 1 simply approaches -1. The limit describes the value that a function approaches as the input (x) approaches a particular value. In this case, as x approaches 0, the expression 'x - 1' approaches -1, so the limit exists and equals -1. Since there are no discontinuities or undefined points in this expression, the limit is well-defined and exists as x approaches zero.Rate this question:
-
- 11.
What is the derivative of x³ with respect to x?
-
3x²
-
2x³
-
3x² + 1
-
X²
Correct Answer
A. 3x²Explanation
The derivative of x³ with respect to x is found using the power rule, which states that the derivative of xⁿ is n*xⁿ⁻¹. For x³, n = 3. Applying the power rule, the derivative is 3x². This means that as x increases, the rate of change of the function x³ is proportional to 3x². The derivative provides information about how steep the graph of x³ is at any point. The slope of the curve increases as x moves away from zero, with the function increasing more rapidly for larger values of x.Rate this question:
-
- 12.
What is the integral of 2x with respect to x?
-
X² + C
-
X²
-
2x + C
-
2x² + C
Correct Answer
A. X² + CExplanation
The integral of 2x with respect to x is solved by applying the power rule for integration, which states that the integral of xⁿ with respect to x is (xⁿ⁺¹)/(n+1) + C. For 2x, n = 1. Applying the rule, the integral is (2x²)/2 + C, which simplifies to x² + C. The constant C represents the constant of integration and accounts for the fact that there are infinitely many antiderivatives of a function, differing by a constant value. Thus, the result is x² + C, where C is arbitrary.Rate this question:
-
- 13.
What is the second derivative of 4x⁴ + 3x² with respect to x?
-
12x³ + 6x
-
12x³ + 6x + 3
-
16x³ + 6x
-
12x³ + 3x
Correct Answer
A. 12x³ + 6xExplanation
To find the second derivative of 4x⁴ + 3x², we first differentiate the function once. The derivative of 4x⁴ is 16x³, and the derivative of 3x² is 6x. Therefore, the first derivative is 16x³ + 6x. Differentiating this again gives the second derivative. The derivative of 16x³ is 48x², and the derivative of 6x is 6. Thus, the second derivative is 48x² + 6, which describes the rate of change of the rate of change of the function. This second derivative indicates how the curvature of the graph behaves at any point.Rate this question:
-
- 14.
What is the limit of sin(x)/x as x approaches 0?
-
0
-
1
-
Undefined
-
Infinity
Correct Answer
A. 1Explanation
The limit of sin(x)/x as x approaches 0 is a well-known result in calculus, and its value is 1. This can be shown using L'Hopital's Rule or by using the Taylor series expansion of sin(x). As x approaches 0, both the numerator and denominator approach 0, forming an indeterminate 0/0 form. By applying L'Hopital's Rule, we differentiate the numerator and denominator. The derivative of sin(x) is cos(x), and the derivative of x is 1. Thus, the limit becomes cos(0)/1, which equals 1.Rate this question:
-
- 15.
What is the derivative of cos(x) with respect to x?
-
-sin(x)
-
Sin(x)
-
Cos(x)
-
-cos(x)
Correct Answer
A. -sin(x)Explanation
The derivative of cos(x) with respect to x is -sin(x). This is a standard result from differentiation, derived from the fundamental trigonometric identities. The derivative of the cosine function describes the rate of change of the cosine curve at any point. When you differentiate cos(x), you are essentially calculating how fast the value of cos(x) is changing with respect to x. The result is -sin(x) because the graph of cos(x) slopes downward as x increases, which is why the derivative has a negative sign. This represents the rate of decrease of the cosine function.Rate this question:
-
Quiz Review Timeline (Updated): Dec 5, 2024 +
Our quizzes are rigorously reviewed, monitored and continuously updated by our expert board to maintain accuracy, relevance, and timeliness.
-
Current Version
-
Dec 05, 2024Quiz Edited by
ProProfs Editorial Team -
Jun 22, 2010Quiz Created by
Andrew Paul
Back to top