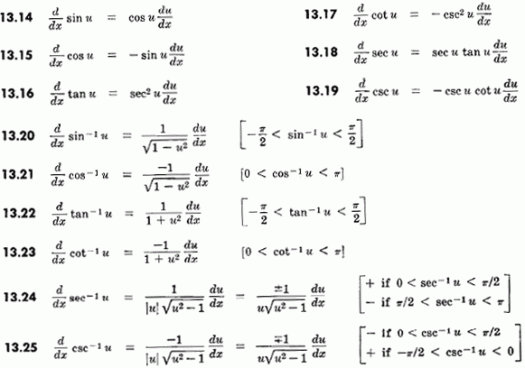
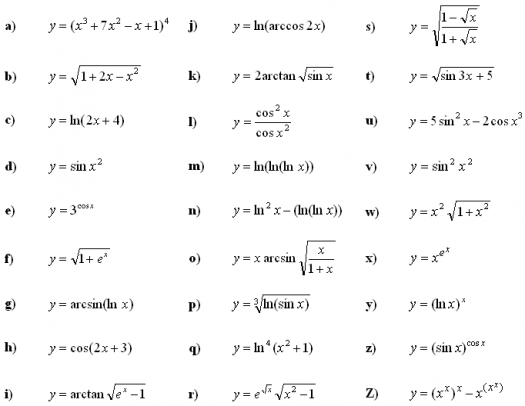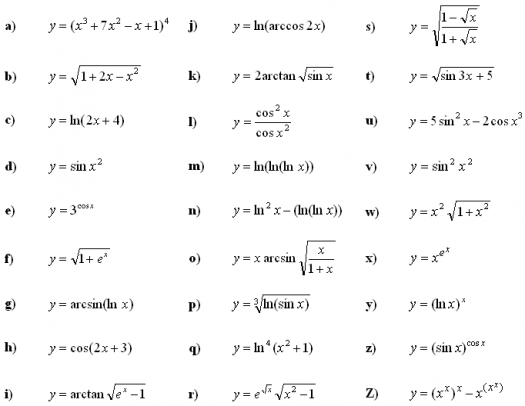Advanced Derivatives Assessment Test

2.

Find dy/dx if y = 52x-1.

52x-1 ln 10
52x-1 ln 5
52x-1 ln 25
52x-1 ln 2

Correct Answer

A. 52x-1 ln 25

Explanation

The given function is y = 52x-1. To find dy/dx, we need to take the derivative of y with respect to x. The derivative of 52x-1 is obtained by applying the power rule of differentiation, which states that if we have a function of the form ax^n, then its derivative is nax^(n-1). In this case, the derivative of 52x-1 is -52x^0 = -52. Therefore, the derivative of y with respect to x is -52. However, none of the given answer choices match -52. Therefore, the correct answer is not available.

Rate this question:

3.

Differentiate ax2 + c to the ½ power.

-2ax
2ax
2ax + c
Ax + 2c

Correct Answer

A. 2ax

Explanation

The correct answer is 2ax. When we differentiate ax^2 + c with respect to x, the power rule of differentiation tells us to multiply the coefficient of x^2 (which is 2a) by the exponent (which is 1) and subtract 1 from the exponent. This gives us 2ax. The constant term c does not affect the derivative, so it is not included in the answer.

Rate this question:

4.

Find the derivative of arcos 4x with respect to x.

-3/ [1 – (5x)^2]^2
-4 /0.5
[1 – (4x)^2]^0.5
-4 / [(4x)^2 – 1]^0.5

Correct Answer

A. -4 / [(4x)^2 – 1]^0.5

Explanation

The correct answer is -4 / [(4x)^2 – 1]^0.5.

To find the derivative of arccos(4x) with respect to x, we can use the chain rule. The derivative of arccos(u) with respect to u is -1 / sqrt(1 - u^2). In this case, u = 4x. So, the derivative of arccos(4x) with respect to x is -1 / sqrt(1 - (4x)^2). Simplifying this expression gives us -4 / [(4x)^2 - 1]^0.5, which matches the given answer.

Rate this question:

5.

Find the derivative of (y + 1)3 – y3 with respect to y.

6y – 3
3y – 3
6y + 3
3y + 6

Correct Answer

A. 6y + 3

Explanation

The given expression is a binomial expansion of (y + 1)^3 - y^3. To find the derivative, we can apply the power rule of differentiation. Taking the derivative of (y + 1)^3, we get 3(y + 1)^2. Taking the derivative of y^3, we get 3y^2. Subtracting these two derivatives, we get 3(y + 1)^2 - 3y^2. Simplifying this expression further, we get 3y^2 + 6y + 3 - 3y^2. The y^2 terms cancel out, leaving us with 6y + 3. Therefore, the correct answer is 6y + 3.

Rate this question:

6.

If a simple constant, what is the derivative of y = za?

A za-1
(a) - 2z
Xa
AX

Correct Answer

A. A za-1

Explanation

The derivative of y = za can be found using the power rule for differentiation. According to the power rule, when differentiating a term with a variable raised to a constant power, the derivative is equal to the constant multiplied by the variable raised to the power minus one. In this case, the constant is "a" and the variable is "z". Therefore, the derivative of y = za is aza-1.

Rate this question:

7.

What is the derivative of ln (cos y)?

Sec y
-sec y
–tan y
Tan y

Correct Answer

A. -sec y

Explanation

The derivative of ln (cos y) can be found using the chain rule. The derivative of ln(u) is 1/u times the derivative of u. In this case, u = cos y. The derivative of cos y is -sin y. Therefore, the derivative of ln (cos y) is -sin y / cos y, which can be simplified to -sec y.

Rate this question:

8.

What is the second derivative of y by implicit differentiation from the equation 4×2 + 8y2 = 36?

32xy
64×2
(– 9/4) y3
(- 16/9) y3

Correct Answer

A. (– 9/4) y3

Explanation

The second derivative of y can be found using implicit differentiation. To find the second derivative, we need to differentiate the equation twice with respect to x. The given equation is 4x^2 + 8y^2 = 36. Differentiating the equation once gives us 8x + 16yy' = 0. Differentiating again gives us 8 + 16y'(dy/dx) + 16y(dy/dx)' = 0. Since we are looking for the second derivative, we need to solve for (dy/dx)'. Rearranging the equation, we have (dy/dx)' = -8/(16y + 16yy'). Substituting y' = -4x/(8y), we get (dy/dx)' = -8/(16y - 16xy/2y) = -8/(16y - 8x) = -1/(2y - x). Simplifying further, we have (dy/dx)' = -1/(2y - x) = -9/(4y^3). Therefore, the second derivative is (-9/4)y^3.

Rate this question:

9.

What is the second derivative of this equation y3 – 5×2 + y = 0?

10y – 5
6y – 10
3y + 10
3×2 – 6y

Correct Answer

A. 6y – 10

Explanation

The second derivative of an equation can be found by taking the derivative of the first derivative. In this case, the first derivative of y^3 - 5*2 + y = 0 is 3y^2 - 10. Taking the derivative of this again gives us the second derivative, which is 6y. However, since the question asks for the second derivative of the equation y^3 - 5*2 + y = 0, the constant term -10 in the first derivative needs to be included in the second derivative as well. Therefore, the correct answer is 6y - 10.

Rate this question:

10.

What is the derivative of arccos 4y?

4 / (1 +16×2)0.5
4 / (1 – 16×2)0.5
-4 / (1 + 4×2)0.5
4 / (1 – 4×2)0.5

Correct Answer

A. 4 / (1 – 4×2)0.5

Explanation

The derivative of arccos 4y is found using the chain rule. The derivative of arccos u is -1 / (sqrt(1 - u^2)), where u = 4y in this case. Plugging in u = 4y into the derivative formula gives -1 / (sqrt(1 - (4y)^2)). Simplifying this expression gives -1 / (sqrt(1 - 16y^2)), which is equivalent to -1 / (sqrt(1 - 4x^2)). The given answer, 4 / (1 - 4x^2)^0.5, is the same as the derived expression but with a positive sign. Therefore, the given answer is correct.

Rate this question:

Advanced Derivatives Assessment Test

What is the derivative of (y + 5) / (y2 – 1) with respect to y?

Quiz Preview

Find dy/dx if y = 52x-1.

Differentiate ax2 + c to the ½ power.

Find the derivative of arcos 4x with respect to x.

Find the derivative of (y + 1)3 – y3 with respect to y.

If a simple constant, what is the derivative of y = za?

What is the derivative of ln (cos y)?

What is the second derivative of y by implicit differentiation from the equation 4×2 + 8y2 = 36?

What is the second derivative of this equation y3 – 5×2 + y = 0?

What is the derivative of arccos 4y?