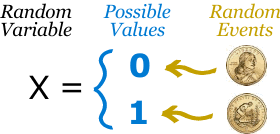Random Variables Assessment Test
(117).jpg)
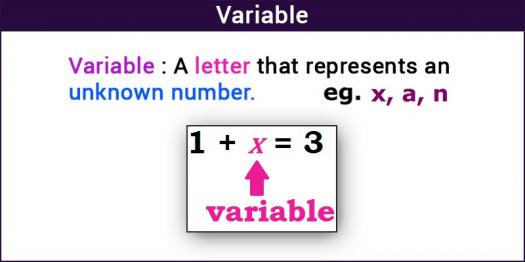
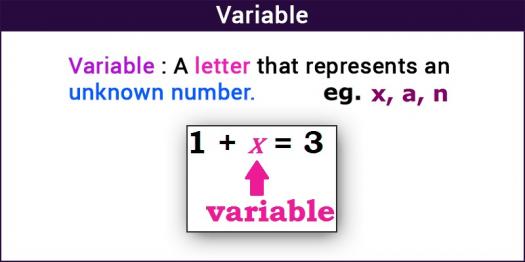
Random variables, as taught in statistics and probability, is a variable whose possible values are numerical outcomes of a random phenomenon. Take this quiz to find out more.
- 1.
A random variable can also be referred to as...
- A.
Clear
- B.
Open
- C.
Branched
- D.
Stochastic
Correct Answer
D. StochasticExplanation
A random variable can also be referred to as "stochastic" because it represents a variable whose outcome is determined by chance or probability. Stochastic variables are used in probability theory and statistics to model and analyze uncertain events or processes. They can take on different values with varying probabilities, and their behavior is often described using probability distributions.Rate this question:
-
- 2.
A variable whose possible values are numerical outcomes of a random phenomenon is termed...
- A.
Open variable
- B.
Clear variable
- C.
Random variable
- D.
Closed variable
Correct Answer
C. Random variableExplanation
A random variable is a term used to describe a variable that can take on different numerical outcomes as a result of a random or uncertain event or phenomenon. This means that the values of the variable are not predetermined or fixed, but rather depend on the outcome of the random process. Therefore, the correct answer is "Random variable."Rate this question:
-
- 3.
Random variables that can assume only a countable number of values are called...
- A.
Open
- B.
Closed
- C.
Discrete
- D.
Continuous
Correct Answer
C. DiscreteExplanation
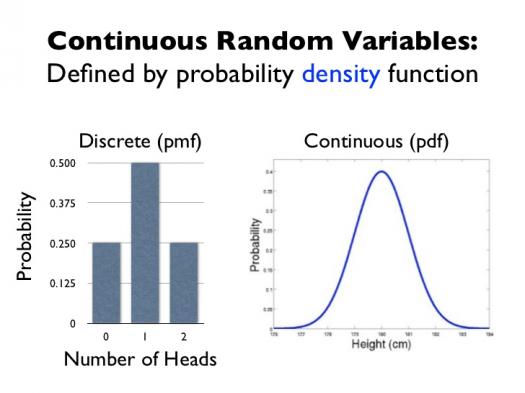
Random variables that can assume only a countable number of values are called discrete. Discrete random variables have specific, separate, and distinct values. They can be expressed as whole numbers or a finite set of values. Unlike continuous random variables, discrete random variables cannot take on any value within a given range.Rate this question:
-
- 4.
Random variables that can take on any of the countless number of values in an interval are called...
- A.
Continuous
- B.
Discrete
- C.
Closed
- D.
Open
Correct Answer
A. ContinuousExplanation
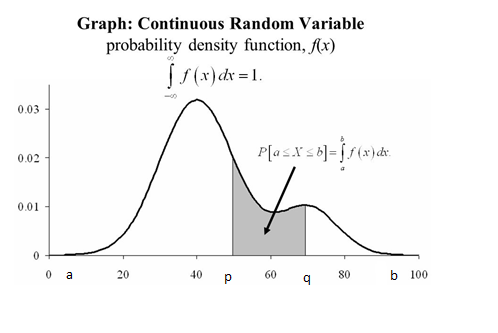
Random variables that can take on any of the countless number of values in an interval are called continuous. This means that the variable can take on any value within a range, including fractions and decimals. Unlike discrete variables, which can only take on specific, separate values, continuous variables have an infinite number of possible values. Closed and open are not appropriate terms to describe random variables, as they refer to intervals rather than the variables themselves.Rate this question:
-
- 5.
Variables which cannot take any value at all are...
- A.
Nonrandom
- B.
Ordinary
- C.
Calling
- D.
Ordinal
Correct Answer
A. NonrandomExplanation
Nonrandom variables are those that do not have any variation or variability in their values. In other words, these variables cannot take on different values and remain constant. They are fixed and unchanging. In contrast, random variables can take on different values based on chance or probability. Therefore, nonrandom variables cannot take any value at all, as they are not subject to variation or randomness.Rate this question:
-
- 6.
An example of a continuous variable is...
- A.
Distance
- B.
Acceleration
- C.
Force
- D.
Power
Correct Answer
A. DistanceExplanation
A continuous variable is one that can take on any value within a certain range. In this case, distance is a continuous variable because it can be measured in any unit of length and can have any value within that unit. Acceleration, force, and power, on the other hand, are not continuous variables as they have specific units and can only take on certain values within those units.Rate this question:
-
- 7.
Which of these restricts the measurement of a continuous variable?
- A.
Figure
- B.
Accuracy
- C.
Place
- D.
Number
Correct Answer
B. AccuracyExplanation
Accuracy restricts the measurement of a continuous variable because accuracy refers to the degree of closeness between a measured value and the true value of the variable. In the context of measurement, accuracy determines how precise and reliable the measurement is. Therefore, if the accuracy is low, it means that the measurement may have a larger margin of error, limiting the ability to accurately measure and quantify the continuous variable.Rate this question:
-
- 8.
If a variable can take on any value between two specified values, it is called...
- A.
Continuous variable
- B.
Discrete variable
- C.
Line variable
- D.
Real
Correct Answer
A. Continuous variableExplanation
A continuous variable is a variable that can take on any value within a specified range. Unlike discrete variables, which can only take on specific values, a continuous variable can take on any value within a range, including decimal values. In this case, the variable can take on any value between two specified values, indicating that it is a continuous variable.Rate this question:
-
- 9.
A random variable which takes values greater than or equal to zero with probability one is...
- A.
Nonnegative
- B.
Real
- C.
Trap
- D.
Slope
Correct Answer
A. NonnegativeExplanation
A random variable that takes values greater than or equal to zero with probability one is referred to as nonnegative. This means that the variable cannot have negative values and is restricted to zero or positive values only.Rate this question:
-
- 10.
Which is a type of random variables?
- A.
Continuous
- B.
Linear
- C.
Normal
- D.
Ordinal
Correct Answer
A. ContinuousExplanation
A continuous random variable is a type of random variable that can take on any value within a certain range or interval. It can have an infinite number of possible outcomes and is typically associated with measurements or quantities that can be expressed as real numbers. Examples of continuous random variables include height, weight, time, and temperature. In contrast, other options like linear, normal, and ordinal are not types of random variables but rather describe different characteristics or properties of random variables.Rate this question:
-
Quiz Review Timeline +
Our quizzes are rigorously reviewed, monitored and continuously updated by our expert board to maintain accuracy, relevance, and timeliness.
-
Current Version
-
Mar 20, 2023Quiz Edited by
ProProfs Editorial Team -
Jan 10, 2018Quiz Created by
Cripstwick
 Back to top
Back to top