9054 Any Solution From A & D

Reviewed by Editorial Team
The ProProfs editorial team is comprised of experienced subject matter experts. They've collectively created over 10,000 quizzes and lessons, serving over 100 million users. Our team includes in-house content moderators and subject matter experts, as well as a global network of rigorously trained contributors. All adhere to our comprehensive editorial guidelines, ensuring the delivery of high-quality content.
Learn about Our Editorial Process
| By Anthony Nunan
A
Anthony Nunan
Community Contributor
Quizzes Created: 132 | Total Attempts: 47,560
| Attempts: 98
SettingsSettings
Please wait...
  • 1/60 Questions
    When a = 30,    and d = 100 The rule for the Arithmetic Sequence is - ProProfs

    When a = 30,    and d = 100 The rule for the Arithmetic Sequence is

Please wait...
9054 Any Solution From A & D - Quiz
About This Quiz

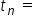
Using the first term (a) and the common difference (d), find the solution for the following problems.

Quiz Preview

  • 2. 
    When a = 30,    and d = 100 The rule for the Arithmetic Sequence is - ProProfs

    When a = 30,    and d = 100 The rule for the Arithmetic Sequence is

  • 3. 
    Above are the values for first term and common difference for an Arithmetic Sequence. What is the value of term 32 of this sequence? - ProProfs

    Above are the values for first term and common difference for an Arithmetic Sequence. What is the value of term 32 of this sequence?

    Explanation
    a is the first term, d is the common difference. Use the rule OR the App to calculate the term. Larger values are on page 1.3 in the table.

    Rate this question:

  • 4. 
    Above are the values for the first term and common difference for an Arithmetic Series. A series is the sum of a sequence. Also provided is a value for 'n', the total number of terms in the series. Find the value for the series. - ProProfs

    Above are the values for the first term and common difference for an Arithmetic Series. A series is the sum of a sequence. Also provided is a value for 'n', the total number of terms in the series. Find the value for the series.

    Explanation
    a is the first term, d is the common difference. Use the Series rule OR an App to calculate the term. Higher term values are on page 1.3 in the table. Make sure you use the 'series' column, not the 'sequence' column.

    Rate this question:

  • 5. 
    When a = 15 ,    and d = -4 The rule for the Arithmetic Sequence is - ProProfs

    When a = 15 ,    and d = -4 The rule for the Arithmetic Sequence is

  • 6. 
    When a =  1,    and d = 4 The rule for the Arithmetic Sequence is - ProProfs

    When a =  1,    and d = 4 The rule for the Arithmetic Sequence is

    Explanation
    The given answer, -3+4n, represents the formula for finding the nth term of an arithmetic sequence. In this formula, -3 is the initial term (a) and 4 is the common difference (d). By substituting the values of a=1 and d=4, we can find any term in the sequence by plugging in the value of n.

    Rate this question:

  • 7. 
    When a = 25,    and d = 6 The rule for the Arithmetic Sequence is - ProProfs

    When a = 25,    and d = 6 The rule for the Arithmetic Sequence is

  • 8. 
    When a = 38,    and d = 10 The rule for the Arithmetic Sequence is - ProProfs

    When a = 38,    and d = 10 The rule for the Arithmetic Sequence is

    Explanation
    The rule for the Arithmetic Sequence is given by the formula a + dn, where a is the first term, d is the common difference, and n is the term number. In this case, a = 28 and d = 10. So, the correct answer is 28 + 10n, which represents the nth term of the arithmetic sequence when a = 28 and d = 10.

    Rate this question:

  • 9. 
    When a = 6,    and d = 10 The rule for the Arithmetic Sequence is - ProProfs

    When a = 6,    and d = 10 The rule for the Arithmetic Sequence is

    Explanation
    The given answer, -4+10n, represents the formula for finding the nth term of an arithmetic sequence when the first term (a) is 6 and the common difference (d) is 10. In this case, the formula simplifies to -4+10n, where n represents the position of the term in the sequence.

    Rate this question:

  • 10. 
    When a = 7,    and d = 10 The rule for the Arithmetic Sequence is - ProProfs

    When a = 7,    and d = 10 The rule for the Arithmetic Sequence is

  • 11. 
    When a = 23,    and d = -100 The rule for the Arithmetic Sequence is - ProProfs

    When a = 23,    and d = -100 The rule for the Arithmetic Sequence is

  • 12. 
    When a = 8,    and d = -100 The rule for the Arithmetic Sequence is - ProProfs

    When a = 8,    and d = -100 The rule for the Arithmetic Sequence is

  • 13. 
    When a = 18,    and d = -9 The rule for the Arithmetic Sequence is - ProProfs

    When a = 18,    and d = -9 The rule for the Arithmetic Sequence is

    Explanation
    The given answer, 27-9n, is the rule for the arithmetic sequence when a = 18 and d = -9. In an arithmetic sequence, the first term is represented by 'a' and the common difference between each term is represented by 'd'. By substituting the given values into the rule, we get 27-9n as the expression to find the nth term of the sequence.

    Rate this question:

  • 14. 
    When a = 11,    and d = -3 The rule for the Arithmetic Sequence is - ProProfs

    When a = 11,    and d = -3 The rule for the Arithmetic Sequence is

  • 15. 
    When a = 35,    and d = -5 The rule for the Arithmetic Sequence is - ProProfs

    When a = 35,    and d = -5 The rule for the Arithmetic Sequence is

  • 16. 
    When a = -9,    and d = -2 The rule for the Arithmetic Sequence is - ProProfs

    When a = -9,    and d = -2 The rule for the Arithmetic Sequence is

  • 17. 
    When a = -2,    and d = -8 The rule for the Arithmetic Sequence is - ProProfs

    When a = -2,    and d = -8 The rule for the Arithmetic Sequence is

  • 18. 
    When a = -38,    and d = 200 The rule for the Arithmetic Sequence is - ProProfs

    When a = -38,    and d = 200 The rule for the Arithmetic Sequence is

  • 19. 
    When a = -24,    and d = -5 The rule for the Arithmetic Sequence is - ProProfs

    When a = -24,    and d = -5 The rule for the Arithmetic Sequence is

    Explanation
    The given expression represents the general term of an arithmetic sequence. In an arithmetic sequence, each term is obtained by adding a constant difference (d) to the previous term. In this case, the constant difference is -5. Therefore, the general term can be written as -19 - 5n, where n represents the position of the term in the sequence.

    Rate this question:

  • 20. 
    When a = -36,    and d = -10 The rule for the Arithmetic Sequence is - ProProfs

    When a = -36,    and d = -10 The rule for the Arithmetic Sequence is

  • 21. 
    When a = -19,    and d = -20 The rule for the Arithmetic Sequence is - ProProfs

    When a = -19,    and d = -20 The rule for the Arithmetic Sequence is

  • 22. 
    When a = -26,    and d = -6 The rule for the Arithmetic Sequence is - ProProfs

    When a = -26,    and d = -6 The rule for the Arithmetic Sequence is

  • 23. 
    When a = -14,    and d = 9 The rule for the Arithmetic Sequence is - ProProfs

    When a = -14,    and d = 9 The rule for the Arithmetic Sequence is

    Explanation
    The given answer, -23+9n, represents the formula for finding the nth term of an arithmetic sequence. In this case, the first term (a) is -14 and the common difference (d) is 9. By substituting these values into the formula, we get -23+9n. This means that each term in the sequence can be found by multiplying the position of the term (n) by 9 and then subtracting 23.

    Rate this question:

  • 24. 
    When a = -7,    and d = 7 The rule for the Arithmetic Sequence is - ProProfs

    When a = -7,    and d = 7 The rule for the Arithmetic Sequence is

    Explanation
    The given answer, -14+7n, represents the rule for the arithmetic sequence when a = -7 and d = 7. In an arithmetic sequence, each term is found by adding a constant difference, d, to the previous term. In this case, the first term is -7 and the common difference is 7. By substituting these values into the formula, we get -14+7n, where n represents the position of the term in the sequence. This formula allows us to find any term in the sequence by plugging in the corresponding value of n.

    Rate this question:

  • 25. 
    When a = -31,    and d = 9 The rule for the Arithmetic Sequence is - ProProfs

    When a = -31,    and d = 9 The rule for the Arithmetic Sequence is

    Explanation
    The given rule for the arithmetic sequence is -40+9n. This means that the nth term of the sequence can be found by multiplying the common difference, which is 9, by the position of the term in the sequence, n, and then subtracting 40. In this case, when a = -31, we can substitute this value into the rule to find the term. So, the term would be -40 + 9n = -40 + 9(-31) = -40 - 279 = -319.

    Rate this question:

  • 26. 
    When a = -11,    and d = 200 The rule for the Arithmetic Sequence is - ProProfs

    When a = -11,    and d = 200 The rule for the Arithmetic Sequence is

    Explanation
    The given expression represents the formula for finding the nth term of an arithmetic sequence. In this case, the first term (a) is -11 and the common difference (d) is 200. By substituting these values into the formula, we can find any term in the sequence by replacing the "n" with the desired term number. For example, to find the 5th term, we would replace "n" with 5 and simplify the expression to get -211+200(5) = -211+1000 = 789.

    Rate this question:

  • 27. 
    When a = -4,    and d = 7 The rule for the Arithmetic Sequence is - ProProfs

    When a = -4,    and d = 7 The rule for the Arithmetic Sequence is

    Explanation
    The given answer, -11+7n, represents the formula for finding the nth term of an arithmetic sequence. In this case, the first term (a) is -4 and the common difference (d) is 7. By substituting these values into the formula, we can determine any term in the sequence by replacing n with the desired term number. For example, if we want to find the 5th term, we would substitute n=5 into the formula: -11+7(5) = 24. Therefore, the 5th term of the sequence is 24.

    Rate this question:

  • 28. 
    When a = -29,    and d = 5 The rule for the Arithmetic Sequence is - ProProfs

    When a = -29,    and d = 5 The rule for the Arithmetic Sequence is

    Explanation
    The given rule for the arithmetic sequence is -34+5n, where n represents the position of the term in the sequence. This means that each term in the sequence can be found by substituting the corresponding value of n into the equation. In this case, when a = -29 and d = 5, we can find the term by substituting n = 1 into the equation: -34 + 5(1) = -29. Therefore, the correct answer is -29.

    Rate this question:

  • 29. 
    When a = -21,    and d = 2 The rule for the Arithmetic Sequence is - ProProfs

    When a = -21,    and d = 2 The rule for the Arithmetic Sequence is

    Explanation
    The given rule for the arithmetic sequence is -23+2n. This means that to find any term in the sequence, we need to substitute the value of n (the position of the term) into the equation. In this case, since a = -21, we can substitute -21 into the equation and solve for n. Thus, the correct answer is -23+2n.

    Rate this question:

  • 30. 
    When a = -14,    and d = 9 The rule for the Arithmetic Sequence is - ProProfs

    When a = -14,    and d = 9 The rule for the Arithmetic Sequence is

    Explanation
    The given arithmetic sequence is represented by the formula -23 + 9n, where n represents the position of each term in the sequence. By substituting the values of a = -14 and d = 9 into the formula, we can find the correct answer.

    Rate this question:

  • 31. 
    When a = -36,    and d = 20 The rule for the Arithmetic Sequence is - ProProfs

    When a = -36,    and d = 20 The rule for the Arithmetic Sequence is

    Explanation
    The given answer, -56+20n, represents the rule for the arithmetic sequence when a = -36 and d = 20. In an arithmetic sequence, each term is found by adding a constant difference, d, to the previous term. In this case, the first term is -36 and the common difference is 20. By substituting these values into the formula, we get -56+20n, where n represents the position of a term in the sequence. This formula will give us the correct term for any position in the sequence.

    Rate this question:

  • 32. 
    When a = -16,    and d = 20 The rule for the Arithmetic Sequence is - ProProfs

    When a = -16,    and d = 20 The rule for the Arithmetic Sequence is

    Explanation
    The given rule for the arithmetic sequence is -36+20n, where n represents the position of the term in the sequence. This means that each term in the sequence can be found by substituting the value of n into the equation. In this case, when a = -16 and d = 20, we can substitute these values into the equation to find the answer. Therefore, the answer is -36+20n.

    Rate this question:

  • 33. 
    When a = -26,    and d = 20 The rule for the Arithmetic Sequence is - ProProfs

    When a = -26,    and d = 20 The rule for the Arithmetic Sequence is

    Explanation
    The given answer, -46+20n, represents the formula for finding the nth term of an arithmetic sequence. In this case, the first term of the sequence is -46 and the common difference between each term is 20. By substituting the value of n, the position of the term in the sequence, we can calculate the corresponding term.

    Rate this question:

  • 34. 
    When a = 3,    and d = 200 The rule for the Arithmetic Sequence is - ProProfs

    When a = 3,    and d = 200 The rule for the Arithmetic Sequence is

    Explanation
    The given answer is an expression that represents the nth term of an arithmetic sequence. In this case, the common difference (d) is 200 and the first term (a) is 3. The expression -197+200n represents the formula for finding the nth term of the sequence. By substituting the values of a and d into the formula, we can find any term in the sequence by plugging in the value of n.

    Rate this question:

  • 35. 
    When a = 31,    and d = 200 The rule for the Arithmetic Sequence is - ProProfs

    When a = 31,    and d = 200 The rule for the Arithmetic Sequence is

    Explanation
    The given answer, -169+200n, represents the formula for finding the nth term of an arithmetic sequence when the first term (a) is 31 and the common difference (d) is 200. In this formula, -169 represents the difference between the first term and the constant term, and 200n represents the product of the common difference and the position of the term in the sequence. By substituting different values of n into the formula, we can find the corresponding terms of the arithmetic sequence.

    Rate this question:

  • 36. 
    When a = 26,    and d = 5 The rule for the Arithmetic Sequence is - ProProfs

    When a = 26,    and d = 5 The rule for the Arithmetic Sequence is

    Explanation
    The given answer, 21+5n, represents the rule for the arithmetic sequence when a = 26 and d = 5. In an arithmetic sequence, each term is found by adding a constant difference, d, to the previous term. In this case, the first term, a, is 26, and the common difference, d, is 5. Therefore, the rule for finding the nth term in this arithmetic sequence is 21+5n, where n represents the position of the term in the sequence.

    Rate this question:

  • 37. 
    Above are the values for first term and common difference for an Arithmetic Sequence. What is the value of term 34 of this sequence? - ProProfs

    Above are the values for first term and common difference for an Arithmetic Sequence. What is the value of term 34 of this sequence?

    Explanation
    a is the first term, d is the common difference. Use the rule OR the App to calculate the term. Larger values are on page 1.3 in the table.

    Rate this question:

  • 38. 
    Above are the values for first term and common difference for an Arithmetic Sequence. What is the value of term 35 of this sequence? - ProProfs

    Above are the values for first term and common difference for an Arithmetic Sequence. What is the value of term 35 of this sequence?

    Explanation
    a is the first term, d is the common difference. Use the rule OR the App to calculate the term. Larger values are on page 1.3 in the table.

    Rate this question:

  • 39. 
    Above are the values for first term and common difference for an Arithmetic Sequence. What is the value of term 40 of this sequence? - ProProfs

    Above are the values for first term and common difference for an Arithmetic Sequence. What is the value of term 40 of this sequence?

    Explanation
    a is the first term, d is the common difference. Use the rule OR the App to calculate the term. Larger values are on page 1.3 in the table.

    Rate this question:

  • 40. 
    Above are the values for first term and common difference for an Arithmetic Sequence. What is the value of term 31 of this sequence? - ProProfs

    Above are the values for first term and common difference for an Arithmetic Sequence. What is the value of term 31 of this sequence?

    Explanation
    a is the first term, d is the common difference. Use the rule OR the App to calculate the term. Larger values are on page 1.3 in the table.

    Rate this question:

  • 41. 
    Above are the values for first term and common difference for an Arithmetic Sequence. What is the value of term 34 of this sequence? - ProProfs

    Above are the values for first term and common difference for an Arithmetic Sequence. What is the value of term 34 of this sequence?

    Explanation
    a is the first term, d is the common difference. Use the rule OR the App to calculate the term. Larger values are on page 1.3 in the table.

    Rate this question:

  • 42. 
    Above are the values for first term and common difference for an Arithmetic Sequence. What is the value of term 22 of this sequence? - ProProfs

    Above are the values for first term and common difference for an Arithmetic Sequence. What is the value of term 22 of this sequence?

    Explanation
    a is the first term, d is the common difference. Use the rule OR the App to calculate the term. Larger values are on page 1.3 in the table.

    Rate this question:

  • 43. 
    Above are the values for first term and common difference for an Arithmetic Sequence. What is the value of term 23 of this sequence? - ProProfs

    Above are the values for first term and common difference for an Arithmetic Sequence. What is the value of term 23 of this sequence?

    Explanation
    a is the first term, d is the common difference. Use the rule OR the App to calculate the term. Larger values are on page 1.3 in the table.

    Rate this question:

  • 44. 
    Above are the values for first term and common difference for an Arithmetic Sequence. What is the value of term 21 of this sequence? - ProProfs

    Above are the values for first term and common difference for an Arithmetic Sequence. What is the value of term 21 of this sequence?

    Explanation
    a is the first term, d is the common difference. Use the rule OR the App to calculate the term. Larger values are on page 1.3 in the table.

    Rate this question:

  • 45. 
    Above are the values for first term and common difference for an Arithmetic Sequence. What is the value of term 38 of this sequence? - ProProfs

    Above are the values for first term and common difference for an Arithmetic Sequence. What is the value of term 38 of this sequence?

    Explanation
    a is the first term, d is the common difference. Use the rule OR the App to calculate the term. Larger values are on page 1.3 in the table.

    Rate this question:

  • 46. 
    Above are the values for first term and common difference for an Arithmetic Sequence. What is the value of term 24 of this sequence? - ProProfs

    Above are the values for first term and common difference for an Arithmetic Sequence. What is the value of term 24 of this sequence?

    Explanation
    a is the first term, d is the common difference. Use the rule OR the App to calculate the term. Larger values are on page 1.3 in the table.

    Rate this question:

  • 47. 
    Above are the values for the first term and common difference for an Arithmetic Series. A series is the sum of a sequence. Also provided is a value for 'n', the total number of terms in the series. Find the value for the series. - ProProfs

    Above are the values for the first term and common difference for an Arithmetic Series. A series is the sum of a sequence. Also provided is a value for 'n', the total number of terms in the series. Find the value for the series.

    Explanation
    a is the first term, d is the common difference. Use the Series rule OR an App to calculate the term. Higher term values are on page 1.3 in the table. Make sure you use the 'series' column, not the 'sequence' column.

    Rate this question:

  • 48. 
    Above are the values for the first term and common difference for an Arithmetic Series. A series is the sum of a sequence. Also provided is a value for 'n', the total number of terms in the series. Find the value for the series. - ProProfs

    Above are the values for the first term and common difference for an Arithmetic Series. A series is the sum of a sequence. Also provided is a value for 'n', the total number of terms in the series. Find the value for the series.

    Explanation
    a is the first term, d is the common difference. Use the Series rule OR an App to calculate the term. Higher term values are on page 1.3 in the table. Make sure you use the 'series' column, not the 'sequence' column.

    Rate this question:

  • 49. 
    Above are the values for the first term and common difference for an Arithmetic Series. A series is the sum of a sequence. Also provided is a value for 'n', the total number of terms in the series. Find the value for the series. - ProProfs

    Above are the values for the first term and common difference for an Arithmetic Series. A series is the sum of a sequence. Also provided is a value for 'n', the total number of terms in the series. Find the value for the series.

    Explanation
    a is the first term, d is the common difference. Use the Series rule OR an App to calculate the term. Higher term values are on page 1.3 in the table. Make sure you use the 'series' column, not the 'sequence' column.

    Rate this question:

Quiz Review Timeline (Updated): Mar 21, 2023 +

Our quizzes are rigorously reviewed, monitored and continuously updated by our expert board to maintain accuracy, relevance, and timeliness.

  • Current Version
  • Mar 21, 2023
    Quiz Edited by
    ProProfs Editorial Team
  • May 11, 2015
    Quiz Created by
    Anthony Nunan
Back to Top Back to top
Advertisement
×

Wait!
Here's an interesting quiz for you.

We have other quizzes matching your interest.