Perimeter And Area
(150).jpg "Perimeter And Area - Quiz")
Math Unit 8
Questions and Answers
- 1.
Understanding area and perimeter can help me to know what it will cost me to put a new floor in my home or put a fence around my yard.
- A.
True
- B.
False
Correct Answer
A. TrueExplanation
Understanding area and perimeter is important because it allows me to calculate the amount of material I will need for a project such as putting a new floor in my home or installing a fence around my yard. By knowing the area, I can determine the amount of flooring or fencing material required, which in turn helps me estimate the cost of the project accurately. Therefore, the statement is true.Rate this question:
-
- 2.
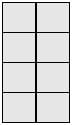
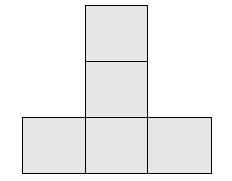
What is the area of the figure?
- A.
4
- B.
8
- C.
2
Correct Answer
B. 8Explanation
The area of the figure is 8 because the figure is a rectangle with a length of 4 units and a width of 2 units. To find the area of a rectangle, you multiply the length by the width, which in this case is 4 x 2 = 8.Rate this question:
-
- 3.
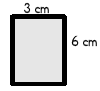
Which number sentence represents the area of the figure?
- A.
3 + 6 =
- B.
3 x 6 =
- C.
3 + 6 + 3 + 6 =
Correct Answer
B. 3 x 6 =Explanation
The number sentence "3 x 6 =" represents the area of the figure because it is a multiplication equation, and the area of a figure is calculated by multiplying the length by the width. In this case, the length is 3 and the width is 6, so multiplying them together gives us the area of the figure.Rate this question:
-
- 4.
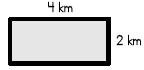
Which number sentence represents the area of the figure?
- A.
2 + 4 =
- B.
4 + 4 =
- C.
4 x 2 =
Correct Answer
C. 4 x 2 =Explanation
The number sentence 4 x 2 = represents the area of the figure.Rate this question:
-
- 5.
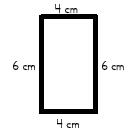
Which number sentence represents the perimeter of the figure?
- A.
4+6+4+6 =
- B.
4 + 6 =
- C.
6 x 4 =
Correct Answer
A. 4+6+4+6 =Explanation
The given number sentence, 4+6+4+6, represents the perimeter of the figure.Rate this question:
-
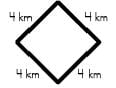
- 6.
For which figure would the perimeter be solved by the number sentence 4+6+4+6?a. b. c.
- A.
Figure a
- B.
Figure b
- C.
Figure c
Correct Answer
C. Figure cExplanation
Figure c would have a perimeter that is solved by the number sentence 4+6+4+6 because it has sides of length 4 and 6. The perimeter of a figure is the sum of the lengths of all its sides, so adding up the lengths of the sides in figure c gives us a perimeter of 20.Rate this question:
-
- 7.
A rectangle has a length of 8 meters and a width of 5 meters. What is its perimeter and area?
- A.
Perimeter: 26 meters, Area: 40 square meters
- B.
Perimeter: 26 meters, Area: 30 square meters
- C.
Perimeter: 40 meters, Area: 26 square meters
- D.
Perimeter: 30 meters, Area: 40 square meters
Correct Answer
A. Perimeter: 26 meters, Area: 40 square metersExplanation
To find the perimeter and area of a rectangle with a length of 8 meters and a width of 5 meters:
The perimeter is calculated by adding the length and the width, then multiplying by 2. So, (8 + 5) times 2 equals 26 meters.
The area is calculated by multiplying the length by the width. So, 8 times 5 equals 40 square meters.
Therefore, the correct answer is: Perimeter: 26 meters, Area: 40 square metersRate this question:
-
- 8.
Which figure has an area of 5? a. b. c.
- A.
A
- B.
B
- C.
C
Correct Answer
A. A -
- 9.
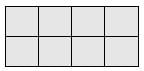
Considering a single cube is a unit, what will be the perimeter of a rectangle formed by eight such cubes?
- A.
12
- B.
4
- C.
4 x 2
Correct Answer
A. 12Explanation
If a single cube has a unit area (which means each face of the cube has a side length of 1 unit), and you arrange 8 of these cubes to form a rectangular shape, it would be a 2x2x2 cube.
To find the perimeter of this rectangular shape, you can add up the lengths of all the edges. In this case, the perimeter would be:
Perimeter = 2 * (length + width + height)
Perimeter = 2 * (2 + 2 + 2)
Perimeter = 2 * 6
Perimeter = 12 units
So, the perimeter of the rectangle formed by 8 such cubes would be 12 units.Rate this question:
-
- 10.
A rectangle has a perimeter of 26 cm and an area of 40 cm². What is the difference between the length and width of the rectangle?
- A.
2 cm
- B.
3 cm
- C.
5 cm
- D.
6 cm
Correct Answer
B. 3 cmExplanation
Let the length of the rectangle be 'l' and the width be 'w'. We are given:
Perimeter: 2(l + w) = 26 cm, which simplifies to l + w = 13 cm
Area: l * w = 40 cm²
We need to find the difference between the length and width, i.e., |l - w|.
To solve this, we can use the fact that (l + w)² - 4lw = (l - w)².
Substituting the given values: 13² - 4 * 40 = (l - w)² This simplifies to 169 - 160 = (l - w)² Therefore, 9 = (l - w)² Taking the square root of both sides, we get |l - w| = 3 cm.Rate this question:
-
Quiz Review Timeline +
Our quizzes are rigorously reviewed, monitored and continuously updated by our expert board to maintain accuracy, relevance, and timeliness.
-
Current Version
-
Oct 15, 2024Quiz Edited by
ProProfs Editorial Team -
Apr 21, 2014Quiz Created by
Susan Johnson
- Data Handling Quizzes
- Decimal Quizzes
- Discrete Mathematics Quizzes
- Equation Quizzes
- Fraction Quizzes
- Function Quizzes
- Geometry Quizzes
- Graph Quizzes
- Measurement Quizzes
- Multiplication Quizzes
- Number Quizzes
- Percentage Quizzes
- Place Value Quizzes
- Probability Quizzes
- Problem Solving Quizzes
- Ratio Quizzes
- Statistics Quizzes
- Trigonometry Quizzes
Back to top