Five Number Summary And Box And Whisker Plot

Reviewed by Editorial Team
The ProProfs editorial team is comprised of experienced subject matter experts. They've collectively created over 10,000 quizzes and lessons, serving over 100 million users. Our team includes in-house content moderators and subject matter experts, as well as a global network of rigorously trained contributors. All adhere to our comprehensive editorial guidelines, ensuring the delivery of high-quality content.
Learn about Our Editorial Process
| By Mahaffeymath
M
Mahaffeymath
Community Contributor
Quizzes Created: 1 | Total Attempts: 1,631
| Attempts: 1,631
SettingsSettings
Please wait...
  • 1/10 Questions
    Based on the box and whisker plot below, what is the median of the data? - ProProfs

    Based on the box and whisker plot below, what is the median of the data?

    • 30
    • 45
    • 20
Please wait...
About This Quiz

When you feel you are ready, take the following checkpoint quiz. You must get 80% before moving on to lesson 3.
Be sure the enter your name in the box below.

Five Number Summary And Box And Whisker Plot - Quiz

Quiz Preview

  • 2. 
    Based on the box and whisker plot below, what is the range of the data? - ProProfs

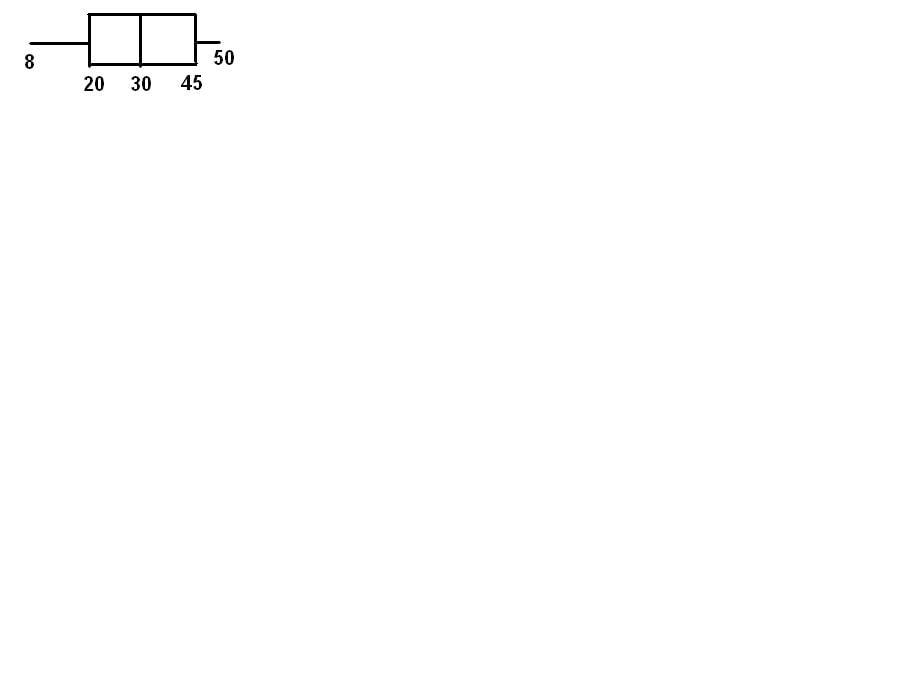
    Based on the box and whisker plot below, what is the range of the data?

    • 42

    • 25

    • 30

    Correct Answer
    A. 42
    Explanation
    The range of the data can be determined by subtracting the smallest value from the largest value. In this case, the largest value on the box and whisker plot is 42 and the smallest value is not visible on the plot. Therefore, the range of the data is 42.

    Rate this question:

  • 3. 
    Based on the box and whisker plot below, what is the IQR of the data? - ProProfs

    Based on the box and whisker plot below, what is the IQR of the data?

    • 42

    • 25

    • 30

    Correct Answer
    A. 25
    Explanation
    The IQR (Interquartile Range) is a measure of statistical dispersion, specifically the range between the first quartile (Q1) and the third quartile (Q3) of a dataset. In this case, since the answer is 25, it means that the range between Q1 and Q3 is 25 units.

    Rate this question:

  • 4. 

    Determine the 5 number summary for the set of data:5, 20, 15, 25, 0, 10, 15, 5, 25, 30, 20

    • 5, 15, 10, 25, 20

    • 0, 7.5, 17.5, 22.5, 30

    • 0, 5, 15, 25, 30

    Correct Answer
    A. 0, 5, 15, 25, 30
    Explanation
    The 5 number summary consists of the minimum value, the first quartile (Q1), the median (Q2), the third quartile (Q3), and the maximum value of the data set. In this case, the minimum value is 0, the first quartile is 5, the median is 15, the third quartile is 25, and the maximum value is 30. Therefore, the correct answer is 0, 5, 15, 25, 30.

    Rate this question:

  • 5. 

    What percent of the data listed below is higher than the median?88, 53, 72, 67, 48, 63, 25, 34, 46, 55, 72, 77

    • 25%

    • 50%

    • 75%

    Correct Answer
    A. 50%
    Explanation
    The median is the middle value of a set of data when arranged in ascending order. In this case, the data listed in ascending order is 25, 34, 46, 48, 53, 55, 63, 67, 72, 72, 77, 88. The median is the average of the two middle values, which are 55 and 63. Therefore, there are 6 values (72, 72, 77, 88) that are higher than the median, out of a total of 12 values. So, the percentage of data higher than the median is 6/12 or 50%.

    Rate this question:

  • 6. 

    Determine the Interquartile range for the following set of data:5, 20, 15, 25, 0, 10, 15, 5, 25, 30, 20

    • 20

    • 25

    • 15

    Correct Answer
    A. 20
    Explanation
    The interquartile range is a measure of the spread of the middle 50% of the data. To find it, we first need to arrange the data in ascending order: 0, 5, 5, 10, 15, 15, 20, 20, 25, 25, 30. The lower quartile (Q1) is the median of the lower half of the data, which is 10. The upper quartile (Q3) is the median of the upper half of the data, which is 25. The interquartile range is then calculated by subtracting Q1 from Q3: 25 - 10 = 15. Therefore, the correct answer is 15.

    Rate this question:

  • 7. 

    What is the 5 number summary of the following data?100, 150, 50, 30, 90, 50

    • 30, 50, 50, 100, 150

    • 30, 50, 90, 100, 150

    • 30, 50, 75, 100, 150

    Correct Answer
    A. 30, 50, 75, 100, 150
    Explanation
    The 5 number summary provides a summary of the distribution of the data. It consists of the minimum value, the first quartile (Q1), the median (Q2), the third quartile (Q3), and the maximum value. In this case, the minimum value is 30, the first quartile is 50, the median is 75, the third quartile is 100, and the maximum value is 150.

    Rate this question:

  • 8. 

    What percent of the data listed below is higher than the upper quartile?88, 53, 72, 67, 48, 63, 25, 34, 46, 55, 72, 77

    • 25%

    • 50%

    • 75%

    Correct Answer
    A. 25%
    Explanation
    The upper quartile is the median of the upper half of the data. To find the upper quartile, we first need to arrange the data in ascending order: 25, 34, 46, 48, 53, 55, 63, 67, 72, 72, 77, 88. The upper half of the data is 63, 67, 72, 72, 77, 88. Since there are 6 numbers in the upper half and only 1 number (88) is higher than the upper quartile, the percentage of data higher than the upper quartile is 1/6 or approximately 16.7%. Therefore, the correct answer is 25%, which is the closest option to 16.7%.

    Rate this question:

  • 9. 

    What percent of the data listed below is higher than the lower quartile?88, 53, 72, 67, 48, 63, 25, 34, 46, 55, 72, 77

    • 25%

    • 50%

    • 75%

    Correct Answer
    A. 75%
    Explanation
    The lower quartile is the median of the lower half of the data. In this case, the lower quartile is 48. Out of the 12 data points listed, there are 9 data points (72, 67, 63, 55, 72, 77) that are higher than 48. Therefore, the percentage of the data that is higher than the lower quartile is 9/12 = 75%.

    Rate this question:

  • 10. 

    What is the interquartile range of the following data? 100, 150, 50, 30, 90, 50

    • 50

    • 75

    • 100

    Correct Answer
    A. 50
    Explanation
    To find the interquartile range (IQR) of the given data, follow these steps:
    Order the data: 30, 50, 50, 90, 100, 150
    Find the median (Q2):
    There are 6 data points, so the median is the average of the 3rd and 4th values:
    Median = (50 + 90) / 2 = 70
    Find the lower quartile (Q1):
    Q1 is the median of the first half of the data (30, 50, 50):
    Q1 = 50 (middle value of the first three numbers)
    Find the upper quartile (Q3):
    Q3 is the median of the second half of the data (90, 100, 150):
    Q3 = 100 (middle value of the last three numbers)
    Calculate the IQR:
    IQR = Q3 - Q1
    IQR = 100 - 50 = 50

    Rate this question:

Quiz Review Timeline (Updated): May 23, 2024 +

Our quizzes are rigorously reviewed, monitored and continuously updated by our expert board to maintain accuracy, relevance, and timeliness.

  • Current Version
  • May 23, 2024
    Quiz Edited by
    ProProfs Editorial Team
  • Dec 30, 2010
    Quiz Created by
    Mahaffeymath
Back to Top Back to top
Advertisement
×

Wait!
Here's an interesting quiz for you.

We have other quizzes matching your interest.