Math Honors: Right Triangle Test

Are you ready to test your geometry skills? Take our Honors Right Triangle Test and challenge yourself with advanced questions on right triangles! This quiz is designed for high-achieving students, math enthusiasts, and anyone looking to deepen their understanding of right triangle properties and theorems.
Each question is carefully crafted to challenge your critical thinking and problem-solving abilities. Whether you're studying for an upcoming test, brushing up on your geometry skills, or simply love math, this quiz offers a fun and engaging way to learn.
By taking the Honors Right Triangle Test, you'll gain valuable insights into your strengths and Read moreareas for improvement. Share your results with classmates and friends to see how you compare and to foster a collaborative learning experience. Perfect for honors students and anyone passionate about geometry, this quiz is a great way to enhance your understanding and achieve academic success. Take the quiz now and see how well you know right triangles!
Right Triangle Questions and Answers
- 1.
What is the area of a triangle with base 15 and height 5?
Explanation
The area of a triangle can be calculated using the formula: Area = (base * height) / 2. In this case, the base is given as 15 and the height is given as 5. Plugging these values into the formula, we get (15 * 5) / 2 = 75 / 2 = 37.5. Therefore, the area of the triangle is 37.5.Rate this question:
- 2.
What is the area of a triangle with base 12 and height 4?
Explanation
The area of a triangle is calculated by multiplying the base by the height and dividing the result by 2. In this case, the base is 12 and the height is 4. Therefore, the area of the triangle is (12 * 4) / 2 = 24.Rate this question:
- 3.
What is the area of the triangle below?
Explanation
The area of a triangle is given by: 1/2 x Base x Height
Given that the base is 14 units and the height is 5 units, we can substitute these values into the formula:
=1/2 x 14 x 5
=1/2 x 70
=35Rate this question:
- 4.
What is the area of the triangle below?
Explanation
The area of a triangle is given by: 1/2 x Base x Height
Given that the base is 10 units and the height is 11 units, we can substitute these values into the formula:
=1/2 x 10 x 11
=1/2 x 110
=55Rate this question:
- 5.
A right triangle has two legs of lengths 4, and 8. What is the length of the hypotenuse?
- A.
8.94
- B.
9
- C.
12
- D.
6.92
- E.
3.46
Correct Answer
A. 8.94Explanation
The length of the hypotenuse of a right triangle can be found using the Pythagorean theorem, which states that the square of the hypotenuse is equal to the sum of the squares of the other two sides. In this case, the squares of the legs are 4^2 = 16 and 8^2 = 64. Adding these values together gives 16 + 64 = 80. Taking the square root of 80 gives approximately 8.94, which is the length of the hypotenuse.Rate this question:
-
- 6.
A right triangle has a hypotenuse of length 13 and one leg of length 10. What is the length of the other leg?
- A.
8
- B.
16.40
- C.
8.31
- D.
23
- E.
3
Correct Answer
C. 8.31Explanation
Using the Pythagorean theorem, we can find the length of the other leg of the right triangle. The theorem states that the square of the hypotenuse is equal to the sum of the squares of the other two sides. In this case, we have the hypotenuse as 13 and one leg as 10. By substituting these values into the equation and solving for the missing leg, we find that the length of the other leg is approximately 8.31.Rate this question:
-
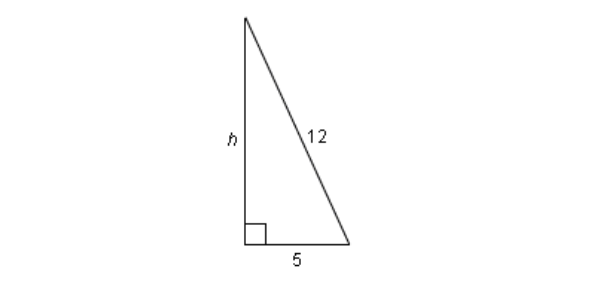
- 7.
What is the length of the missing side in the right triangle below?
- A.
101.12
- B.
11.18
- C.
25
- D.
5
- E.
5.43
Correct Answer
B. 11.18Explanation
To find the length of the missing side in the right triangle, we can use the Pythagorean theorem. The Pythagorean theorem states that in a right triangle, the square of the length of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the lengths of the other two sides.
The formula is:
c^2 = a^2 + b^2
where ccc is the hypotenuse, and aaa and bbb are the other two sides.
Given:
The length of side AB (one of the legs) = 10 units
The length of side BC (the hypotenuse) = 15 units
We need to find the length of side AC (the other leg).
Plugging the known values into the Pythagorean theorem:
15^2 = 10^2 + AC^2
225 = 100 + AC^2
Subtracting 100 from both sides to solve for AC^2:
225−100=AC^2
125 = AC^2
Taking the square root of both sides to find ACACAC:
AC=√125
AC=5√5
So, the length of the missing side AC 5√5 units, is approximately 11.18 units.Rate this question:
-
- 8.
What is the length of the missing side in the right triangle below?
- A.
4.99
- B.
9
- C.
12
- D.
8.60
- E.
12.87
Correct Answer
D. 8.60Explanation
To find the length of the missing side in this right triangle, we will again use the Pythagorean theorem:
c^2=a^2+b^2
Given:
The length of side AB = 7 units (one of the legs)
The length of side AC = 5 units (the other leg)
We need to find the length of side BC (the hypotenuse).
Plugging the known values into the Pythagorean theorem:
BC^2=AB^2+AC^2
BC^2 = 7^2 + 5^2
BC^2 = 49 + 25
BC^2 = 74
BC=√74
So, the length of the missing BC is √74 units, which is approximately 8.60 units.Rate this question:
-
- 9.
What is the area of a right triangle with one leg 4 and the other 9?
Correct Answer
18Explanation
To find the area of a right triangle, you can use the formula:
Area = 1/2 x base x height
Given that the base is 9 units and the height is 4 units, we can substitute these values into the formula:
Area= 1/2 x 9 x 4
=1/2x36
=18Rate this question:
- 10.
Ken leans a 12-foot ladder against his house. He places the ladder so that the base is 5 feet from the house. How far up the house does the ladder reach?
Correct Answer
10.91Explanation
To determine how far up the house the ladder reaches, we can use the Pythagorean theorem. The ladder forms a right triangle with the house, where the ladder is the hypotenuse (12 feet), the distance from the base of the ladder to the house is one leg (5 feet), and the height the ladder reaches up the house is the other leg (let's call it h)
The Pythagorean theorem states:
c^2=a^2+b^2
where
c is the hypotenuse, and
𝑎
a and b are the legs of the right triangle.
Given:
c=12 feet
a=5 feet
b=h (the height we need to find)
Plugging in the values:
12^2=5^2+b^2
144=25+b^2
b^2=144-25
b^2=119
b=√119
h≈10.91
So, the ladder reaches approximately 10.91 feet up the house.Rate this question:
- 11.
What right triangle congruence theorem proves that the two triangles are congruent?
- A.
HL
- B.
LL
- C.
SAS
- D.
HA
- E.
HAL
Correct Answer
A. HLExplanation
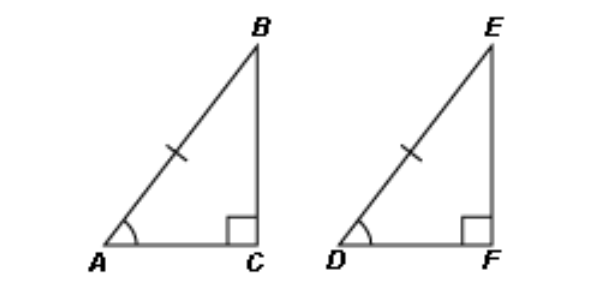
The HL (Hypotenuse-Leg) congruence theorem states that if the hypotenuse and one leg of a right triangle are congruent to the hypotenuse and one leg of another right triangle, then the two triangles are congruent. In other words, if the lengths of the hypotenuse and one leg of two right triangles are equal, then the triangles are congruent.Rate this question:
-
- 12.
What right triangle congruence theorem proves that the two triangles are congruent?
- A.
LA
- B.
LL
- C.
ASA
- D.
HA
- E.
AA
Correct Answer
D. HAExplanation
The correct answer, HA, refers to the Hypotenuse-Angle congruence theorem. This theorem states that if the hypotenuse and one acute angle of a right triangle are congruent to the hypotenuse and one acute angle of another right triangle, then the two triangles are congruent. In this case, the given triangles must have congruent hypotenuses and one congruent acute angle in order to be proven congruent using the HA theorem.Rate this question:
-
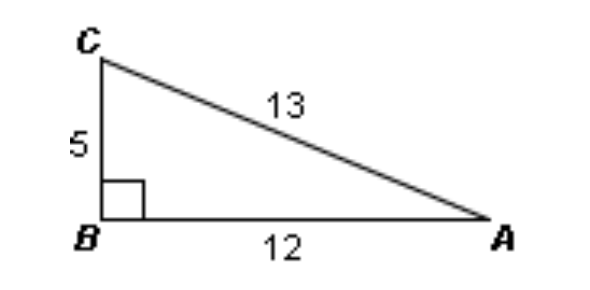
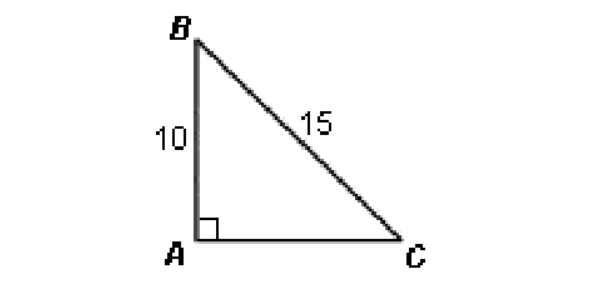
- 13.
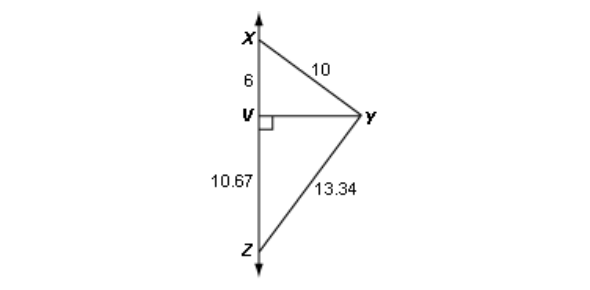
What is the distance from point Y to XZ?
Correct Answer
8Explanation
Given:
VX = 6 units (one leg of the right triangle)
XY = 10 units (the hypotenuse)
We need to find VY, the other leg of the right triangle.
Using the Pythagorean theorem:
XY^2=VX^2+VY^2
10^2=6^2+VY^2
100=36+VY^2
VY^2=100-36
VY^2=64
VY=√64
VY=8Rate this question:
- 14.
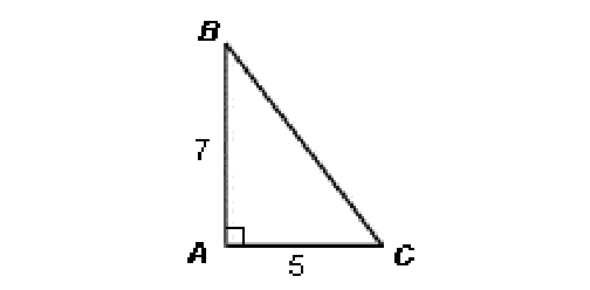
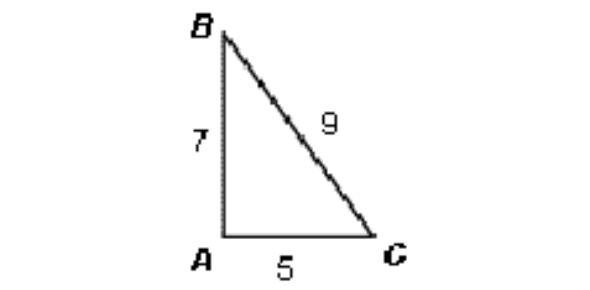
True or False: The triangle below is a right triangle.
- A.
True
- B.
False
Correct Answer
B. FalseExplanation
To determine if the given triangle is a right triangle, we need to check if the Pythagorean theorem holds true for the sides of the triangle. The Pythagorean theorem states that in a right triangle, the square of the length of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the lengths of the other two sides.
Given:
AB=7 units (one leg)
AC=5 units (the other leg)
BC=9 units (the hypotenuse)
We need to check if:
BC^2=AB^2+AC^2
Calculating each term:
9^2=7^2+5^2
81=49+25
81≠74
Since BC^2≠AB^2+AC^2 (81 ≠ 74), the Pythagorean theorem does not hold true for this triangle.
Therefore, the given triangle is not a right triangle.
Hence the answer is False.Rate this question:
-
- 15.
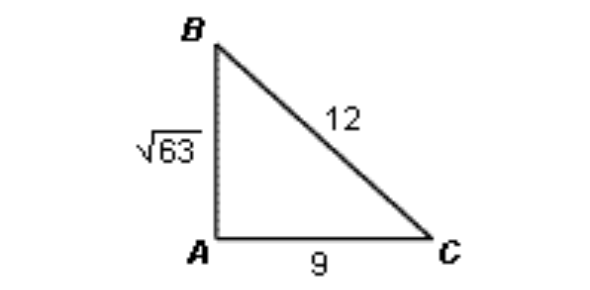
True or False: The triangle below is a right triangle.
- A.
True
- B.
False
Correct Answer
A. TrueExplanation
To determine if the given triangle is a right triangle, we can use the Pythagorean theorem. According to the Pythagorean theorem, in a right triangle, the square of the length of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the lengths of the other two sides.
Given:
AB=√63 (one leg)
AC=9 (the other leg)
BC=12 (the hypotenuse)
We need to check if:
BC^2=AB^2+AC^2
12^2=(√63)^2+9^2
144=63+81
144=144
Since BC^2=AB^2+AC^2, the Pythagorean theorem holds true for this triangle.
Therefore, the given triangle is a right triangle.Rate this question:
-
- 16.
What is s? (Hint: use the definition for the 30-6-90 right triangle)
- A.
12
- B.
24
- C.
20.78
- D.
12√3
- E.
12√2
Correct Answer
D. 12√3Explanation
In a 30°-60°-90° right triangle, the side lengths have a specific ratio. The sides opposite the 30°, 60°, and 90° angles are in the ratio 1 : √3 : 2, respectively.
Given that the side opposite the 60° angle is 12 units, we can find the other sides using this ratio.
Let's denote:
sss as the side opposite the 30° angle.
The hypotenuse as the side opposite the 90° angle.
According to the ratio, if the side opposite the 60° angle is 12, then:
The oppositeRate this question:
-
- 17.
What is s? (Hint: use the definition for the 30-60-90 right triangle)
- A.
8
- B.
8√2
- C.
4√3
- D.
8√3
- E.
Option 6
Correct Answer
A. 8 -
- 18.
What is s? (Hint: Use the definition for a 45-45-90 special right triangle)
- A.
12
- B.
6
- C.
12√2
- D.
24
- E.
12√3
Correct Answer
C. 12√2Explanation
The side "s" in a 45-45-90 special right triangle is equal to the length of one of the legs multiplied by the square root of 2. In this case, the length of one of the legs is 12, so "s" would be equal to 12 multiplied by the square root of 2, which is 12√2.Rate this question:
-
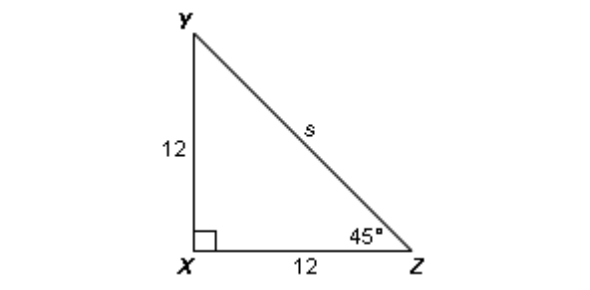
- 19.
What is s? (Hint: Use the definition for a 45-45-90 special right triangle)
- A.
8
- B.
4
- C.
8√3
- D.
8√2
- E.
4√2
Correct Answer
A. 8Explanation
The length of side s in a 45-45-90 special right triangle is equal to the length of the other two sides, which are both 8 units long. Therefore, the correct answer is 8.Rate this question:
-
- 20.
What is the sin 30?
Correct Answer
7/14, 1/2Explanation
To find the value of sin30∘, we can use the properties of a 30°-60°-90° triangle.
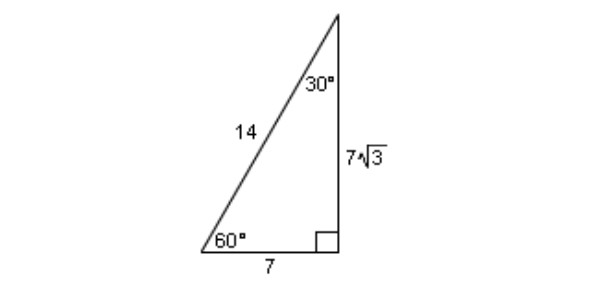
In a 30°-60°-90° triangle:
The side opposite the 30° angle is half the length of the hypotenuse.
The side opposite the 60° angle is √3 times the length of the side opposite the 30° angle.
The hypotenuse is twice the length of the side opposite the 30° angle.
Given the triangle in the image:
The hypotenuse is 14 units.
The side opposite the 30° angle is 7 units.
The side opposite the 60° angle is 7√3 units.
The sine of an angle in a right triangle is defined as the length of the opposite side divided by the length of the hypotenuse. Therefore:
Sin 30∘ = Opposite/Hypotenuse
For this triangle:
Sin 30∘ = 7/14=1/2Rate this question:
- 21.
What is sec 60?
Correct Answer
14/7 , 2Explanation
To find the value of sec 60∘, we can use the properties of a 30°-60°-90° triangle.
In a 30°-60°-90° triangle:
The side opposite the 30° angle is half the length of the hypotenuse.
The side opposite the 60° angle is √3 times the length of the side opposite the 30° angle.
The hypotenuse is twice the length of the side opposite the 30° angle.
Given the triangle in the image:
The hypotenuse is 14 units.
The side opposite the 30° angle is 7 units.
The side opposite the 60° angle is 7√3
The secant of an angle is the reciprocal of the cosine of that angle. Therefore:
sec 60∘ = 1/Cos 60∘
We know that,
Cos 60∘ = Adjacent/Hypotenuse
For this triangle:
The side adjacent to the 60° angle is 7 units (opposite to 30°).
The hypotenuse is 14 units.
So,
Cos 60∘ = 7/14 = 1/2
Therefore,
sec 60∘ = 1/1/2
sec 60∘=2Rate this question:
- 22.
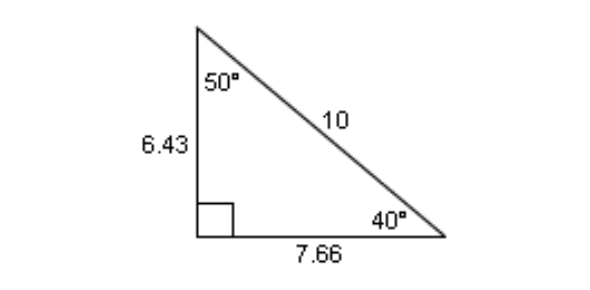
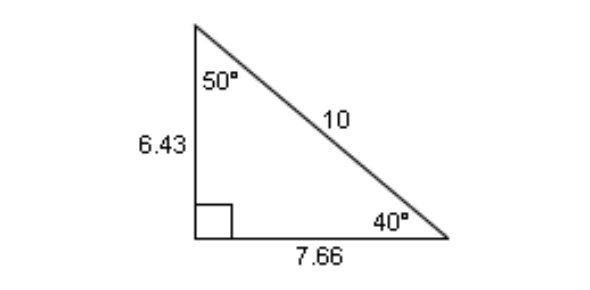
What is the tan 50?
Correct Answer
7.66/6.43, 0.839Explanation
The correct answer is 7.66/6.43. The question asks for the value of the tangent of 50 degrees. The tangent of an angle is the ratio of the length of the side opposite the angle to the length of the side adjacent to the angle in a right triangle. Since the question does not specify a unit, we can assume it is referring to radians. Using a calculator, we can find that the approximate value of the tangent of 50 degrees is 1.1918. However, the given answer is in the form of a fraction, which is not equivalent to the approximate value. Therefore, the given answer is incorrect.Rate this question:
- 23.
What is the cos 40?
Correct Answer
7.66/10, 0.766 - 24.
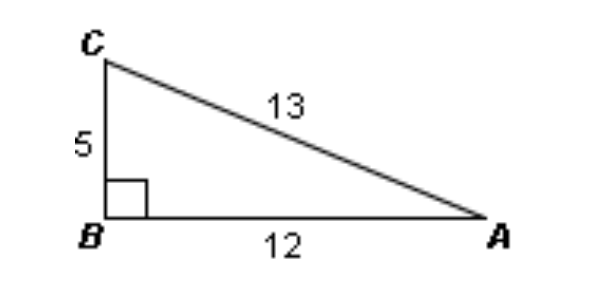
What is cot C?
Correct Answer
5/12Explanation
The value of cot C is 5/12.Rate this question:
- 25.
What is csc A?
Correct Answer
13/5 - 26.
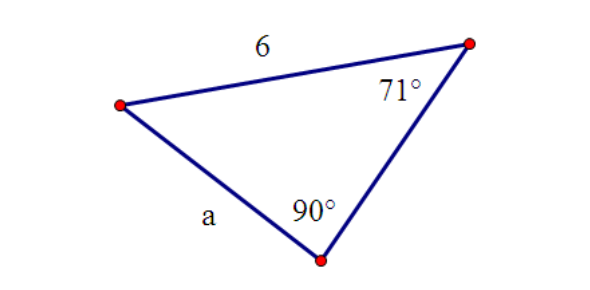
What is a?
- A.
6
- B.
5.67
- C.
7
- D.
1.95
- E.
17.45
Correct Answer
B. 5.67Explanation
To find the value of aaa when the angle opposite to side aaa is 71°, we can use the sine function for a right triangle.
Given:
Hypotenuse = 6 units
Angle opposite to side aaa = 71°
We use the sine function because we know the opposite angle (71°) and the hypotenuse. The sine of an angle in a right triangle is defined as the ratio of the opposite side to the hypotenuse:
Sin (θ) = Opposite / Hypotenuse
Here, θ=71∘, opposite side = a, and hypotenuse = 6 units. So,
Sin (71∘) = a/6
To solve for a:
a=6⋅sin(71∘)
Using a calculator to find sin(71∘):
sin(71∘)≈0.9455
Now, multiply by 6:
a≈6 x 0.9455
So, the value of a is approximately 5.67 units (rounded to two decimal places).
4oRate this question:
-
- 27.
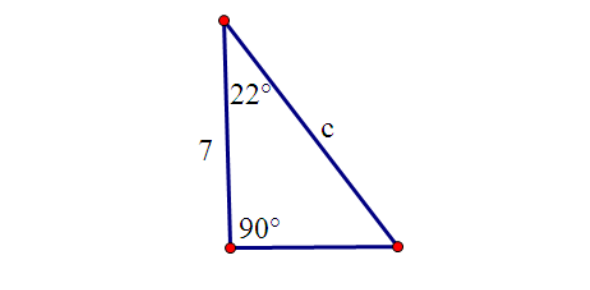
What is c?
- A.
2.62
- B.
8
- C.
7.5
- D.
7
- E.
6.51
Correct Answer
C. 7.5Explanation
Given:
Adjacent side = 7 units
Angle = 22°
We use the cosine function because we know the adjacent side and the angle. The cosine of an angle in a right triangle is defined as the ratio of the adjacent side to the hypotenuse:
cos(θ)= adjacent / hypotenuse
Here, θ=22∘, the adjacent side = 7 units, and the hypotenuse = c. So,
Cos(22∘)=7/c
c=7/Cos(22∘)
Using a calculator to find cos(22∘):
cos(22∘)≈0.9272
Therefore,
c=7/0.9272
c=7.5 (Rounded to one decimal place)Rate this question:
-
- 28.
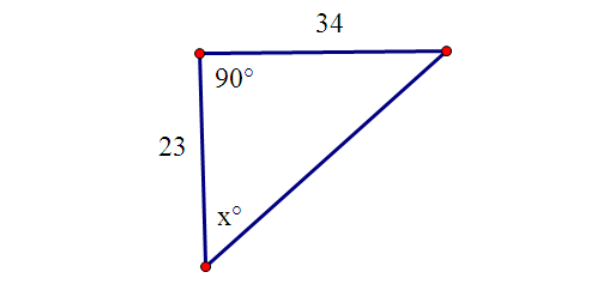
What is x, in degrees?
- A.
42.57
- B.
34.08
- C.
0.56
- D.
55.92
- E.
0.42
Correct Answer
D. 55.92Explanation
You are right. My apologies for the confusion. Let's correctly identify the sides relative to angle xxx:
Opposite side to x = 34 units
Adjacent side to x = 23 units
We should use the tangent function to find angle x:
The tangent of an angle in a right triangle is defined as the ratio of the length of the opposite side to the length of the adjacent side:
tan(x) = opposite/adjacent
Given:
Opposite side = 34 units
Adjacent side = 23 units
tan(x)= 23/34
To find the angle x, we take the inverse tangent (arctan) of the ratio:
x=tan−1(34/23)
Using a calculator to find the inverse tangent:
x≈tan−1(1.4783)
x≈56.31∘
So, the value of angle xxx is approximately 56.31 degrees.Rate this question:
-
- 29.
True or false? Two right triangles are similar if the acute angles of one triangle are congruent to the acute angles of the other triangle.
- A.
True
- B.
False
Correct Answer
A. TrueExplanation
Two right triangles are similar if the acute angles of one triangle are congruent to the acute angles of the other triangle. This is because in similar triangles, the corresponding angles are congruent. In right triangles, the acute angles are complementary, meaning that the sum of their measures is 90 degrees. Therefore, if the acute angles of two right triangles are congruent, it implies that the third angles are also congruent, resulting in similar triangles.Rate this question:
-
- 30.
Which of the following are Pythagorean triples? Check all that apply.
- A.
7, 12, 14.1
- B.
25, 60, 72.4
- C.
25, 60, 65
- D.
5, 12, 13
- E.
8, 16, 17
- F.
16, 30, 34
Correct Answer(s)
C. 25, 60, 65
D. 5, 12, 13
F. 16, 30, 34 -
- 31.
Which of the following are not congruence theorems for right triangles? Check all that apply.
- A.
HL
- B.
HA
- C.
HH
- D.
LA
- E.
LL
- F.
AA
Correct Answer(s)
C. HH
F. AAExplanation
The two congruence theorems for right triangles are HL (hypotenuse-leg) and HA (hypotenuse-angle). HH (hypotenuse-hypotenuse) and AA (angle-angle) are not congruence theorems for right triangles.Rate this question:
-
- 32.
A 45-45-90 triangle has a hypotenuse of 12√2, what is the length of one of it's legs?
Correct Answer(s)
12Explanation
In a 45-45-90 triangle, the two legs are congruent, meaning they have the same length. The hypotenuse is equal to the length of one leg multiplied by the square root of 2. In this case, the hypotenuse is given as 12√2, so the length of one leg can be found by dividing the hypotenuse by √2. Simplifying this, we get 12 as the length of one of the legs.Rate this question:
- 33.
In a 30-60-90 triangle the hypotenuse is 42, what is the length of the side opposite from the 30 degree angle?
Correct Answer(s)
21Explanation
In a 30-60-90 triangle, the side opposite the 30 degree angle is half the length of the hypotenuse. Therefore, if the hypotenuse is 42, the length of the side opposite the 30 degree angle would be half of that, which is 21.Rate this question:
- 34.
On the square baseball diamond, the distance from first base to second base is 90 feet. What is the distance from 2nd base to home plate? (Round your answer to two decimal places)
Correct Answer(s)
63.64Explanation
A baseball diamond is a square, and the distance from one base to the next is the side length of the square. The distance from first base to second base forms the hypotenuse of a right triangle where the legs are the sides of the square.
Given:
The distance from first base to second base (the hypotenuse) is 90 feet.
We need to find the distance from second base to home plate, which is the same as the distance from first base to third base. This distance is also the diagonal of the square, and it can be found using the Pythagorean theorem in a 45°-45°-90° triangle.
For a 45°-45°-90° triangle, if the legs are aaa and the hypotenuse is c:Rate this question:
- 35.
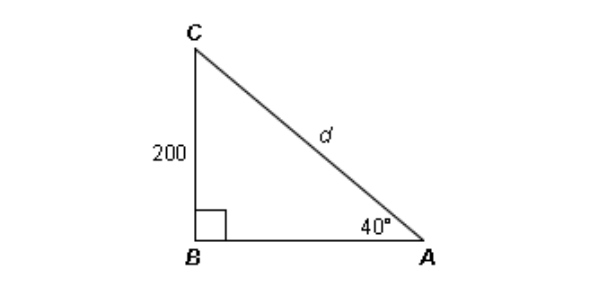
A ski resort is building a new ski lift that will transport tourists from the base of the mountain to its highest point. This mountain has a vertical height of 200 yards, and the ski lift will rise at an angle of 40 degrees. When the project is completed, how many yards, d, will a tourist travel from the base of the mountain to its peak? (Round your answer to two decimal places)
Correct Answer(s)
311.14Explanation
To find the distance d that a tourist will travel from the base to the peak of the mountain, we need to use trigonometric functions in the given right triangle.
Given:
Vertical height BC=200 yards (opposite side)
Angle ∠CAB=40∘
Hypotenuse d (the distance the tourist will travel)
We can use the sine function, which relates the opposite side and the hypotenuse:Rate this question:
Quiz Review Timeline +
Our quizzes are rigorously reviewed, monitored and continuously updated by our expert board to maintain accuracy, relevance, and timeliness.
-
Current Version
-
Jun 05, 2024Quiz Edited by
ProProfs Editorial Team -
Jan 12, 2011Quiz Created by
Smatook
 Back to top
Back to top






.webp)
.webp)
.webp)