Linear Functions-points And Slopes
Please complete the following questions
Questions and Answers
- 1.
Calculate the slope of the line that goes through A(-4, 5) and B(2, -7)
- A.
2
- B.
-1/2
- C.
1/2
- D.
-2
- E.
None of the above
Correct Answer
D. -2Explanation
The slope of a line can be calculated using the formula (change in y)/(change in x). In this case, the change in y is -7 - 5 = -12 and the change in x is 2 - (-4) = 6. Therefore, the slope is -12/6 = -2.Rate this question:
-
- 2.
Calculate the slope of the line that goes through (2, -1) and (0, -2)
- A.
2
- B.
-1/2
- C.
1/2
- D.
-2
- E.
None of the Above
Correct Answer
C. 1/2Explanation
To calculate the slope of a line, we use the formula: slope = (change in y) / (change in x). In this case, the change in y is -1 - (-2) = 1, and the change in x is 2 - 0 = 2. So, the slope is 1/2.Rate this question:
-
- 3.
Identify the slope in the equation C = - 200 t + 540
- A.
C
- B.
200
- C.
T
- D.
540
- E.
None of the above
Correct Answer
E. None of the above -
- 4.
If the first differences are _______________ then the relationship is linear.
Correct Answer
constantExplanation
If the first differences are constant, it means that the difference between consecutive terms in the sequence is always the same. This indicates a linear relationship, where each term can be obtained by adding a fixed value to the previous term. In a linear relationship, the rate of change between any two terms remains constant throughout the sequence.Rate this question:
- 5.
Rate of change can also be referred to as the _______________ of a line.
Correct Answer
slopeExplanation
The rate of change refers to how much a quantity changes in relation to another quantity. In the context of a line, the rate of change is commonly referred to as the slope. The slope represents the steepness or inclination of the line and indicates how much the dependent variable changes for a given change in the independent variable. Therefore, the correct answer for this question is slope.Rate this question:
- 6.
Calculate the rise length for the line going through A(3, 2) and B(-5, 0)
- A.
8
- B.
-8
- C.
-2
- D.
2
- E.
None of the above
Correct Answer
D. 2Explanation
The rise length is the vertical distance between two points on a line. To calculate it, we subtract the y-coordinate of point A from the y-coordinate of point B. In this case, the y-coordinate of A is 2 and the y-coordinate of B is 0. Therefore, the rise length is 2.Rate this question:
-
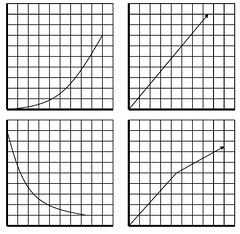
- 7.
Which graph is linear?
- A.
Top-Left
- B.
Top-Right
- C.
Bottom-Left
- D.
Bottom-Right
Correct Answer
B. Top-RightExplanation
The graph that is linear is the one in the top-right position.Rate this question:
-
- 8.
Determine the rate of change for the relation shown in the graph.
- A.
2
- B.
1/2
- C.
-1/2
- D.
-2
Correct Answer
C. -1/2Explanation
The rate of change for the relation shown in the graph is -1/2. This means that for every unit increase in the x-coordinate, the y-coordinate decreases by 1/2. In other words, as x increases, y decreases at a constant rate of 1/2.Rate this question:
-
- 9.
Determine the rate of change for the relation shown in the table of values.
- A.
-2
- B.
-1/2
- C.
1/2
- D.
2
Correct Answer
B. -1/2Explanation
The rate of change for the relation shown in the table of values is -1/2. This means that for every unit increase in the input variable, the output variable decreases by 1/2.Rate this question:
-
- 10.
Determine the slope of the line that passes through (-9,7) and (2, -6)
- A.
-13/11
- B.
-11/13
- C.
11/13
- D.
13/11
Correct Answer
A. -13/11Explanation
To determine the slope of a line passing through two points, we use the formula: slope = (change in y)/(change in x). In this case, the change in y is -6 - 7 = -13, and the change in x is 2 - (-9) = 11. Therefore, the slope is -13/11.Rate this question:
-
- 11.
Determine the slope of the line that passes through (15,-8) and (10, 3)
- A.
11/5
- B.
1
- C.
-1
- D.
-11/5
Correct Answer
D. -11/5Explanation
To determine the slope of a line passing through two points, we use the formula: slope = (change in y)/(change in x). In this case, the change in y is -8 - 3 = -11, and the change in x is 15 - 10 = 5. Therefore, the slope is -11/5.Rate this question:
-
- 12.
What is the slope from the bottom right of the hill to the top of the hill.
- A.
2.5
- B.
2/5
- C.
-2/5
- D.
-2.5
Correct Answer
D. -2.5Explanation
The slope from the bottom right of the hill to the top of the hill is -2.5. This means that for every unit of horizontal distance, there is a decrease of 2.5 units in vertical distance. In other words, the hill has a steep descent from the bottom right to the top.Rate this question:
-
- 13.
Which ordered pairs are also in the relation where the rise is -2, the run is 3, and (6,2) lies on the line.
- A.
(-3, 4) and (3,8)
- B.
(-9, -12) and (-6, 2)
- C.
(0, 9) and (-2, 12)
- D.
(9, 0) and (12, -2)
Correct Answer
D. (9, 0) and (12, -2)Explanation
The ordered pairs (9, 0) and (12, -2) are also in the relation because they satisfy the given conditions. The rise is -2, which means that the y-coordinate decreases by 2 units. The run is 3, which means that the x-coordinate increases by 3 units. Starting from the point (6, 2), if we decrease the y-coordinate by 2 units, we get (6, 0). If we increase the x-coordinate by 3 units, we get (9, 0). Similarly, starting from (6, 2), if we decrease the y-coordinate by 2 units, we get (6, 0). If we increase the x-coordinate by 3 units, we get (12, -2). Therefore, (9, 0) and (12, -2) are in the relation.Rate this question:
-
- 14.
Determine which ordered pairs are also in the relation where the rise is 3, the run is 2, and (-4,10) lies on the line.
- A.
(-8, -4) and (0, 16)
- B.
(4, 22) and (-2,13)
- C.
(-4, -10) and (-16, 8)
- D.
(8, 22) and (-8, 4)
Correct Answer
B. (4, 22) and (-2,13)Explanation
The given question states that the rise is 3 and the run is 2. This means that for every increase of 2 units in the x-coordinate, the y-coordinate increases by 3 units. We are also given that (-4, 10) lies on the line.
For the ordered pair (4, 22), the change in x-coordinate is 4 - (-4) = 8 units, and the change in y-coordinate is 22 - 10 = 12 units. The ratio of the change in y-coordinate to the change in x-coordinate is 12/8 = 3/2, which matches the given rise and run. Therefore, (4, 22) is in the relation.
For the ordered pair (-2, 13), the change in x-coordinate is -2 - (-4) = 2 units, and the change in y-coordinate is 13 - 10 = 3 units. The ratio of the change in y-coordinate to the change in x-coordinate is 3/2, which matches the given rise and run. Therefore, (-2, 13) is also in the relation.
Hence, the correct answer is (4, 22) and (-2, 13).Rate this question:
-
- 15.
What is the slope of the relation y = -4/5x - 4
- A.
4
- B.
-4
- C.
4/5
- D.
-4/5
Correct Answer
D. -4/5Explanation
The slope of a linear relation is the coefficient of the x-term. In this case, the given equation is in the form y = mx + b, where m is the slope. Therefore, the slope of the relation y = -4/5x - 4 is -4/5.Rate this question:
-
Quiz Review Timeline +
Our quizzes are rigorously reviewed, monitored and continuously updated by our expert board to maintain accuracy, relevance, and timeliness.
-
Current Version
-
Aug 21, 2023Quiz Edited by
ProProfs Editorial Team -
Mar 03, 2013Quiz Created by
Melanie10100
- Arithmetic Quizzes
- Calculus Quizzes
- Data Handling Quizzes
- Decimal Quizzes
- Discrete Mathematics Quizzes
- Fraction Quizzes
- Function Quizzes
- Graph Quizzes
- Measurement Quizzes
- Multiplication Quizzes
- Number Quizzes
- Percentage Quizzes
- Place Value Quizzes
- Probability Quizzes
- Problem Solving Quizzes
- Ratio Quizzes
- Shape Quizzes
- Statistics Quizzes
- Trigonometry Quizzes
Back to top