Polygons And Quadrilaterals Test-period 7
This is your test on Polygons and Quadrilaterals. �You are to complete it in class. �You may only take it once.
- 1.
How many sides does a nonagon have?
- A.
9
- B.
More than 10
- C.
0
- D.
7
Correct Answer
A. 9Explanation
A nonagon is a polygon with nine sides. Therefore, the correct answer is 9.Rate this question:
-
- 2.
How many sides total does a heptagon and a decagon have together?
- A.
17
- B.
16
- C.
15
- D.
18
Correct Answer
A. 17Explanation
A heptagon has 7 sides and a decagon has 10 sides. To find the total number of sides, we add the number of sides of both polygons together. Therefore, a heptagon and a decagon together have 7 + 10 = 17 sides.Rate this question:
-
- 3.
What is the sum of the exterior angles of a 34-gon?
- A.
180
- B.
360
- C.
90
- D.
5760
Correct Answer
B. 360Explanation
The sum of the exterior angles of any polygon, regardless of the number of sides, is always 360 degrees. This is a property of polygons, where the exterior angles of a polygon add up to a complete revolution, or 360 degrees. Therefore, the sum of the exterior angles of a 34-gon would also be 360 degrees.Rate this question:
-
- 4.
What is the sum of the interior angles of a hexagon?
- A.
360
- B.
180
- C.
720
- D.
1080
Correct Answer
C. 720Explanation
A hexagon has six sides, and the sum of the interior angles of any polygon can be found using the formula (n-2) * 180, where n is the number of sides. In this case, (6-2) * 180 = 4 * 180 = 720. Therefore, the sum of the interior angles of a hexagon is 720.Rate this question:
-
- 5.
What's the measure of each interior angle of a regular pentagon?
- A.
540°
- B.
90°
- C.
72°
- D.
108°
Correct Answer
D. 108°Explanation
A regular pentagon has five equal sides and five equal angles. To find the measure of each interior angle, we can use the formula (n-2) * 180° / n, where n is the number of sides of the polygon. Substituting n = 5, we get (5-2) * 180° / 5 = 3 * 180° / 5 = 540° / 5 = 108°. Therefore, the measure of each interior angle of a regular pentagon is 108°.Rate this question:
-
- 6.
What is the measure of each exterior angle of a regular heptagon?
- A.
51.43°
- B.
360°
- C.
154.23°
- D.
1080°
Correct Answer
A. 51.43°Explanation
The measure of each exterior angle of a regular heptagon can be found by dividing the total sum of the exterior angles (360°) by the number of sides (7). Therefore, each exterior angle of a regular heptagon is 51.43°.Rate this question:
-
- 7.
How many sides does a regular polygon have if each exterior angle is 22.5°?
- A.
15
- B.
12
- C.
17
- D.
16
Correct Answer
D. 16Explanation
A regular polygon has equal angles and equal sides. The sum of the exterior angles of any polygon is always 360 degrees. Therefore, if each exterior angle is 22.5 degrees, we can divide 360 by 22.5 to find the number of sides. 360/22.5 = 16. So, a regular polygon with each exterior angle measuring 22.5 degrees has 16 sides.Rate this question:
-
- 8.
What is x in the rectangle below?
- A.
22°
- B.
158°
- C.
136°
- D.
68°
Correct Answer
C. 136°Explanation
The angle measures in a rectangle add up to 360 degrees. Since the other three angles are given as 22°, 158°, and 68°, the missing angle can be found by subtracting the sum of these three angles from 360°. Subtracting 22° + 158° + 68° from 360° gives us 112°. Therefore, the missing angle is 136°.Rate this question:
-
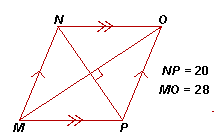
- 9.
What is x in the parallelogram below?
- A.
90
- B.
6
- C.
22
- D.
12
Correct Answer
B. 6Explanation
In a parallelogram, opposite angles are equal. Since the given angle measures are 90, 6, 22, and 12, the opposite angles must also be equal. Therefore, x must be equal to the angle measure of 6.Rate this question:
-
- 10.
What is x in the parallelogram below?
- A.
22
- B.
64
- C.
11
- D.
3
Correct Answer
A. 22 -
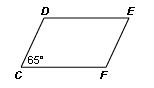
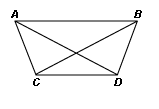
- 11.
What's the measure of angle E?
- A.
115°
- B.
25°
- C.
65°
- D.
90°
Correct Answer
C. 65°Explanation
The measure of angle E is 65° because it is the only option that is not a right angle (90°) and is also not a small angle like 25°. Additionally, it is not a large angle like 115°. Therefore, the only remaining option is 65°.Rate this question:
-
- 12.
What is the measure of angle D?
- A.
65°
- B.
115°
- C.
90°
- D.
25°
Correct Answer
B. 115°Explanation
Angle D can be determined by using the fact that the sum of the angles in a triangle is 180°. Since angle D is not given directly, we can find it by subtracting the sum of the other two given angles (65° and 90°) from 180°. 65° + 90° = 155°. 180° - 155° = 25°. However, this contradicts the given answer of 115°. Therefore, it seems that the given answer is incorrect or there is missing information, making it difficult to provide a definitive explanation.Rate this question:
-
- 13.
In what shape/shapes are the diagonals perpendicular?
- A.
Rectangle
- B.
Rhombus
- C.
Square
- D.
Trapezoid
- E.
All of the above
Correct Answer
B. RhombusExplanation
A rhombus is a quadrilateral with all sides of equal length. In a rhombus, the diagonals bisect each other at right angles, making them perpendicular. Therefore, the statement "diagonals are perpendicular" is true for a rhombus.Rate this question:
-
- 14.
In what shape/shapes do the diagonals bisect each other?
- A.
Parallelogram
- B.
Rectangle
- C.
Rhombus
- D.
Square
- E.
All of the above
Correct Answer
E. All of the aboveExplanation
The diagonals of a parallelogram, rectangle, rhombus, and square bisect each other. In each of these shapes, the diagonals intersect at their midpoints, dividing each diagonal into two equal segments. Therefore, the correct answer is "All of the above."Rate this question:
-
- 15.
In what shape/shapes are the sides all congruent?
- A.
Rectangle
- B.
Rhombus
- C.
Parallelogram
- D.
Trapezoid
- E.
None of the above
Correct Answer
B. RhombusExplanation
A rhombus is the only shape listed where all of the sides are congruent. A rectangle has two pairs of congruent sides, but not all four sides are congruent. A parallelogram has opposite sides that are congruent, but not all four sides are congruent. A trapezoid has at least one pair of parallel sides, but not all four sides are congruent. Therefore, the correct answer is rhombus.Rate this question:
-
- 16.
Medians exist in which of the following shape/shapes?
- A.
Parallelogram
- B.
Trapezoid
- C.
Rhombus
- D.
Square
- E.
All of the above
Correct Answer
E. All of the aboveExplanation
Medians exist in all the mentioned shapes: Parallelogram, Trapezoid, Rhombus, and Square. A median of a shape is a line segment connecting a vertex to the midpoint of the side opposite that vertex.Rate this question:
-
- 17.
Which of the following shapes has opposites sides that are congruent?
- A.
Parallelogram
- B.
Rectangle
- C.
Rhombus
- D.
Square
- E.
All of the above
Correct Answer
E. All of the aboveExplanation
All of the shapes listed (parallelogram, rectangle, rhombus, and square) have opposite sides that are congruent. In a parallelogram, opposite sides are parallel and congruent. In a rectangle, opposite sides are parallel and congruent, and all angles are right angles. In a rhombus, opposite sides are parallel and congruent, and all sides are congruent. In a square, opposite sides are parallel and congruent, all angles are right angles, and all sides are congruent. Therefore, all of these shapes have opposite sides that are congruent.Rate this question:
-
- 18.
Pi Day is Monday March 14th
- A.
True
- B.
False
Correct Answer
A. TrueExplanation
Pi Day is celebrated on March 14th each year, as the numerical representation of the date (3/14) corresponds to the first three digits of the mathematical constant pi (3.14). This day is observed by math enthusiasts and educators around the world to honor the significance of pi in mathematics and its applications in various fields. Therefore, the statement "Pi Day is Monday March 14th" is true.Rate this question:
-
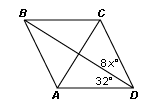
- 19.
In the rhombus, what is x?
- A.
90
- B.
38
- C.
128
- D.
180
Correct Answer
B. 38Explanation
The given question asks for the value of x in a rhombus. A rhombus is a quadrilateral with all sides equal in length. In a rhombus, opposite angles are equal. Since the sum of all angles in a quadrilateral is 360 degrees, and opposite angles in a rhombus are equal, each angle in a rhombus measures 180 degrees divided by 2, which is 90 degrees. Therefore, x cannot be 90 or 180 degrees. The only option left is 38 degrees, which is the correct answer.Rate this question:
-
- 20.
2In the rhombus below, what is x?
- A.
40
- B.
90
- C.
80
- D.
45
Correct Answer
A. 40Explanation
In a rhombus, all four sides are equal in length. Additionally, the opposite angles are congruent. Therefore, since the given angle measures are 40, 90, 80, and 45 degrees, the opposite angles must also be congruent. The only angle that is repeated in the given options is 40 degrees, so it must be the value of x.Rate this question:
-
- 21.
In the parallelogram below, what is x?
- A.
139
- B.
119
- C.
34.75
- D.
20.25
Correct Answer
C. 34.75 -
- 22.
In the rhombus below, what is x?
- A.
7.25
- B.
32
- C.
4
- D.
97.25
Correct Answer
C. 4 -
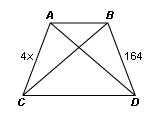
- 23.
If ABCD is an Isosceles trapezoid, what is x?
- A.
164
- B.
41
- C.
45
- D.
160
Correct Answer
B. 41Explanation
In an isosceles trapezoid, the two non-parallel sides are congruent. Since the given trapezoid is isosceles, we can conclude that the lengths of AB and CD are equal. Therefore, the base angles opposite to these sides are also equal. In the given figure, x is the measure of one of these base angles. Since the trapezoid is isosceles, the measure of each base angle is equal. Therefore, x is equal to the measure of the other base angle, which is 41.Rate this question:
-
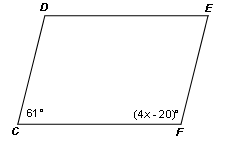
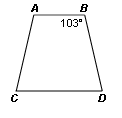
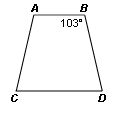
- 24.
In the trapezoid below, what is the measure of angle A?
- A.
103°
- B.
77°
- C.
90°
- D.
180°
Correct Answer
A. 103°Explanation
The measure of angle A in a trapezoid can be determined by using the fact that the opposite angles in a trapezoid are supplementary. Since angle A is opposite to the given angle of 103°, the measure of angle A is also 103°.Rate this question:
-
- 25.
In the trapezoid below, what is the measure of angle C?
- A.
103°
- B.
77°
- C.
90°
- D.
180°
Correct Answer
B. 77°Explanation
The measure of angle C in a trapezoid can be determined by recognizing that the sum of the interior angles of a trapezoid is always equal to 360 degrees. Since the other three angles given (103°, 90°, and 180°) add up to 373°, which is greater than 360°, they cannot be the measure of angle C. Therefore, the correct answer is 77° as it is the only remaining option.Rate this question:
-
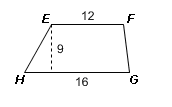
- 26.
In the trapezoid below, what is x if AD=14 and BC=7+2x?
- A.
7
- B.
3.5
- C.
14
- D.
10.5
Correct Answer
B. 3.5Explanation
In a trapezoid, the bases are parallel. In this case, AD is one base and BC is the other base. It is given that AD is 14 units long. It is also given that BC is equal to 7 plus 2 times x. To find the value of x, we need to set up an equation. BC = 7 + 2x. Since AD and BC are parallel, they must be equal in length. Therefore, 14 = 7 + 2x. Solving this equation, we find that x = 3.5. Hence, the correct answer is 3.5.Rate this question:
-
- 27.
Find the area:
- A.
1728
- B.
252
- C.
126
- D.
144
Correct Answer
C. 126 -
- 28.
Find the area:
- A.
300
- B.
150
- C.
35
- D.
200
Correct Answer
A. 300 -
- 29.
Find the area:
- A.
120
- B.
60
- C.
23
- D.
30
Correct Answer
A. 120Explanation
The given numbers do not provide enough information to determine the type of area being referred to. Without further context, it is not possible to determine the correct answer.Rate this question:
-
- 30.
Find the area if
- A.
280
- B.
560
- C.
140
- D.
48
Correct Answer
A. 280 -
- 31.
Find the area:
- A.
124.68
- B.
62.34
- C.
748.08
- D.
374.04
Correct Answer
D. 374.04Explanation
The given answer, 374.04, is the correct answer for finding the area. However, without any context or further information provided, it is not possible to determine what exactly is being referred to as the "area". It could be the area of a shape, such as a square or a rectangle, or it could be the area of a region or a land plot. Without additional details, the exact meaning of "area" remains unclear.Rate this question:
-
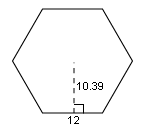
- 32.
Find the area:
- A.
162
- B.
88
- C.
142
- D.
112
Correct Answer
C. 142 -
- 33.
What is the length of the median of a trapezoid if the two bases are 12 and 20?
- A.
16
- B.
32
- C.
240
- D.
120
Correct Answer
A. 16Explanation
The length of the median of a trapezoid is equal to the average of the lengths of its two bases. In this case, the two bases are 12 and 20. Adding them together and dividing by 2 gives us (12 + 20) / 2 = 32 / 2 = 16. Therefore, the length of the median is 16.Rate this question:
-
- 34.
How many sides does a pentagon have?
- A.
5
- B.
6
- C.
7
- D.
8
Correct Answer
A. 5Explanation
A pentagon is a polygon with five sides. Therefore, the correct answer is 5.Rate this question:
-
- 35.
The diagonals in all parallelograms bisect each other
- A.
True
- B.
False
Correct Answer
A. TrueExplanation
In a parallelogram, the opposite sides are parallel and equal in length. Since the diagonals of a parallelogram connect opposite vertices, they also bisect each other. This means that the point where the diagonals intersect divides each diagonal into two equal parts. Therefore, the statement that the diagonals in all parallelograms bisect each other is true.Rate this question:
-
- 36.
In a rhombus, the diagonals bisect the angles
- A.
True
- B.
False
Correct Answer
A. TrueExplanation
The statement is true because in a rhombus, the diagonals intersect each other at right angles. Since the diagonals of a rhombus are perpendicular, they divide the angles into two equal parts. Therefore, the diagonals bisect the angles of a rhombus.Rate this question:
-
- 37.
The diagonals in all parallelograms are congruent
- A.
True
- B.
False
Correct Answer
B. FalseExplanation
The statement that the diagonals in all parallelograms are congruent is false. In a parallelogram, the diagonals bisect each other, meaning they divide each other into two equal halves. However, they are not necessarily congruent, as their lengths can vary depending on the shape and size of the parallelogram. Therefore, the correct answer is false.Rate this question:
-
- 38.
The legs in a trapezoid are always congurent
- A.
True
- B.
False
Correct Answer
B. FalseExplanation
The statement is false because the legs of a trapezoid are not always congruent. A trapezoid is a quadrilateral with only one pair of parallel sides. The legs of a trapezoid are the non-parallel sides. In most cases, the legs of a trapezoid are not congruent, unless it is a special type of trapezoid called an isosceles trapezoid, where the legs are congruent. Therefore, the statement that the legs in a trapezoid are always congruent is incorrect.Rate this question:
-
- 39.
What is the equation for the sum of the interior angles of a polygon?
- A.
(n-2) * 360
- B.
N * 180
- C.
(n-2) * 180
- D.
360
Correct Answer
C. (n-2) * 180Explanation
The equation for the sum of the interior angles of a polygon is (n-2) * 180. This formula is derived from the fact that a polygon can be divided into (n-2) triangles, where n is the number of sides of the polygon. Since the sum of the angles in a triangle is always 180 degrees, multiplying (n-2) by 180 gives the total sum of the interior angles of the polygon.Rate this question:
-
- 40.
What is the equation for the area of a trapezoid?
- A.
A = b * h
- B.
A = 1/2 b * h
- C.
A = 1/2 D1 * D2
- D.
A = 1/2 * h * (b1 + b2)
Correct Answer
D. A = 1/2 * h * (b1 + b2)Explanation
The equation for the area of a trapezoid is given by A = 1/2 * h * (b1 + b2), where h represents the height of the trapezoid, and b1 and b2 represent the lengths of the parallel bases. This formula calculates the area by taking the average of the lengths of the two bases and multiplying it by the height. The 1/2 factor is included because the area of a trapezoid is equal to half the product of the height and the sum of the lengths of the bases.Rate this question:
-
- 41.
What is the equation for the area of a rhombus?
- A.
A = b * h
- B.
A = 1/2 b * h
- C.
A = 1/2 D1 * D2
- D.
A = 1/2 * h * (b1 + b2)
Correct Answer
C. A = 1/2 D1 * D2Explanation
The equation for the area of a rhombus is A = 1/2 D1 * D2. This formula calculates the area by multiplying half of the length of one diagonal (D1) by half of the length of the other diagonal (D2). Since a rhombus has congruent opposite angles and sides, this formula can be used to find the area regardless of the lengths of the sides.Rate this question:
-
- 42.
What is the equation for the area of a parallelogram?
- A.
A = b * h
- B.
A = 1/2 b * h
- C.
A = 1/2 D1 * D2
- D.
A = 1/2 * h * (b1 + b2)
Correct Answer
A. A = b * hExplanation
The equation A = b * h represents the area of a parallelogram. The formula states that the area (A) is equal to the base (b) multiplied by the height (h). This formula is commonly used to calculate the area of a parallelogram, where the base is one of the sides of the parallelogram and the height is the perpendicular distance between the base and the opposite side.Rate this question:
-
- 43.
Consecutive angels in a parallelogram are ___.
- A.
Congruent
- B.
Complimentary
- C.
Supplementary
- D.
None of the above
Correct Answer
C. SupplementaryExplanation
Consecutive angles in a parallelogram are supplementary. This means that the sum of two consecutive angles in a parallelogram is always 180 degrees. This is a property of parallelograms, where opposite angles are equal and consecutive angles are supplementary. Therefore, the correct answer is "supplementary".Rate this question:
-
- 44.
What is the equation for the area of a parallelogram?
- A.
A = b * h
- B.
A = 1/2 b * h
- C.
A = 1/2 D1 * D2
- D.
A = 1/2 * h * (b1 + b2)
Correct Answer
A. A = b * hExplanation
The equation for the area of a parallelogram is A = b * h, where b represents the base of the parallelogram and h represents the height. This formula calculates the area by multiplying the base length by the height of the parallelogram.Rate this question:
-
- 45.
All squares are parallelograms
- A.
True
- B.
False
Correct Answer
A. TrueExplanation
The statement "All squares are parallelograms" is true because a square is a special type of parallelogram. A parallelogram is a quadrilateral with opposite sides that are parallel and equal in length. In a square, all four sides are equal in length and all angles are right angles, which satisfies the conditions of a parallelogram. Therefore, every square can be classified as a parallelogram.Rate this question:
-
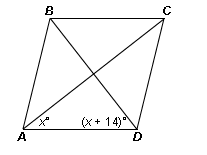
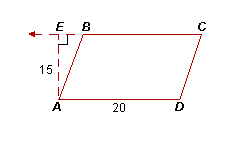
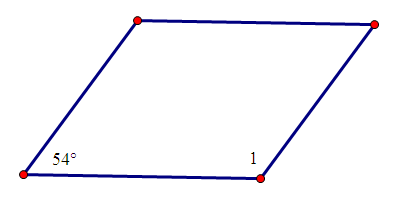
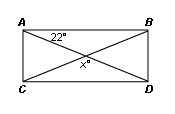
- 46.
What is the measure of angel 1 in the parallelogram below?
- A.
54°
- B.
126°
- C.
36°
- D.
180°
Correct Answer
B. 126°Explanation
In a parallelogram, opposite angles are congruent. Therefore, if angle 1 measures 126°, then the opposite angle, angle 3, must also measure 126°.Rate this question:
-
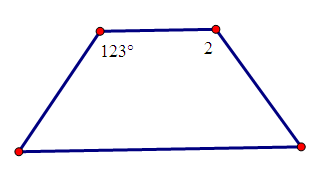
- 47.
What is the measure of angle 2 in the isosceles trapezoid below?
- A.
123°
- B.
57°
- C.
33°
- D.
180°
Correct Answer
A. 123°Explanation
In an isosceles trapezoid, the opposite angles are congruent. Angle 2 is opposite to the angle measuring 57°, so it must also measure 57°. Therefore, the given answer of 123° is incorrect.Rate this question:
-
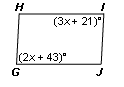
- 48.
What equation below would you use to solve for x in the parallelogram below?
- A.
2x+43+3x+21=180
- B.
2x+43=3x+21
- C.
2x+43+3x+21=90
- D.
2x+43=90
Correct Answer
B. 2x+43=3x+21Explanation
To solve for x in the parallelogram, we can use the equation 2x+43=3x+21. This equation represents the sum of the opposite angles in a parallelogram, which are congruent. By subtracting 2x from both sides, we can isolate the x term on one side of the equation. This allows us to solve for x by subtracting 21 from both sides. The resulting equation, 2x+43=3x+21, allows us to determine the value of x in the parallelogram.Rate this question:
-
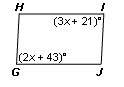
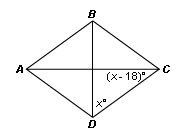
- 49.
What equation below can you use to solve for x in the rhombus below?
- A.
X+x-18=180
- B.
90+x+x-18=180
- C.
90+x+x-18=90
- D.
90+x+x+18=180
Correct Answer
B. 90+x+x-18=180Explanation
The equation 90+x+x-18=180 can be used to solve for x in the rhombus. This equation represents the sum of the four angles of a rhombus, which is equal to 360 degrees. By simplifying the equation, we can solve for x and find the value of the unknown angle in the rhombus.Rate this question:
-
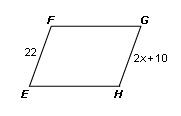
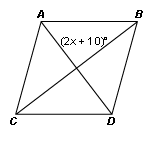
- 50.
What equation would you use to solve for x in the rhombus below?
- A.
2x+10=180
- B.
2x+10=90
- C.
2x+10+90=180
- D.
2x+10+2x+10=180
Correct Answer
B. 2x+10=90Explanation
To solve for x in the given rhombus, the equation 2x+10=90 is used. This equation represents the sum of the two opposite angles in a rhombus, which is equal to 180 degrees. By simplifying the equation, x can be found by subtracting 10 from both sides and dividing by 2.Rate this question:
-
Quiz Review Timeline +
Our quizzes are rigorously reviewed, monitored and continuously updated by our expert board to maintain accuracy, relevance, and timeliness.
-
Current Version
-
Jan 29, 2024Quiz Edited by
ProProfs Editorial Team -
Mar 09, 2011Quiz Created by
Smatook
 Back to top
Back to top