SAT Section 2 Math
This is Section 2 of the SAT. You are allowed to use a calculator, but it is not required. Fill in the bubble for the best answer to each question.
- 1.
Marigolds are to be planted inside a circular flower garden so that there are 4 marigolds per square foot. The circumference of the garden is 20 feet. If marigolds are available only in packs of 6, how many packs of 6 flowers are needed?
- A.
9
- B.
25
- C.
30
- D.
34
- E.
39
Correct Answer
B. 25Explanation
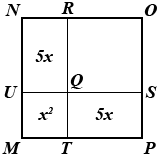
Since M N O P andQ R O S are squares, M N = N O = 8 and Q R = R O. Thus, M U = N R and the rectangle with area x^2 must be a square with sides of length x. The rectangles with area 5 times x must have sides of length x and 5. Thus, the area of Q R O S is equal to 5^2 = 25.
Another approach is the following. Since Q R O S is a square, Q R = R O. Thus, M U = N R and M U Q T is a square with sides of length x. The rectangles with area 5 times x must have sides of length x and 5. Since the area of M N O P is 64:
(x + 5)^2 = 64
x^2 + (5 times x) + (5 times x) + 25 = 64
Thus, the area of Q R O S is 25.Rate this question:
-
- 2.
In the xy-plane, the points with coordinates (0, -5) and (6, -2) lie on line l. Line p contains the point with coordinates (-5, 0) and is perpendicular to line l. What is the x-coordinate of the point where lines l and p intersect?
- A.
6
- B.
13
- C.
14
- D.
20
- E.
22
Correct Answer
E. 22Explanation
The first step is to determine the area of the garden. Since the circumference is 20 feet, the radius is 20 over (2 times pi) = 10 over pi and the area is pi times (r^2) or pi times ((10 over pi)^2) = 100 over pi square feet. If there are 4 marigolds per square foot, 4 times (100 over pi) marigolds are needed. To find out how many packs of 6 flowers are needed, divide 400 over pi by 6. The result is 21.22; thus, 22 packs would be needed. The correct answer is E.Rate this question:
-
- 3.
The line with equation y=7 is graphed on the same xy-plane as the circle with center (4, 5) and radius 3. What are the x-coordinates of the points of intersection of the line and the circle?
- A.
-6
- B.
-5
- C.
-4
- D.
-3
- E.
-2
Correct Answer
E. -2Explanation
It is often helpful to make a sketch for this type of question.
(Graphic)
The slope of line l is equal to negative 5 minus (negative 2) over (0 minus 6) = negative 3 over negative 6 = 1 over 2.
Since negative 5 is the y-intercept, the equation of l is y = ((1 over 2) times x) minus 5.
Since line p is perpendicular to line l, the slope of p is equal to negative 2 (the negative reciprocal of the slope of l ). Since line p contains the point with coordinates(negative 5 comma 0), the equation of line p can be found by substitution.
Stacked Equation
To find the x coordinate of the point where the two lines intersect, solve the equation ((1 over 2) times x) minus 5 = negative (2 times x) minus 10. The lines intersect at the point where x = negative 2.
It is also possible to graph the two linear equations to determine where they intersect. Using a graphing calculator, let y_1 = ((1 over 2) times x) minus 5 and y_2 = negative (2 times x) minus 10. You can find the point of intersection graphically in the standard viewing window, or you can examine a table of values to find the x-value where y_1 = y_2.Rate this question:
-
- 4.
The figure above shows a square region divided into four rectangular regions, three of which have areas 5x, 5x, and , respectively. If the area of MNOP is 64, what is the area of square QROS?
- A.
10
- B.
20
- C.
25
- D.
30
- E.
40
Correct Answer
A. 10Explanation
One way to solve this problem is to set up an equation using one variable. Let n represent the number of 3 dollars tickets sold; then 50 minus n represents the number of 5 dollars tickets sold.
(3 times n) + (5 times (50 minus n)) = 230
(3 times n) +250 minus (5 times n) = 230
20 = 2 times n
10 = n
There were 10 3 dollar tickets sold.
Simultaneous equations can also be set up to solve the problem. Let n represent the number of 3 dollar tickets sold and p represent the number of 5 dollar tickets sold.
n + p = 50
(3 times n) + (5 times p) = 230
Another way to solve this problem is to realize that each of the tickets costs at least 3 dollars. At 3 dollars each, a total of 150 dollars would be collected from selling all 50 of the tickets. Since the remaining 80 dollars was the extra 2 dollars collected from each of the 5 dollar tickets, 40 of the 5 dollar tickets were sold. This implies that 10 of the 3 dollar tickets were sold.Rate this question:
-
- 5.
The dimensions of a rectangular solid are 3 inches by 4 inches by 5 inches. The length of each edge of the solid is to be increased by 20%. What is the surface area, in square inches, of the new solid?
- A.
-5 and 5
- B.
-1 and 1
- C.
1.35 and 6. 65
- D.
1.76 and 6.24
- E.
2 and 6
Correct Answer
D. 1.76 and 6.24Explanation
For this question, it may help to draw a diagram.
Graphic
The line y = 7 intersects the circle in 2 points. These two points and the center of the circle form a triangle with two sides of length 3 (since the radius of the circle is 3) and with height 2. (The distance between the point (4 comma 5) and the line y = 7 is the distance between (4 comma 5) and (4 comma 7).)
Graphic
Using the Pythagorean theorem, you can find that A B = square root 5. Point B has x-coordinate 4. Therefore, the x-coordinate of the points of intersection are 4 minus square root 5 and 4 + square root 5 or 1.76 and 6.24. The correct answer is D.
You could also solve the problem algebraically. A circle with center (4 comma 5) and radius 3 has equation (x minus 4)^2 + (y minus 5)^2 = 9. Substitute y = 7 into the equation, and it simplifies to (x minus 4 ) ^2 = 5. Solving for x produces the two x-coordinates of the points of intersection of the circle and the line.Rate this question:
-
- 6.
What is the largest integer, n, for which 297/(3n) is an integer?
- A.
1
- B.
2
- C.
3
- D.
4
- E.
5
Correct Answer
C. 3Explanation
297/3 = 99 ; 99/3 = 33 ; 33/3 = 11. So, n = 3.
Note 297/(33) = 297/27 = 11, whereas 297/(34) = 297/81 = 3.67.Rate this question:
-
- 7.
How old will Mr. Winkle be p years from now if he was q years old r years ago?
- A.
P − q − r
- B.
P + q + r
- C.
P − q + r
- D.
p + q − r
- E.
−p + q + r
Correct Answer
B. P + q + rExplanation
Mr. Winkle is presently (q + r) years old.
Therefore, he will be (p + q + r) years old p years from now.
If the problem appears confusing, choose an easy set of numbers,
e.g., p = 7; q = 50 and r = 3.
Then, Mr. Winkle was 50 years old 3 years ago.
Therefore, he is 53 and will be 60 years old 7 years from now.
Note that p + q + r = 7 + 50 + 3 = 60.Rate this question:
-
- 8.
An employee wishes to distribute a total of $35,000 among four of his employees. What is the largest amount an employee will get if the money is distributed among them in the ratio of 1:2:3:4?
- A.
$3,500
- B.
$5,000
- C.
$7,000
- D.
$4,000
- E.
$20,000
Correct Answer
D. $4,000Explanation
Let the distributed amounts be m, 2m, 3m and 4m. Then,
m + 2m + 3m + 4m = 35000 or 10m = 35000. Thus, m = 3500 or 4m = 14000.Rate this question:
-
- 9.
Two-fifths of the caps in a box are red, one-fourth are blue, and the rest are green. What is the ratio of the green caps to the blue caps?
- A.
5:8
- B.
5:7
- C.
7:8
- D.
8:7
- E.
7:5
Correct Answer
E. 7:5Explanation
Let there be y caps in the box. Then, there are 2y/5 red caps.
One-fourth of the caps are blue. So, there are y/4 blue caps.
Therefore, number of green caps = y − 2y/5 − y/4 =(20y − 8y − 5y)/20 = 7y/20.
Ratio of green caps to blue caps = (7/20) ÷ (1/4) = (7/20) × 4 = 7/5Rate this question:
-
- 10.
Your math marks decreased by 60% from the first quiz to the second quiz. If your marks in the third quiz are identical to those in the first quiz, by what percent did your marks increase from the second to third quiz?
- A.
60%
- B.
100%
- C.
140%
- D.
150%
- E.
250%
Correct Answer
D. 150%Explanation
Let m be your marks in the first quiz.
Then, your marks are 0.4m in the second quiz and m in the third quiz.
Percent Increase
= (Marks in third Quiz - Marks in second quiz) × 100/Marks in second quiz
= (m − 0.4m) × 100 / 0.4m = (0.6/0.4) × 100 = 150.Rate this question:
-
- 11.
What is the largest of the four consecutive even integers whose sum is 892?
- A.
218
- B.
220
- C.
222
- D.
226
- E.
230
Correct Answer
D. 226Explanation
Let the largest of the four consecutive even integers be m. Then,
m + (m − 2) + (m − 4) + (m − 6) = 892 or 4m − 12 = 892.
Thus, m = 904 / 4 = 226.
The four consecutive even integers are 220, 222, 224 and 226.Rate this question:
-
- 12.
If 6y -- 4z = 47 and y + 11z = 2, what is the average (arithmetic mean) of y and z?
- A.
4.5
- B.
3.5
- C.
9
- D.
7
- E.
5
Correct Answer
B. 3.5Explanation
Adding the equations gives 7y + 7z = 49.
Dividing by 7 gives y + z = 7.
So, Arithmetic Mean = (y + z)/2 = 7/2 = 3.5.Rate this question:
-
- 13.
A quiz consists of true and false questions. The ratio of the number of true questions to the number of false questions is 4:3. About what percent of the questions are false?
- A.
43%
- B.
57%
- C.
67%
- D.
83%
- E.
86%
Correct Answer
A. 43%Explanation
Percent of false questions = (3/7) × 100 = 42.86.
So, about 43% of the questions are false.
Alternatively, one could reason out without any calculations as follows.
For every 4 true questions, there are 3 false questions. So, there are fewer false questions than true ones, i.e., less than 50% of the questions are
false. The only option is 43%.Rate this question:
-
- 14.
What is the smallest prime factor of 1,001?
- A.
3
- B.
5
- C.
7
- D.
11
- E.
13
Correct Answer
C. 7Explanation
Now, 1001 is divisible by neither 3 nor 5.
So, let's try to divide by 7. We find 1001 / 7 = 143 = 13 × 11.
Since 1001 = 13 × 11 × 7, the smallest prime factor is 7.Rate this question:
-
- 15.
What is z if 2.5% of z equals 75% of 50?
- A.
10
- B.
25
- C.
50
- D.
75
- E.
1500
Correct Answer
E. 1500Explanation
Now, (2.5/100) z = (75/100) 50. So, z = (75/2.5) 50 = (750/25) 50 = 1500.
Alternatively, 75 is 30 times 2.5. So, z must be 30 times 50.
Of the given options, only 1500 is large enough.
To save time, you can sometimes guess the answer without doing detailed computations.Rate this question:
-
- 16.
An agent receives a commission of 40 cents for every $50 of business she procures. What percent is the agent's commission?
- A.
0.8%
- B.
1.0%
- C.
1.25%
- D.
1.5%
- E.
2.5%
Correct Answer
A. 0.8%Explanation
Percent Commission = (0.40/50) × 100 = 40/50 = 0.8.
Alternatively, 1% of $50 is 50 cents. Since the commission is 40 cents, it is less than 1%. So, the only option is 0.8%.Rate this question:
-
- 17.
What is the smallest of four consecutive odd integers whose sum is 968?
- A.
235
- B.
239
- C.
243
- D.
245
- E.
249
Correct Answer
B. 239Explanation
Let the smallest of the four consecutive odd integers be m. Then,
m + (m + 2) + (m + 4) + (m + 6) = 968 or 4m + 12 = 968.
Thus, m = 956 / 4 = 239.
The four consecutive odd integers are 239, 241, 243 and 245.Rate this question:
-
- 18.
A small square region inside a larger square is shaded. The diagonal of the larger square is three times the diagonal of the small square in length. What fraction of the area of the larger square is not shaded?
- A.
1/9
- B.
1/3
- C.
2/3
- D.
8/9
- E.
Cannot be determined
Correct Answer
D. 8/9Explanation
Let b be the side of the square and d the length of its diagonal.
Then, by Pythagoras theorem, d2 = b2 + b2 = 2 b2.
Since the diagonal of the larger square is 3 times that of the small square,
the side of the larger square is 3 times the side of the small square.
If b is the side of the small shaded square, then its area = b2.
Now, 3b is the side of the larger square and its area = 9b2.
Thus, unshaded area = 8b2 and
fraction of the area of the larger square unshaded = 8/9.Rate this question:
-
- 19.
The arithmetic mean (average) of 3, 5, 7, and y is 100. What is the value of y?
- A.
85
- B.
95
- C.
105
- D.
115
- E.
385
Correct Answer
E. 385Explanation
The arithmetic mean of n numbers is their sum divided by n.
So, (3 + 5 + 7 + y) / 4 = 100 or 15 + y = 100 × 4 = 400.
Thus, y = 400 − 15 = 385.
Given that 3, 5 and 7 are small numbers and the average 100 is relatively large, it is possible to guess the answer as 385.Rate this question:
-
- 20.
What is 2% of 7%?
- A.
0.014%
- B.
0.09%
- C.
0.14%
- D.
1.4%
- E.
14%
Correct Answer
C. 0.14%Explanation
2% of 7 = (2/100) × 7 = 14/100 = 0.14. So, 2% of 7% = 0.14%.Rate this question:
-
Quiz Review Timeline +
Our quizzes are rigorously reviewed, monitored and continuously updated by our expert board to maintain accuracy, relevance, and timeliness.
-
Current Version
-
Mar 20, 2023Quiz Edited by
ProProfs Editorial Team -
Jul 01, 2010Quiz Created by
Ihatesats


