Linear Functions Of Slope Quiz

Approved & Edited by ProProfs Editorial Team

The ProProfs editorial team is comprised of experienced subject matter experts. They've collectively created over 10,000 quizzes and lessons, serving over 100 million users. Our team includes in-house content moderators and subject matter experts, as well as a global network of rigorously trained contributors. All adhere to our comprehensive editorial guidelines, ensuring the delivery of high-quality content.

Learn about Our Editorial Process

| By Tjkim

Tjkim

Community Contributor

Quizzes Created: 25 | Total Attempts: 77,032

Questions: 13 | Attempts: 998 | Updated: Aug 6, 2024

Questions

Settings

Feedback

During the Quiz End of Quiz

Difficulty

Sequential Easy First Hard First

Create your own Quiz

Share on Facebook Share on Twitter Share on Whatsapp Share on Pinterest Share on Email Copy to Clipboard Embed on your website

When it comes to linear functions, the slope of a line or hill is calculated by the ratio of the vertical change between two points to the horizontal change between those two same points. Can you calculate it?

Questions and Answers

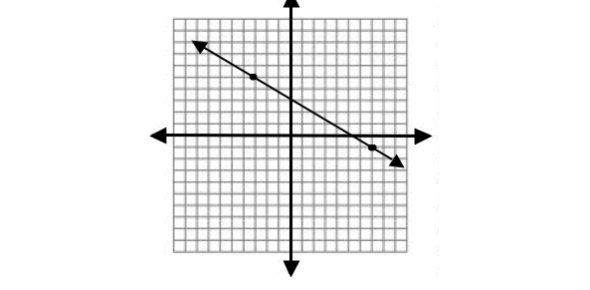
1.

Find the slope of the line.

Explanation
The answer options provided are all equivalent representations of the same value, which is the slope of the line. The slope is a measure of how steep a line is, and it represents the ratio of the vertical change (rise) to the horizontal change (run) between any two points on the line. In this case, the slope is 1/4 or 0.25 or 0.25, all of which indicate that for every 1 unit increase in the horizontal direction, the line increases by 1/4 or 0.25 or 0.25 units in the vertical direction.

Rate this question:

5
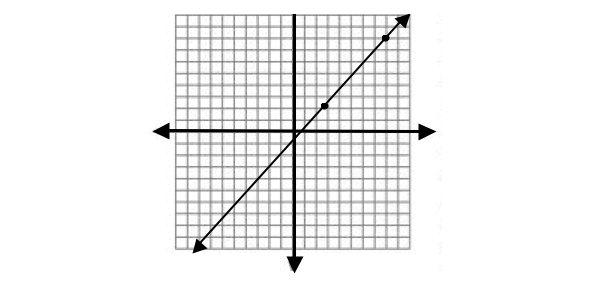
2.

Find the slope of the line.

Explanation
The answer choices provided are three different representations of the same number: 1/5, 0.2, and 0.2. They all represent the slope of the line. The slope of a line measures the steepness of the line and is calculated as the change in the y-coordinates divided by the change in the x-coordinates. In this case, all three representations indicate that the line has a slope of 1/5 or 0.2 or 0.2.

Rate this question:

2
3.

Find the slope of the line.

Explanation
The given answer options are 3/5, 0.6, and 0.6. These options represent the slope of the line. The slope of a line is a measure of how steep the line is. It is calculated by dividing the change in the y-coordinate by the change in the x-coordinate between two points on the line. In this case, the slope can be represented as 3/5, 0.6, or 0.6.

Rate this question:

2
4.

Find the slope of the line.
5.

Find the slope of the line.
6.

Find the slope of the line.

Explanation
The slope of a line is a measure of how steep the line is. In this case, the given answer of 1 suggests that the line has a slope of 1, which means that for every unit increase in the x-coordinate, the y-coordinate also increases by 1. This indicates a positive and constant rate of change between the x and y coordinates of the line.

Rate this question:
7.

Find the slope of the line.

Explanation
The slope of a line is a measure of how steep the line is. In this case, the given answer options of .5, 0.5, and 1/2 all represent the same value, which is the slope of the line. This means that the line has a slope of 1/2, which indicates that for every increase of 1 unit in the x-coordinate, the y-coordinate increases by 1/2 unit.

Rate this question:
8.

Find the slope of the line.

Explanation
The slope of a line is a measure of how steep the line is. In this case, both 1.5 and 3/2 represent the same value, which is the slope of the line. Therefore, either 1.5 or 3/2 can be considered the correct answer for the slope of the line.

Rate this question:
9.

Find the slope of the line.

Explanation
The given answer is a list of three values: 3/8, .325, and 0.325. These values do not represent the slope of a line. A slope is a single numerical value that represents the steepness of a line. Therefore, the explanation is that the given answer is incorrect.

Rate this question:
10.

Find the slope of the line.

Explanation
The slope of a line is a measure of how steep the line is. In this case, the given answer of 6 indicates that the line has a slope of 6. This means that for every unit increase in the x-coordinate, the y-coordinate increases by 6 units.

Rate this question:
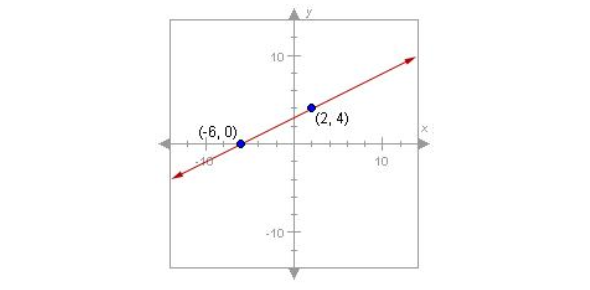
11.

Find the slope of the line.
- A.
  
  0
- B.
  
  Undefined
- C.
  
  0/12
- D.
  
  12/0
Correct Answer
A. 0

Explanation
The slope of a line is determined by the change in the y-coordinates divided by the change in the x-coordinates between two points on the line. In this case, the line has a constant y-coordinate of 0, which means that there is no change in the y-coordinate regardless of the change in the x-coordinate. Therefore, the slope of the line is 0.

Rate this question:
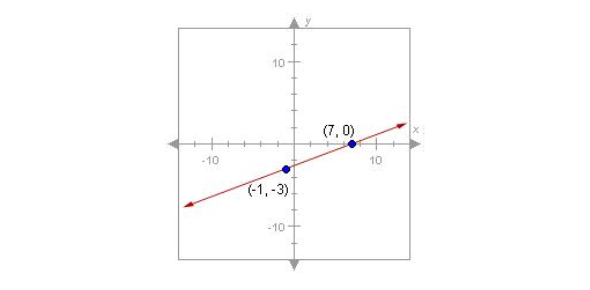
12.

Find the slope of the line.
- A.
  
  -6/10
- B.
  
  6/10
- C.
  
  -3/5
- D.
  
  3/5
Correct Answer
C. -3/5

Explanation
The slope of a line is defined as the change in y-coordinates divided by the change in x-coordinates between any two points on the line. In this case, the slope is -3/5. This means that for every 5 units we move to the right on the x-axis, we move 3 units downwards on the y-axis. The negative sign indicates that the line is decreasing as we move from left to right.

Rate this question:

0
13.

Find the slope of the line.
- A.
  
  -5/-6
- B.
  
  5/6
- C.
  
  -5/6
- D.
  
  -6/5
- E.
  
  6/5
Correct Answer
E. 6/5

Explanation
The slope of a line is determined by the ratio of the change in the y-coordinates to the change in the x-coordinates between two points on the line. In this case, the slope is 6/5 because the numerator represents a change in the y-coordinate of 6 and the denominator represents a change in the x-coordinate of 5. This means that for every 5 units the x-coordinate increases, the y-coordinate increases by 6 units.

Rate this question:

2