Ideal Gas Law Lesson: Key Principles, Examples, and Calculations
Lesson Overview
- What Is the Ideal Gas Law?
- What Is the History of the Ideal Gas Law?
- How Do These Laws Combine into the Ideal Gas Law?
- How Does the Combined Gas Law Work?
- What Are the Properties of Gases in Relation to the Gas Laws?
- How Are Moles of Gas Calculated Using the Gas Laws?
- Conclusion
Gases are all around us, from the air we breathe to the fuel that powers engines. But how do we describe their behavior in a predictable way? The Ideal Gas Law is a powerful equation that connects four key properties of gases: pressure, volume, temperature, and the number of particles. This lesson explores the fundamental principles behind the Ideal Gas Law, how it builds on simpler gas laws, and how to use it for real-world calculations. With clear examples and step-by-step problem solving, you'll learn how this law helps scientists and engineers model everything from weather patterns to chemical reactions.
What Is the Ideal Gas Law?
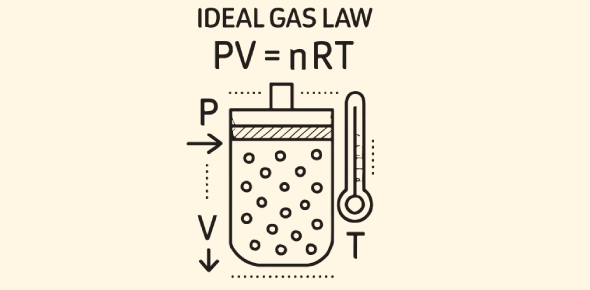
The Ideal Gas Law is a fundamental equation in chemistry and physics that describes the behavior of an "ideal" gas-a hypothetical gas that perfectly follows a set of assumptions about molecular behavior. It is represented by the equation
PV=nRT
In this equation
- P represents the pressure of the gas,
- V is the volume of the gas,
- n is the number of moles of the gas,
- R is the universal gas constant, and
- T is the absolute temperature of the gas measured in Kelvin.
The Ideal Gas Law combines several simpler gas laws-Boyle's Law, Charles's Law, Avogadro's Law, and Gay-Lussac's Law-into a single comprehensive equation. Each of these individual laws describes the relationship between two of the four variables (pressure, volume, temperature, and moles) while holding the others constant. The Ideal Gas Law brings these relationships together, showing how the state of a gas is affected when any of these variables change.
What Is the History of the Ideal Gas Law?
The Ideal Gas Law has its roots in a series of discoveries made over more than two centuries. Scientists studying gases noticed patterns in how gases respond to changes in pressure, volume, and temperature. These observations led to several individual gas laws:
- Boyle's Law (1662): Robert Boyle discovered that for a fixed amount of gas at constant temperature, pressure and volume are inversely proportional.
- Charles's Law (1787): Jacques Charles found that at constant pressure, the volume of a gas increases proportionally with temperature.
- Gay-Lussac's Law (1809): Joseph Gay-Lussac observed that gas pressure increases with temperature at constant volume.
- Avogadro's Law (1811): Amedeo Avogadro proposed that equal volumes of gases at the same temperature and pressure contain the same number of molecules.
These laws were eventually combined into one equation:
PV = nRT,
where P is pressure, V is volume, n is the number of moles, R is the gas constant, and T is temperature in kelvin.
This unified model-formulated in the 19th century-became known as the Ideal Gas Law, providing a simple, reliable way to describe gas behavior under a range of conditions.
Take These Quizzes
(73).jpg)
How Do These Laws Combine into the Ideal Gas Law?
The Ideal Gas Law unifies four fundamental gas laws into a single equation by connecting pressure (P), volume (V), temperature (T), and the amount of gas (n). Each individual law shows a specific relationship between two or more of these variables:
- Boyle's Law: P ∝ 1/V (when T and n are constant)
- Charles's Law: V ∝ T (when P and n are constant)
- Gay-Lussac's Law: P ∝ T (when V and n are constant)
- Avogadro's Law: V ∝ n (when P and T are constant)
Combining all these proportional relationships gives:
PV ∝ nT
To convert this proportionality into an equation, a constant R (the ideal gas constant) is introduced, leading to the Ideal Gas Law:
PV = nRT
This equation accurately models gas behavior under ideal conditions-low pressure and high temperature-and is widely used in both science and engineering.
Fig: Graphical Representation of the Three Gas Laws
How Does the Combined Gas Law Work?
The Combined Gas Law merges Boyle's, Charles's, and Gay-Lussac's Laws into one equation to describe the relationship between pressure (P), volume (V), and temperature (T) when the amount of gas (n) is constant. It allows us to calculate how a gas will behave when two or more variables change at once.
The formula is:
(P₁ × V₁) / T₁ = (P₂ × V₂) / T₂
Where:
- P₁, V₁, T₁ are the initial pressure, volume, and temperature
- P₂, V₂, T₂ are the final pressure, volume, and temperature
- Temperature must always be in kelvin (K)
This equation helps predict how a gas sample reacts when conditions shift. For example, if a gas is compressed (volume decreases) and heated (temperature increases), the pressure might stay the same or increase, depending on the exact values. By using the Combined Gas Law, you can solve for any one unknown when the other five values are known.
What Are the Properties of Gases in Relation to the Gas Laws?
The behavior of gases is governed by four key properties, each directly related to one or more gas laws. Understanding these helps explain how gases respond to changes in their environment:
Pressure (P)
Pressure is the force exerted by gas molecules when they collide with the walls of their container. It's measured in units like atm, Pa, or mmHg.
- Boyle's Law shows that pressure is inversely proportional to volume when temperature is constant (P ∝ 1/V).
- Gay-Lussac's Law shows that pressure is directly proportional to temperature at constant volume (P ∝ T).
Volume (V)
Volume is the amount of space the gas occupies.
- Boyle's Law: Volume decreases as pressure increases (at constant temperature).
- Charles's Law: Volume increases as temperature increases (at constant pressure).
- Avogadro's Law: Volume is directly proportional to the number of gas molecules (V ∝ n).
Temperature (T)
Temperature measures the average kinetic energy of gas particles and must be expressed in kelvin (K) when applying gas laws.
- Higher temperature = faster molecules = higher pressure or volume depending on constraints.
- Appears in all gas laws (Boyle's, Charles's, Gay-Lussac's, and Ideal Gas Law).
Amount of Gas (n)
Measured in moles, this refers to how many particles are present.
- Avogadro's Law: More moles = greater volume (at constant temperature and pressure).
- The Ideal Gas Law includes this in the equation PV = nRT, linking it to all other properties.
Each of these properties is interconnected. Changing one affects the others, and gas laws provide the mathematical tools to predict those effects.
Take These Quizzes
How Are Moles of Gas Calculated Using the Gas Laws?
To calculate the moles of gas (n), you use the Ideal Gas Law:
PV = nRT
Where:
- P = pressure (in atm)
- V = volume (in liters)
- n = number of moles
- R = ideal gas constant (0.0821 L·atm/mol·K)
- T = temperature (in kelvin)
Rearranging the formula to solve for n:
n = PV / RT
This equation allows you to determine how many moles of gas are present if you know the gas's pressure, volume, and temperature. It's especially useful in chemistry for:
- Predicting the amount of gas produced or consumed in a reaction
- Verifying chemical equations through stoichiometry
- Determining molar mass when mass and conditions are known
Example:
If a gas has a pressure of 1.5 atm, volume of 3.0 L, and temperature of 300 K:
n = (1.5 × 3.0) / (0.0821 × 300) ≈ 0.183 moles
This approach provides a practical, accurate way to quantify gas in both laboratory and industrial settings.
Conclusion
In this lesson, we explored the Ideal Gas Law and its underlying gas laws-Boyle's Law, Charles's Law, Avogadro's Law, and Gay-Lussac's Law-that explain how gases behave under varying pressure, volume, and temperature conditions. We traced the historical development of these laws, examined their mathematical relationships, and discussed their practical applications and limitations. Understanding the Combined Gas Law further demonstrated how these principles are integrated to solve gas-related problems.
Rate this lesson:
Back to top