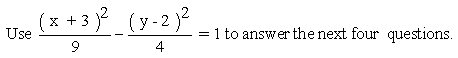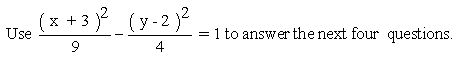Trivia Quiz On Hyperbolas 10.4
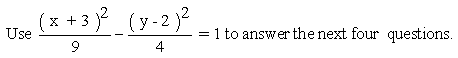
; &nbs p; &nb sp; &n bsp; & nbsp;
Questions and Answers
- 1.
A hyperbola is the conic that has __
- A.
Asymptotes
- B.
Minor axis
- C.
1 focus
Correct Answer
A. AsymptotesExplanation
A hyperbola is a conic section that has asymptotes. Asymptotes are lines that the hyperbola approaches but never intersects. They are used to define the shape and orientation of the hyperbola. The presence of asymptotes distinguishes a hyperbola from other conic sections like ellipses and parabolas. A hyperbola also has a major axis, which is the line segment connecting the vertices, and two foci. However, the number of foci is not specified in the question, so it is not included in the explanation.Rate this question:
-
- 2.
X^2 / 4 - y^2 / 19 = 1 has a major axis length of __?
Correct Answer
2Explanation
The equation x^2 / 4 - y^2 / 19 = 1 represents a hyperbola. The major axis of a hyperbola is the longer axis, which is determined by the term with the larger coefficient. In this case, the term with the larger coefficient is x^2 / 4, so the major axis length is 2.Rate this question:
- 3.
Y^2 / 9 - x^2 / 16 = 1 has foci at __?
Correct Answer
(0,+/-5)
(0,5) (0,-5)Explanation
The equation y^2 / 9 - x^2 / 16 = 1 represents a hyperbola with its center at the origin (0,0). The foci of a hyperbola are located along the transverse axis, which is the y-axis in this case. The distance from the center to each focus is given by c, where c^2 = a^2 + b^2, and a and b are the lengths of the conjugate and transverse axes, respectively. In this equation, a = 3 and b = 4. Plugging these values into the equation, we get c^2 = 3^2 + 4^2 = 9 + 16 = 25. Therefore, c = 5. Since the foci are located along the y-axis, the coordinates of the foci are (0,5) and (0,-5).Rate this question:
- 4.
The center is at?
Correct Answer
(-3,2)Explanation
The answer (-3,2) indicates that the center of the given object, which is not specified in the question, is located at the coordinates (-3,2).Rate this question:
- 5.
The vertices are?
Correct Answer
(0,2)(-6,2)
(0,2) (-6,2)Explanation
The given answer states that the vertices are (0,2)(-6,2) and (0,2)(-6,2). This means that there are two sets of coordinates given, (0,2) and (-6,2), and both sets are repeated.Rate this question:
- 6.
The length of the major axis is?
- A.
3
- B.
6
- C.
9
- D.
12
Correct Answer
B. 6Explanation
The length of the major axis refers to the longest diameter of an ellipse. In this case, the given options represent different lengths for the major axis. Among these options, the correct answer is 6, as it represents the length of the major axis.Rate this question:
-
Quiz Review Timeline +
Our quizzes are rigorously reviewed, monitored and continuously updated by our expert board to maintain accuracy, relevance, and timeliness.
-
Current Version
-
Mar 21, 2023Quiz Edited by
ProProfs Editorial Team -
Apr 19, 2010Quiz Created by
Salgm
 Back to top
Back to top