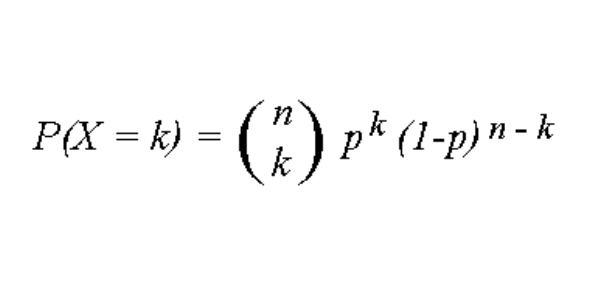In binomial probability assessment, which of the following conditions must be met?

The outcomes of each trial must be independent.

The probability of success must be constant for each trial.

There must be a fixed number of trials.

All of the following are binomial experiments except:

Rolling a die until a 6 appears

Asking 200 people if they have a car

Rolling a die to see if a 5 appears

Binomial Probability Assessment Test Quiz

1.

How many possible outcome(s) has a Binomial probability?
- A.
  
  1
- B.
  
  2
- C.
  
  3
- D.
  
  4
Correct Answer
B. 2

Explanation
A Binomial probability has two possible outcomes: success or failure. It is a discrete probability distribution that models the number of successes in a fixed number of independent Bernoulli trials, where each trial has the same probability of success. Therefore, the correct answer is 2.

Rate this question:
2.

In an experiment, a tossed coin has how many outcomes?
- A.
  
  4
- B.
  
  3
- C.
  
  2
- D.
  
  1
Correct Answer
C. 2

Explanation
When a coin is tossed, there are two possible outcomes: the coin landing on its heads side or the coin landing on its tails side. These outcomes are mutually exclusive, meaning that only one of them can occur at a time. The outcome of a coin toss is random and depends on various factors, such as the force and angle of the toss, the height from which the coin is dropped, and air resistance. Despite these factors, the probability of getting either heads or tails in a fair coin toss is equal, which is 1/2 or 50% for each outcome. It is important to note that the coin does not have a memory of previous tosses, meaning that the outcome of one coin toss does not affect the outcome of any future tosses. Each toss is an independent event with the same probabilities for heads and tails.

Rate this question:
3.

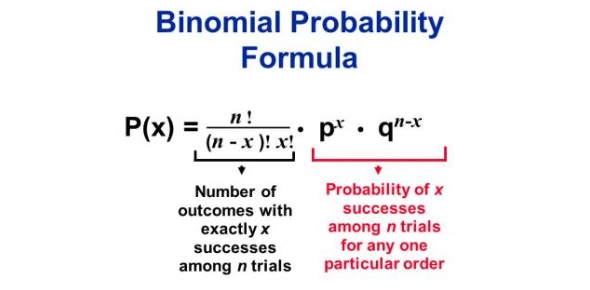
The first variable n in a Binomial experiment shows the _____ the experiment is performed.
- A.
  
  Number of times
- B.
  
  Range
- C.
  
  Variety
- D.
  
  None of the above
Correct Answer
A. Number of times

Explanation
The first variable 'n' in a binomial experiment represents the number of times the experiment is performed, which is also referred to as the number of trials or the sample size. In a binomial experiment, there are two possible outcomes (success or failure), and the experiment is repeated a fixed number of times (n). The binomial probability distribution helps to calculate the probability of achieving a specific number of successes (k) in n trials.

Rate this question:
4.

In Binomial probability experiments, the number of trials must be fixed.
- A.
  
  True
- B.
  
  False
- C.
  
  Undefined
- D.
  
  None of the above
Correct Answer
A. True

Explanation
In binomial probability experiments, the number of trials must be fixed because the binomial distribution is based on a fixed number of independent trials, each with only two possible outcomes (success or failure). The probability of success and failure remains constant for each trial, and the number of trials determines the shape and characteristics of the binomial distribution. Therefore, it is necessary to have a fixed number of trials in order to accurately calculate and analyze the probabilities in a binomial experiment.

Rate this question:
5.

In Binomial Distribution, each trial must be independent.
- A.
  
  True
- B.
  
  False
- C.
  
  Not defined
- D.
  
  None of the above
Correct Answer
A. True

Explanation
In Binomial Distribution, each trial must be independent. This means that the outcome of one trial does not affect the outcome of any other trial. Each trial is considered to be separate and unrelated to the others. This is a fundamental assumption in the binomial distribution, as it allows for the calculation of probabilities based on the number of successes and failures in a fixed number of independent trials.

Rate this question:
6.

In binomial probability assessment, which of the following conditions must be met?
- A.
  
  The outcomes of each trial must be independent.
- B.
  
  The probability of success must be constant for each trial.
- C.
  
  There must be a fixed number of trials.
- D.
  
  All of the above.
Correct Answer
D. All of the above.

Explanation
Binomial probability assessment involves scenarios with fixed trials, each having independent outcomes and a constant probability of success. It's used to calculate the likelihood of obtaining a specific number of successes in a series of trials, providing a statistical framework for various real-world applications, such as quality control and hypothesis testing.

Rate this question:

0
0
7.

In a traffic light consisting of red, yellow and green. What is the probability of getting 2 reds?
- A.
  
  0.343
- B.
  
  0.189
- C.
  
  0.441
- D.
  
  0.027
Correct Answer
B. 0.189

Explanation
The probability of getting 2 reds can be calculated by multiplying the probability of getting a red light on the first try (which is 1/3) with the probability of getting another red light on the second try (which is 1/3). Therefore, the probability of getting 2 reds is (1/3) * (1/3) = 1/9 = 0.111. Since none of the given options match this probability, the correct answer is not available.

Rate this question:

1
0
8.

A coin is tossed 10 times. What is the probability of getting exactly 6 heads?
- A.
  
  2
- B.
  
  0.5373457885
- C.
  
  0.55433
- D.
  
  0.205078125
Correct Answer
D. 0.205078125
9.

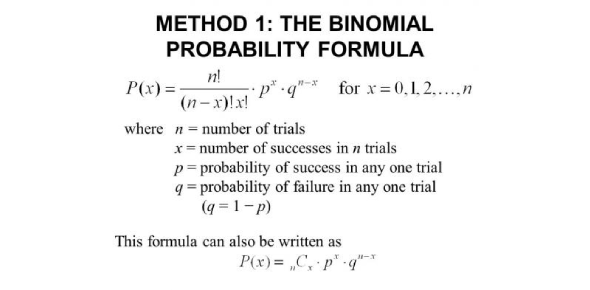
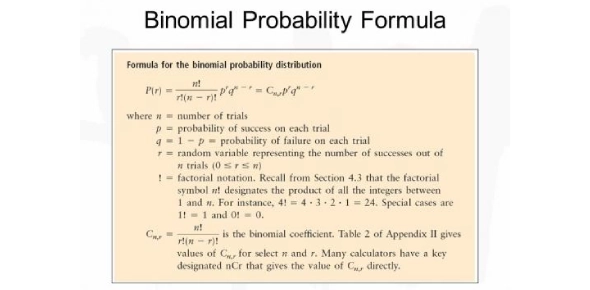
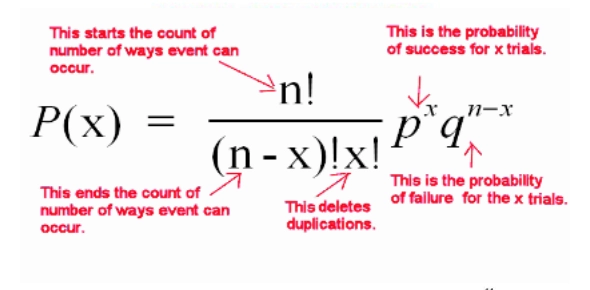
The notation n! stands for:
- A.
  
  N-numerical
- B.
  
  N-optional
- C.
  
  N-exclamation
- D.
  
  N-factorial
Correct Answer
D. N-factorial

Explanation
The notation n! stands for n-factorial. Factorial is a mathematical operation that represents the product of all positive integers from 1 to n. It is denoted by placing an exclamation mark after the number. For example, 5! is equal to 5 x 4 x 3 x 2 x 1, which equals 120.

Rate this question:
10.

All of the following are binomial experiments except:
- A.
  
  Tossing a coin
- B.
  
  Asking 200 people if they have a car
- C.
  
  Rolling a die until a 6 appears
- D.
  
  Rolling a die to see if a 5 appears
Correct Answer
C. Rolling a die until a 6 appears

Explanation
A binomial experiment is one that consists of a fixed number of independent trials, each with two possible outcomes (success or failure), and a constant probability of success. Tossing a coin, asking 200 people if they have a car, and rolling a die to see if a 5 appears all meet these criteria. However, rolling a die until a 6 appears does not have a fixed number of trials, as the number of rolls can vary. Therefore, it is not a binomial experiment.

Rate this question: