Series Convergence & Estimation Assessment Test
-
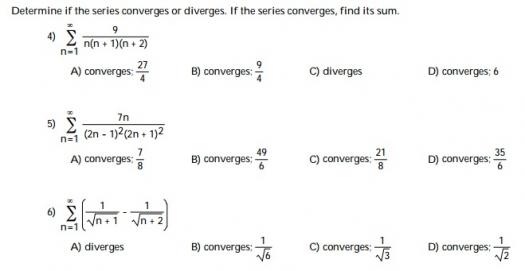
A series that diverges means the partial sums have no...
-
Standard
-
Function
-
Approach infinity
-
Line
-
Take our intelligent assessment test to evaluate your knowledge of how series converges and diverges, and the value a series converges to — called series convergence and estimation.

Quiz Preview
- 2.
A series that converges has a number that is...
-
Approached
-
Fine
-
Done
-
Over
Correct Answer
A. ApproachedExplanation
The correct answer is "Approached" because when a series converges, it means that the terms of the series get closer and closer to a certain number as the series progresses. This number is called the limit of the series. So, the terms of the series "approach" the limit value as the series goes on. Therefore, "Approached" is the appropriate word to describe the behavior of a number in a converging series.Rate this question:
-
- 3.
A sequence which does not converge is said to be...
-
Open
-
Divergent
-
Over
-
Broken
Correct Answer
A. DivergentExplanation
A sequence which does not converge is said to be divergent. This means that the terms in the sequence do not approach a specific value or limit as the sequence progresses. Instead, the terms may fluctuate or diverge in different directions, indicating that the sequence does not have a well-defined limit.Rate this question:
-
- 4.
A series can be defined as...
-
Addition
-
Collection of numbers
-
Line of figures
-
Sequence of sums
Correct Answer
A. Sequence of sumsExplanation
A series can be defined as a sequence of sums, where each term is obtained by adding the previous terms in the sequence. It involves continuously adding numbers in a specific order, resulting in a sum at each step. This definition highlights the primary characteristic of a series, which distinguishes it from other options such as addition, collection of numbers, or line of figures. A series focuses on the cumulative sum of terms, rather than individual numbers or a visual representation.Rate this question:
-
- 5.
A series that converges has a...
-
Finite limit
-
Function
-
Slope
-
Integral
Correct Answer
A. Finite limitExplanation
A series that converges means that the sum of its terms approaches a specific value as the number of terms increases. This specific value is called the limit of the series. In this case, the correct answer is "Finite limit" because a convergent series has a limit that is a finite number, meaning it is not infinite or undefined.Rate this question:
-
- 6.
The fundamental notion on which the whole of analysis ultimately rests is the...
-
Function of a sequence
-
Nomination
-
Limit of a sequence
-
Or donation
Correct Answer
A. Limit of a sequenceExplanation
The correct answer is "Limit of a sequence". In analysis, the concept of limit of a sequence is fundamental. It refers to the value that a sequence approaches as the terms of the sequence get closer and closer to a certain value. The limit of a sequence helps in understanding the behavior and properties of the sequence, and it is crucial in various areas of mathematics, such as calculus and real analysis.Rate this question:
-
- 7.
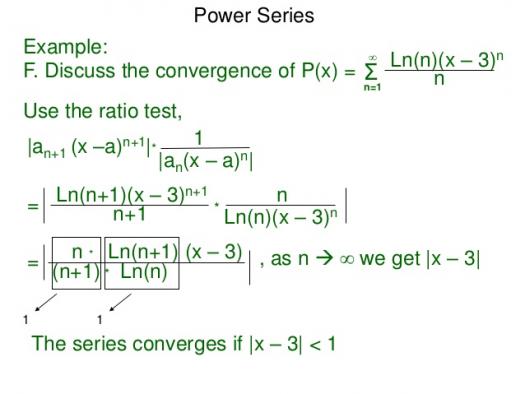
The set of values for which a series converges is the...
-
Positioning
-
Power rule
-
Limiting power
-
Interval of convergence
Correct Answer
A. Interval of convergenceExplanation
The interval of convergence refers to the range of values for which a series converges. It represents the set of all input values that will result in a convergent series. This interval can be determined using various methods such as the power rule or limiting power. Therefore, the correct answer is "Interval of convergence."Rate this question:
-
- 8.
A fundamental concept in calculus and analysis concerning the behavior of that function near a particular input is...
-
Power
-
Limit of a function
-
Theorem
-
Input theory
Correct Answer
A. Limit of a functionExplanation
The correct answer is "Limit of a function." In calculus and analysis, the concept of the limit of a function refers to the behavior of the function as it approaches a specific input value. It helps determine if the function approaches a certain value or becomes infinite as the input approaches a given value. This concept is crucial in understanding the continuity, differentiability, and overall behavior of functions in calculus and analysis.Rate this question:
-
- 9.
A limit converges if it has a...
-
Finite value
-
Number
-
Figure
-
Value
Correct Answer
A. Finite valueExplanation
A limit converges if it has a finite value. This means that as the input approaches a certain value, the output of the function approaches a specific number, rather than becoming infinitely large or undefined. In other words, the function "settles down" and approaches a fixed value as the input gets closer and closer to a certain point.Rate this question:
-
- 10.
An interval in which a power series converges is called the...
-
Radius of convergence
-
Limit line
-
Number line
-
Limitation
Correct Answer
A. Radius of convergenceExplanation
The correct answer is "Radius of convergence". This term refers to the interval in which a power series converges. It represents the distance from the center of the power series to the nearest point where the series converges. The radius of convergence determines the range of values for which the power series provides an accurate approximation of the function it represents.Rate this question:
-
Quiz Review Timeline (Updated): Mar 20, 2023 +
Our quizzes are rigorously reviewed, monitored and continuously updated by our expert board to maintain accuracy, relevance, and timeliness.
-
Current Version
-
Mar 20, 2023Quiz Edited by
ProProfs Editorial Team -
Dec 27, 2017Quiz Created by
Cripstwick
Converging And Diverging Series
Explore the behavior of series in mathematics through this quiz on converging and diverging series. Determine convergence, divergence, and conditional convergence of various...
Questions:
7 |
Attempts:
300 |
Last updated:
Mar 22, 2023
|
Calculus Constants: Kronecker Delta Quiz
Welcome to the Calculus Constants: Kronecker Delta Quiz! Test your knowledge of this fundamental concept in mathematics through a series of thought-provoking questions. The...
Questions:
10 |
Attempts:
93 |
Last updated:
Nov 25, 2023
|
Integration Methods Quiz: Calculus Mathematics
Have you studied Calculus mathematics? If yes, you can play this Integration Methods Quiz to check your knowledge of various methods of Integration. You can get the perfect...
Questions:
10 |
Attempts:
1788 |
Last updated:
Mar 22, 2023
|
Instantaneous Rate Of Change
Can you interpret and/or estimate instantaneous rate of change in multiple representations?
Questions:
5 |
Attempts:
703 |
Last updated:
Mar 21, 2023
|
LIMITS AND DERIVATIVES CLASS 11
This quiz is designed for Class 11 students to assess their understanding of limits and derivatives, key concepts in calculus.
Questions:
10 |
Attempts:
601 |
Last updated:
Dec 30, 2024
|
Chapter 12 : Limits And Derivatives
This quiz titled 'Chapter 12: Limits and Derivatives' evaluates understanding of calculus concepts such as product rule, quotient rule, power rule, and the basics of derivatives...
Questions:
10 |
Attempts:
176 |
Last updated:
Dec 30, 2024
|
 Back to top
Back to top