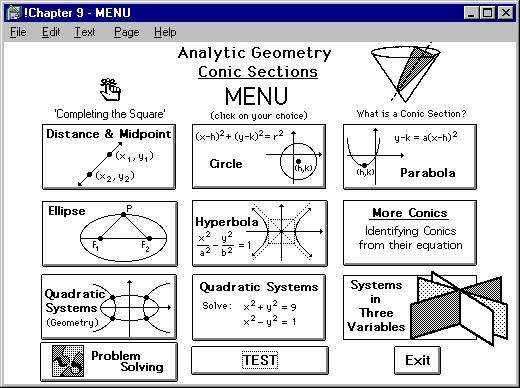
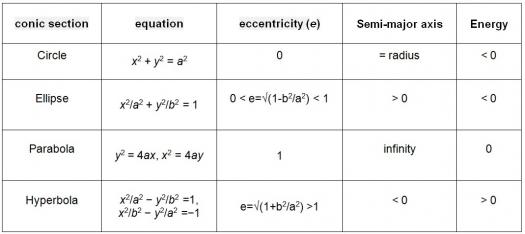
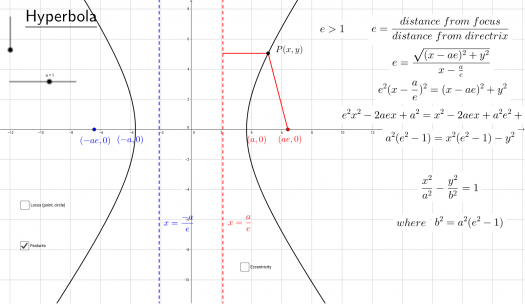
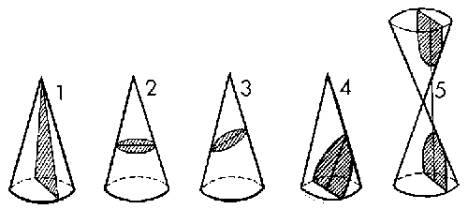
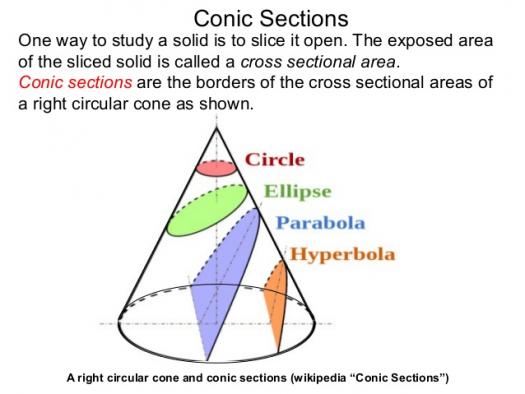
What Do You Know About Conic Sections?

2.

The conic sections have been studied by the ancient .....

Greek mathematician
Latin mathematician
Russian mathematician
British mathematician

Correct Answer

A. Greek mathematician

Explanation

The conic sections, which include the circle, ellipse, parabola, and hyperbola, were extensively studied by ancient Greek mathematicians. They made significant contributions to the understanding and properties of these curves, with prominent mathematicians such as Euclid, Apollonius of Perga, and Archimedes delving into the subject. Their work laid the foundation for the development of conic section theory and its applications in various fields of mathematics and science.

Rate this question:

3.

The conic sections have been studied by the ancient Greek mathematicians with this work culminating around

100 B.C
200 B.C
300 B.C
Option 4

Correct Answer

A. 200 B.C

Explanation

The conic sections were extensively studied by ancient Greek mathematicians, and their work on this subject reached its peak around 200 B.C. During this time, mathematicians such as Euclid and Apollonius made significant contributions to the understanding and classification of conic sections, including the properties of ellipses, parabolas, and hyperbolas. Their work laid the foundation for further advancements in geometry and calculus.

Rate this question:

4.

The greatest progress in the study of conics by the ancient Greeks is due to ....

Euclid
Apollonius of Perga
Menaechmus
Archimedes

Correct Answer

A. Apollonius of Perga

Explanation

Apollonius of Perga is credited with making the greatest progress in the study of conics by the ancient Greeks. He is known for his work "Conics," in which he extensively studied and classified different types of conic sections, including the ellipse, parabola, and hyperbola. Apollonius' work laid the foundation for the modern understanding of conic sections and their properties. His contributions greatly advanced the field of mathematics and had a lasting impact on the study of conics.

Rate this question:

5.

..... (died c. 350 CE) is credited with expounding on the importance of the concept of a conic's focus, and detailing the related concept of a directrix, including the case of the parabola (which is lacking in Apollonius's known works).

Archimedes
Euclid
Menaechmus
Pappus of Alexandr

Correct Answer

A. Pappus of Alexandr

Explanation

Pappus of Alexandr is credited with expounding on the importance of the concept of a conic's focus and detailing the related concept of a directrix, including the case of the parabola. This means that Pappus of Alexandr contributed to the understanding of the geometric properties of conic sections, specifically focusing on the focus and directrix of these curves. This knowledge was lacking in the known works of Apollonius, another important mathematician of the time. Therefore, Pappus of Alexandr is the correct answer as he made significant contributions to the study of conic sections.

Rate this question:

6.

An instrument for drawing conic sections was first described in ....

1000 CE
2000 CE
3000 CE
4000 CE

Correct Answer

A. 1000 CE

Explanation

The correct answer is 1000 CE. This means that an instrument for drawing conic sections was first described in the year 1000 CE.

Rate this question:

7.

An instrument for drawing conic sections was first described in 1000 CE by the Islamic mathematician ......

Muhammed
Al-Kuhi
Yusuf
Dawud

Correct Answer

A. Al-Kuhi

Explanation

Al-Kuhi, an Islamic mathematician, is credited with describing the instrument for drawing conic sections in 1000 CE. This instrument was a significant development in mathematics and allowed for the accurate construction of conic sections, which are important curves in geometry. Al-Kuhi's contribution in describing this instrument showcases the advancements made by Islamic mathematicians during the medieval period.

Rate this question:

8.

..... extended the theory of conics through the "principle of continuity", a precursor to the concept of limits.

Albert Einstein
Johannes Kepler
Isaac Newton
Bill Nye

Correct Answer

A. Johannes Kepler

Explanation

Johannes Kepler extended the theory of conics through the "principle of continuity", which is a precursor to the concept of limits. This means that Kepler built upon the existing understanding of conics and developed the idea of continuity, which eventually led to the concept of limits. Although Albert Einstein, Isaac Newton, and Bill Nye are all notable figures in their respective fields, they did not specifically contribute to the extension of the theory of conics through the principle of continuity.

Rate this question:

9.

Kepler first used the term foci in ....

1604
1514
1918
2008

Correct Answer

A. 1604

10.

Mathematics is usually studied in the ....

School
Church
Mosque
Museum

Correct Answer

A. School

Explanation

Mathematics is usually studied in school because it is a fundamental subject taught in educational institutions. Schools provide a structured environment with qualified teachers who can teach and guide students in understanding mathematical concepts and solving problems. Additionally, schools have resources such as textbooks, classrooms, and interactive tools that facilitate the learning and practice of mathematics. Therefore, it is the most appropriate and common place for students to study mathematics.

Rate this question:

What Do You Know About Conic Sections?

There are how many types of conic section?

Quiz Preview

The conic sections have been studied by the ancient .....

The conic sections have been studied by the ancient Greek mathematicians with this work culminating around

The greatest progress in the study of conics by the ancient Greeks is due to ....

..... (died c. 350 CE) is credited with expounding on the importance of the concept of a conic's focus, and detailing the related concept of a directrix, including the case of the parabola (which is lacking in Apollonius's known works).

An instrument for drawing conic sections was first described in ....

An instrument for drawing conic sections was first described in 1000 CE by the Islamic mathematician ......

..... extended the theory of conics through the "principle of continuity", a precursor to the concept of limits.

Kepler first used the term foci in ....

Mathematics is usually studied in the ....