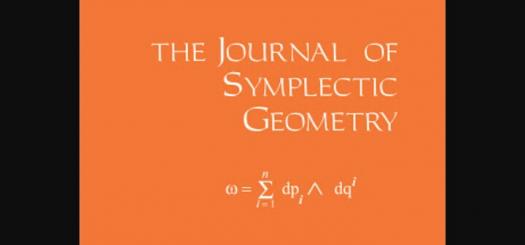
What Do You Know About Symplectic Representations?
-
What condition will a representation of a group G preserve ω?
-
W(w.v,gw)=w(v,w)
-
W(g.v,gw)=w(v,w)
-
W(g.v,vw)=w(v,w)
-
W(g.v,gw)=w(v,g)
-
In the representation theory branch of mathematics, Symplectic representations are a Lie variable-based math on a symplectic vector space or a representation of a gathering which portrays a form or frame that is symplectic.
If you would like to know more about this widespread type of representations, let's asses your knowledge of Symplectic representations.

- 2.
Which indicator is used to identify Symplectic representations?
-
Frobenius-schur indicator
-
Thomas indicator
-
Abel–Jacobi theorem
-
Ado's theorem
Correct Answer
A. Frobenius-schur indicatorExplanation
The Frobenius-Schur indicator is used to identify Symplectic representations. It is a mathematical concept that helps determine the type of representation of a group, specifically whether it is self-dual or not. The indicator takes values of 0, 1, or -1, and a value of 1 indicates a symplectic representation. This indicator is important in the study of representation theory and has applications in various areas of mathematics and physics.Rate this question:
-
- 3.
Which other representations indicate Symplectic representations?
-
Quaternionic representations of indefinite groups
-
Thomas representations
-
Quaternionic representations of finite groups
-
Mathematical folding theorem
Correct Answer
A. Quaternionic representations of finite groupsExplanation
Quaternionic representations of finite groups indicate Symplectic representations. This is because quaternionic representations are a special type of representation in which the group elements are represented by quaternion matrices. When these quaternionic representations are applied to finite groups, they can be used to describe and analyze the symmetries and transformations of these groups. Therefore, quaternionic representations of finite groups are a valid indication of Symplectic representations.Rate this question:
-
- 4.
When effective or faithful representation is a representation (V , φ), what is the homomorphism φ?
-
Injective
-
Objective
-
Functional
-
Perpendicular
Correct Answer
A. InjectiveExplanation
When effective or faithful representation is a representation (V, φ), the homomorphism φ is injective. This means that for every element in the domain of φ, there is a unique element in the codomain that it maps to. In other words, no two distinct elements in the domain are mapped to the same element in the codomain. This ensures that the representation is one-to-one and preserves the structure of the original set.Rate this question:
-
- 5.
When V and W are vector spaces over F, equipped with representations φ and ψ of a group G, then what is an equivariant map from V to W?
-
Vertical map
-
Linear map
-
Functional map
-
Horizontal map
Correct Answer
A. Linear mapExplanation
An equivariant map from V to W is a linear map that preserves the action of the group G. In other words, for any g in G and v in V, the equivariant map satisfies φ(g)v = ψ(g)F(v), where F is the linear map from V to W. Therefore, the correct answer is "Linear map" because it captures the essential property of preserving the group action.Rate this question:
-
- 6.
When α is said to be invertible, what is this called?
-
Functional point
-
Repression
-
Isomorphism
-
Dual movement
Correct Answer
A. IsomorphismExplanation
When α is said to be invertible, it means that there exists an inverse of α, denoted as α^(-1), such that the composition of α and α^(-1) gives the identity element. This property is called isomorphism. Isomorphism is a concept in mathematics that refers to a structure-preserving mapping between two mathematical objects, where the objects have the same structure and can be considered equivalent in some sense. In this case, α being invertible implies that it has a corresponding inverse that preserves the structure of the original object.Rate this question:
-
- 7.
What does the definition of an irreducible representation simple imply?
-
Dual movement
-
Schur's lemma
-
Division algebra
-
Endomorphisms
Correct Answer
A. Schur's lemmaExplanation
The definition of an irreducible representation simple implies Schur's lemma. Schur's lemma states that if a linear operator commutes with all operators in a given irreducible representation, then it must be a scalar multiple of the identity operator. In other words, if an irreducible representation is simple, any operator that commutes with it must be a scalar multiple of the identity. This is a fundamental result in representation theory and is closely related to the concept of irreducibility.Rate this question:
-
- 8.
When a representation is the direct sum of two proper nontrivial subrepresentations, what is it said to be?
-
Repressed
-
Dimensional
-
Decomposable
-
Rational
Correct Answer
A. DecomposableExplanation
When a representation is the direct sum of two proper nontrivial subrepresentations, it is said to be decomposable.Rate this question:
-
- 9.
What do we call the situation when the duality between the circle group S 1 and the integer Z? The analysis of the...
-
Theory of Fourier series
-
Carter theory
-
Birkhoff's theorem
-
Cartan–Dieudonné theorem
Correct Answer
A. Theory of Fourier seriesExplanation
The duality between the circle group S1 and the integer Z is referred to as the theory of Fourier series. This theory explores the relationship between periodic functions and their Fourier series representations, which involve decomposing a function into a sum of sinusoidal components. The duality between S1 and Z arises from the fact that the group of integers Z can be thought of as the set of frequencies that make up the Fourier series representation of a periodic function on the unit circle.Rate this question:
-
- 10.
Which symbol represents Symplectic vector space?
-
(V,W)
-
(V,U)
-
(W, V)
-
(S, U)
Correct Answer
A. (V,W)Explanation
The symbol (V,W) represents a symplectic vector space. The convention in mathematics is to use parentheses to denote an ordered pair or tuple, and in this case, it represents a pair of vector spaces V and W. In symplectic geometry, a symplectic vector space is a vector space equipped with a nondegenerate, skew-symmetric bilinear form called a symplectic form. Therefore, the answer (V,W) is the correct representation for a symplectic vector space.Rate this question:
-
Quiz Review Timeline (Updated): Mar 19, 2023 +
Our quizzes are rigorously reviewed, monitored and continuously updated by our expert board to maintain accuracy, relevance, and timeliness.
-
Current Version
-
Mar 19, 2023Quiz Edited by
ProProfs Editorial Team -
May 25, 2018Quiz Created by
AdeKoju
 Back to top
Back to top