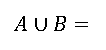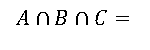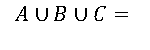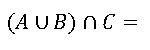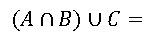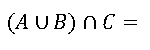Lhs 4.1c Statistics - Fundamentals Of Probability
-
If a marble is randomly chosen from a bag that contains exactly 8 red marbles, 6 blue marbles, and 6 white marbles, what is the probability that the marble will NOT be BLUE?
-
3/4
-
3/5
-
3/10
-
7/10
-
This quiz, titled 'LHS 4.1c Statistics - Fundamentals of Probability', assesses understanding of basic probability concepts using real-world scenarios involving marbles of different colors. It tests the ability to calculate probabilities of specific outcomes and complements statistical learning.
(128).webp "Lhs 4.1c Statistics - Fundamentals Of Probability - Quiz")
Quiz Preview
- 2.
If a marble is randomly chosen from a bag that contains exactly 8 red marbles, 6 blue marbles, and 6 white marbles, what is the probability that the marble will NOT be RED?
-
2/5
-
7/10
-
3/5
-
14/20
Correct Answer
A. 3/5Explanation
The probability of an event happening is calculated by dividing the number of favorable outcomes by the total number of possible outcomes. In this case, the total number of marbles is 8+6+6=20. The number of favorable outcomes (marbles that are not red) is 6+6=12. Therefore, the probability of choosing a marble that is not red is 12/20, which simplifies to 3/5.Rate this question:
-
- 3.
If a marble is randomly chosen from a bag that contains exactly 8 red marbles, 6 blue marbles, and 6 white marbles, what is the probability that the marble will NOT be WHITE?
-
7/10
-
2/5
-
6/20
-
3/10
Correct Answer
A. 7/10Explanation
The probability of selecting a marble that is not white can be calculated by dividing the number of non-white marbles (8 red marbles + 6 blue marbles = 14) by the total number of marbles (8 red marbles + 6 blue marbles + 6 white marbles = 20). Therefore, the probability is 14/20, which simplifies to 7/10.Rate this question:
-
- 4.
A jar contains 4 green marbles, 5 red marbles, and 11 white marbles. If one marble is chosen at random, what is the probability that it will be GREEN?
-
1/3
-
1/4
-
1/5
-
1/16
Correct Answer
A. 1/5Explanation
The probability of choosing a green marble can be calculated by dividing the number of green marbles (4) by the total number of marbles (4 + 5 + 11 = 20). Therefore, the probability is 4/20, which simplifies to 1/5.Rate this question:
-
- 5.
A jar contains 4 green marbles, 5 red marbles, and 11 white marbles. If one marble is chosen at random, what is the probability that it will be RED?
-
1/3
-
1/4
-
1/5
-
1/6
Correct Answer
A. 1/4Explanation
The probability of choosing a red marble can be calculated by dividing the number of red marbles by the total number of marbles in the jar. In this case, there are 5 red marbles out of a total of 20 marbles (4 green + 5 red + 11 white). Therefore, the probability of choosing a red marble is 5/20, which simplifies to 1/4.Rate this question:
-
- 6.
A jar contains 4 green marbles, 5 red marbles, and 11 white marbles. If one marble is chosen at random, what is the probability that it will be WHITE?
-
1/4
-
1/5
-
11/20
-
1/3
Correct Answer
A. 11/20Explanation
The probability of choosing a white marble can be calculated by dividing the number of white marbles (11) by the total number of marbles in the jar (4 + 5 + 11 = 20). Therefore, the probability is 11/20.Rate this question:
-
- 7.
A number from the set {1, 2, 3, …, 20} is selected at random. What is the probability that the number is ODD and less than 15?
-
8/15
-
2/5
-
7/20
-
7/15
Correct Answer
A. 7/20Explanation
The probability of selecting an odd number from the set {1, 2, 3, ..., 20} is 10/20, since there are 10 odd numbers in the set. The probability of selecting a number less than 15 is 14/20, since there are 14 numbers in the set that are less than 15. To find the probability of both events occurring, we multiply the probabilities together: (10/20) * (14/20) = 140/400 = 7/20. Therefore, the correct answer is 7/20.Rate this question:
-
- 8.
A= {2, 4, 6, 8, 10}B= {6, 8, 10, 12, 14}
-
{6, 7, 8, 9, 10}
-
{6, 8, 10}
-
{2, 4, 6, 8, 10, 12}
-
Empty set
Correct Answer
A. {6, 8, 10}Explanation
The correct answer is {6, 8, 10} because it is the intersection of set A and set B. The intersection of two sets is the set of elements that are common to both sets. In this case, the numbers 6, 8, and 10 are present in both set A and set B, so they are the only elements in the intersection.Rate this question:
-
- 9.
A= {2, 4, 6, 8, 10}B= {6, 8, 10, 12, 14}
-
{2, 4, 6, 8, 10, 12, 14}
-
{6, 8, 10}
-
{2, 6, 8, 10, 12, 14}
-
Empty set
Correct Answer
A. {2, 4, 6, 8, 10, 12, 14}Explanation
The answer {2, 4, 6, 8, 10, 12, 14} is the union of sets A and B. The union of two sets is a set that contains all the elements from both sets, without any duplicates. In this case, both sets A and B have some common elements (6, 8, 10), but the union includes all the elements from both sets, including the unique elements from each set. Therefore, the answer is the set that combines all the elements from sets A and B.Rate this question:
-
- 10.
A = {1, 2, 3, 4, 5}B = {2, 3, 4, 5, 6, 7}C= {5, 6, 7, 8, 9, 10}
-
{2, 3, 4, 5}
-
{5, 6}
-
{5}
-
Empty set
Correct Answer
A. {5}Explanation
The correct answer is {5} because it is the only element that is present in all three sets A, B, and C. The rest of the elements are either present in only one or two sets, or not present in any of the sets.Rate this question:
-
- 11.
A = {1, 2, 3, 4, 5}B = {2, 3, 4, 5, 6, 7}C= {5, 6, 7, 8, 9, 10}
-
{1, 2, 3, 4, 5, 6, 7, 8, 9, 10, 11}
-
{5}
-
{1, 2, 3, 4, 5, 6, 7, 8, 9, 10}
-
Empty set
Correct Answer
A. {1, 2, 3, 4, 5, 6, 7, 8, 9, 10}Explanation
The correct answer is {1, 2, 3, 4, 5, 6, 7, 8, 9, 10} because it is the union of sets A, B, and C. The union of sets includes all the elements from each set, without any duplicates. Therefore, the union of sets A, B, and C will include all the numbers from 1 to 10.Rate this question:
-
- 12.
A = {1, 2, 3, 4, 5}B = {2, 3, 4, 5, 6, 7}C= {5, 6, 7, 8, 9, 10}
-
{1, 2, 3, 4, 5, 6, 7}
-
{5, 6, 7}
-
{7, 8, 9}
-
Empty set
Correct Answer
A. {5, 6, 7}Explanation
The correct answer is {5, 6, 7} because it is the intersection of sets B and C. The intersection of two sets includes only the elements that are common to both sets. In this case, the elements 5, 6, and 7 are present in both sets B and C, so they are included in the intersection.Rate this question:
-
- 13.
A = {1, 2, 3, 4, 5}B = {2, 3, 4, 5, 6, 7}C= {5, 6, 7, 8, 9, 10}
-
{1, 2, 3, 4, 5}
-
{1, 2, 3, 4, 5, 6, 7, 8}
-
{2, 3, 4, 5, 6, 7, 8, 9, 10}
-
Empty set
Correct Answer
A. {2, 3, 4, 5, 6, 7, 8, 9, 10}Explanation
The correct answer is {2, 3, 4, 5, 6, 7, 8, 9, 10} because it is the union of sets B and C. The union of two sets includes all the elements that are present in either set, without any repetition. In this case, set B contains numbers from 2 to 7 and set C contains numbers from 5 to 10. The union of B and C will include all these numbers without repetition, resulting in the set {2, 3, 4, 5, 6, 7, 8, 9, 10}.Rate this question:
-
- 14.
A= {5, 10, 15, 20}B = {1, 2, 3, 4, 5}C= {25, 50, 75, 100}
-
{5, 10, 15, 20}
-
{1, 2, 3, 4, 5, 10, 15, 20}
-
{1, 2, 3, 4, 5, 10, 15, 20, 25, 50, 75, 100}
-
Empty set
Correct Answer
A. Empty setExplanation
The empty set is the correct answer because there is no common element between the sets A, B, and C. The sets A, B, and C do not have any elements in common, so the intersection of these sets is an empty set.Rate this question:
-
- 15.
7!=
-
5000
-
7
-
5040
-
10,040
Correct Answer
A. 5040Explanation
The answer 5040 is the correct factorial of 7. Factorial is the product of all positive integers from 1 to the given number. In this case, 7! = 7 x 6 x 5 x 4 x 3 x 2 x 1 = 5040.Rate this question:
-
- 16.
8!/6!=
-
40,320
-
56
-
720
-
0
Correct Answer
A. 56Explanation
The given expression is 8! divided by 6!. The factorial of a number is the product of all positive integers less than or equal to that number. Therefore, 8! is equal to 8 x 7 x 6 x 5 x 4 x 3 x 2 x 1, and 6! is equal to 6 x 5 x 4 x 3 x 2 x 1. When we divide 8! by 6!, the common factors cancel out, leaving us with 8 x 7, which equals 56.Rate this question:
-
- 17.
9! x 0!=
-
362,880
-
200,103
-
0
-
5000
Correct Answer
A. 362,880Explanation
The given expression is 9! x 0!. The factorial of a number is the product of all positive integers less than or equal to that number. The factorial of 9 (9!) is calculated as 9 x 8 x 7 x 6 x 5 x 4 x 3 x 2 x 1, which equals 362,880. The factorial of 0 (0!) is defined as 1. Therefore, when we multiply 9! by 0!, the result is 362,880.Rate this question:
-
- 18.
(7!)/(7-3)!=
-
300
-
410
-
5000
-
210
Correct Answer
A. 210Explanation
The given expression is (7!)/(7-3)! which simplifies to (7!)/4!. The factorial of 7 is 7x6x5x4x3x2x1 and the factorial of 4 is 4x3x2x1. When we cancel out the common terms in the numerator and denominator, we are left with 7x6x5 which equals 210. Therefore, the correct answer is 210.Rate this question:
-
Quiz Review Timeline (Updated): Jan 29, 2025 +
Our quizzes are rigorously reviewed, monitored and continuously updated by our expert board to maintain accuracy, relevance, and timeliness.
-
Current Version
-
Jan 29, 2025Quiz Edited by
ProProfs Editorial Team -
Dec 01, 2010Quiz Created by
Ichihana
Back to top