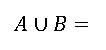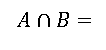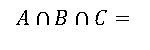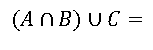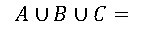Lhs 4.2 Statistics - Permutations And Combinations
LHS 4.2 Statistics - Permutations and Combinations
Questions and Answers
- 1.
There are 20 people who work in an office together. Four of these people are selected to go to the same conference together. How many such selections are possible?
- A.
116280
- B.
4845
- C.
80
- D.
Not enough information
Correct Answer
B. 4845Explanation
The question asks for the number of possible selections of four people from a group of 20 to go to a conference together. This is a combination problem, where order doesn't matter. The formula to calculate combinations is nCr = n! / (r!(n-r)!), where n is the total number of items and r is the number of items being selected. In this case, n = 20 and r = 4. Plugging these values into the formula, we get 20! / (4!(20-4)!) = 4845. Therefore, the correct answer is 4845.Rate this question:
-
- 2.
There are 20 people who work in an office together. Four of these people are selected to attend four different conferences. The first person selected will go to a conference in New York, the second will go to Chicago, the third to San Franciso, and the fourth to Miami. How many such selections are possible?
- A.
116280
- B.
4845
- C.
80
- D.
Not enough information
Correct Answer
A. 116280Explanation
The question asks for the number of possible selections of four people to attend four different conferences. Since the order of the conferences is specified (New York, Chicago, San Francisco, Miami), and each person can only attend one conference, we can use the concept of permutations to calculate the number of selections. The first person can be selected in 20 ways, the second person in 19 ways (as one person has already been selected), the third person in 18 ways, and the fourth person in 17 ways. Therefore, the total number of selections is 20 x 19 x 18 x 17 = 116280.Rate this question:
-
- 3.
Serial numbers for a product are to be made using three letters (using any letter of the alphabet) followed by two single-digit numbers. For example, JGR29 is one such serial number. How many such serial numbers are possible if neither letters nor numbers can be repeated?
- A.
117,000
- B.
15,690
- C.
2,000,000,000
- D.
1,404,000
Correct Answer
D. 1,404,000Explanation
The number of possible serial numbers can be calculated by multiplying the number of choices for each position. For the three letters, there are 26 choices for the first letter, 25 choices for the second letter (since it cannot be the same as the first), and 24 choices for the third letter (since it cannot be the same as the first or second). For the two single-digit numbers, there are 10 choices for each number (0-9). Therefore, the total number of possible serial numbers is 26 * 25 * 24 * 10 * 10 = 1,404,000.Rate this question:
-
- 4.
A 7-card hand is chosen from a standard 52-card deck. How many of these will have four spades and three hearts (remember that there are 13 cards of each suit in a deck)?
- A.
29,446,560
- B.
1001
- C.
204,490
- D.
Not enough information
Correct Answer
C. 204,490Explanation
In order to find the number of 7-card hands with four spades and three hearts, we need to consider the combinations of selecting cards from the spades and hearts suits separately.
First, we choose 4 spades out of the 13 available spades, which can be done in C(13, 4) ways.
Next, we choose 3 hearts out of the 13 available hearts, which can be done in C(13, 3) ways.
Since these two selections are independent, we multiply the number of ways for each selection to get the total number of 7-card hands with four spades and three hearts: C(13, 4) * C(13, 3) = 204,490.
Therefore, the correct answer is 204,490.Rate this question:
-
- 5.
In a new group of 15 employees at a restaurant, 10 are to be assigned as servers, 3 are to be assigned as hosts, and 2 are to be assigned as cashiers. In how many ways can the assignment be made?
- A.
60
- B.
30,030
- C.
3014
- D.
50,000
Correct Answer
B. 30,030Explanation
There are 10 ways to assign the servers, 3 ways to assign the hosts, and 2 ways to assign the cashiers. By the multiplication principle, the total number of ways to make the assignment is 10 * 3 * 2 = 60. However, the question asks for the number of ways to make the assignment, not the total number of possible assignments. Therefore, we need to consider that the order in which the employees are assigned does not matter. So, we divide the total number of possible assignments by the number of ways to arrange the employees within each category. There are 10! ways to arrange the servers, 3! ways to arrange the hosts, and 2! ways to arrange the cashiers. Dividing 60 by (10! * 3! * 2!) gives us the final answer of 30,030.Rate this question:
-
- 6.
In how many ways can a first prize, a second prize and four identical third prizes be awarded to a group of 15 people?
- A.
5005
- B.
3,603,600
- C.
150,150
- D.
None of the answers
Correct Answer
C. 150,150Explanation
In this question, we need to determine the number of ways to award a first prize, a second prize, and four identical third prizes to a group of 15 people.
To calculate this, we use the concept of combinations. The first prize can be awarded to any one of the 15 people, the second prize can be awarded to any one of the remaining 14 people, and the four identical third prizes can be awarded to any combination of 4 people out of the remaining 13 people.
Therefore, the total number of ways to award the prizes is calculated as 15 * 14 * (13 choose 4), which simplifies to 150,150.Rate this question:
-
- 7.
There are 30 students in a statistics class. How many ways can the teacher pick out a group of 5 students?
- A.
150
- B.
17,100,720
- C.
142,506
- D.
None of the answers
Correct Answer
C. 142,506Explanation
The question asks for the number of ways the teacher can pick out a group of 5 students from a class of 30. This is a combination problem, where the order of the students does not matter. The formula to calculate the number of combinations is nCr = n! / (r!(n-r)!), where n is the total number of students and r is the number of students to be picked. In this case, n = 30 and r = 5. Plugging these values into the formula, we get 30! / (5!(30-5)!) = 30! / (5!25!) = (30 * 29 * 28 * 27 * 26) / (5 * 4 * 3 * 2 * 1) = 142,506. Therefore, the correct answer is 142,506.Rate this question:
-
- 8.
Pizza Hut offers 15 different toppings. Assuming no topping can be repeated on a single pizza, how many different 3 topping pizzas be created?
- A.
455
- B.
2730
- C.
45
- D.
None of the answers
Correct Answer
A. 455Explanation
To find the number of different 3-topping pizzas that can be created, we can use the combination formula. The formula for finding the number of combinations is nCr = n! / (r!(n-r)!), where n is the total number of items and r is the number of items chosen at a time. In this case, there are 15 different toppings to choose from and we want to choose 3 toppings for each pizza. Plugging in the values, we get 15! / (3!(15-3)!) = 455. Therefore, the correct answer is 455.Rate this question:
-
- 9.
10 people wait in line for a movie. How many different ways can the line be arranged?
- A.
3,628,800
- B.
1
- C.
100
- D.
1,000
Correct Answer
A. 3,628,800Explanation
The correct answer is 3,628,800. This is because there are 10 people waiting in line for a movie, and the number of different ways they can be arranged is given by the factorial of 10 (10!). The factorial of a number is the product of all positive integers less than or equal to that number. So, 10! = 10 x 9 x 8 x 7 x 6 x 5 x 4 x 3 x 2 x 1 = 3,628,800.Rate this question:
-
- 10.
Of the 40 dogs at the animal shelter, 12 are purebred. If 1 of the 40 dogs is selected at random, what is the probability that it is purebred?
- A.
.12
- B.
.30
- C.
.40
- D.
.70
Correct Answer
B. .30Explanation
The probability of selecting a purebred dog can be calculated by dividing the number of purebred dogs (12) by the total number of dogs (40). Therefore, the probability is 12/40, which simplifies to 0.3 or .30.Rate this question:
-
- 11.
How many 4-person committees can be formed from a club of 12 members?
- A.
11,880
- B.
495
- C.
48
- D.
3
Correct Answer
B. 495Explanation
The number of 4-person committees that can be formed from a club of 12 members can be calculated using the combination formula. The formula for combination is nCr = n! / (r!(n-r)!), where n is the total number of members and r is the number of members in each committee. In this case, n = 12 and r = 4. Plugging these values into the formula, we get 12C4 = 12! / (4!(12-4)!) = (12*11*10*9) / (4*3*2*1) = 495. Therefore, the answer is 495.Rate this question:
-
- 12.
Kareem has 4 sweaters, 6 shirts, and 3 pairs of slacks. How many distinct outfits, each consisting of a sweater, a shirt, and a pair of slacks, can Kareem select?
- A.
13
- B.
36
- C.
72
- D.
216
Correct Answer
C. 72Explanation
Kareem can select a sweater in 4 different ways, a shirt in 6 different ways, and a pair of slacks in 3 different ways. To find the total number of distinct outfits, we multiply these numbers together: 4 x 6 x 3 = 72. Therefore, Kareem can select 72 distinct outfits.Rate this question:
-
- 13.
A={1, 2, 3, 4}B={4, 5, 6, 7}
- A.
{4}
- B.
{1, 2, 3, 4, 5, 6, 7}
- C.
{5, 6, 7}
- D.
{1, 2, 3, 4, 5, 6, 7, 8}
Correct Answer
B. {1, 2, 3, 4, 5, 6, 7}Explanation
The correct answer is {1, 2, 3, 4, 5, 6, 7} because it is the union of sets A and B. The union of two sets is a set that contains all the elements from both sets, without any duplicates. In this case, set A contains the elements 1, 2, 3, and 4, while set B contains the elements 4, 5, 6, and 7. The union of A and B will therefore contain all these elements, resulting in {1, 2, 3, 4, 5, 6, 7}.Rate this question:
-
- 14.
A={1, 2, 3, 4}B={4, 5, 6, 7}
- A.
{4}
- B.
{1, 2, 3, 4, 5, 6, 7}
- C.
{5, 6, 7}
- D.
{1, 2, 3, 4, 5, 6, 7, 8}
Correct Answer
A. {4}Explanation
The correct answer is {4} because it is the intersection of sets A and B. The intersection of two sets is the set of elements that are common to both sets. In this case, the only element that is present in both sets A and B is 4. Therefore, the answer is {4}.Rate this question:
-
- 15.
A={1, 2, 3, 4, 5}B={4, 5, 6, 7}C={5, 6, 7, 8}
- A.
{1, 2, 3, 4, 5, 6, 7, 8}
- B.
{4, 5}
- C.
{5}
- D.
Empty set
Correct Answer
C. {5}Explanation
The correct answer is {5} because it is the only element that is present in all three sets A, B, and C.Rate this question:
-
- 16.
A={1, 2, 3, 4, 5}B={4, 5, 6, 7}C={5, 6, 7, 8}
- A.
{1, 2, 3, 4, 5}
- B.
{4, 5, 6, 7, 8}
- C.
{5}
- D.
{1, 2, 3, 4, 5, 6, 7, 8}
Correct Answer
B. {4, 5, 6, 7, 8}Explanation
The correct answer is {4, 5, 6, 7, 8} because it is the union of sets B and C, which includes all the elements present in both sets.Rate this question:
-
- 17.
A certain bank issues 3-letter identification codes to its customers. If each letter can be used only once per code, how many different codes are possible?
- A.
26
- B.
78
- C.
15,600
- D.
326
Correct Answer
C. 15,600Explanation
Since each letter can only be used once per code, the first letter of the code can be any of the 26 letters of the alphabet. After choosing the first letter, there are 25 letters remaining to choose from for the second letter. Finally, there are 24 letters remaining to choose from for the third letter. Therefore, the total number of different codes possible is 26 * 25 * 24 = 15,600.Rate this question:
-
- 18.
A restaurant’s fixed-price special dinner consists of an appetizer, an entrée, and dessert. If the restaurant offers 5 different types of appetizers, 5 different types of entrees, and 4 different types of desserts, how many different ways to order a fixed-price special dinner?
- A.
60
- B.
100
- C.
200
- D.
3000
Correct Answer
B. 100Explanation
There are 5 choices for the appetizer, 5 choices for the entrée, and 4 choices for the dessert. By the multiplication principle, the total number of ways to order a fixed-price special dinner is equal to the product of the number of choices for each course: 5 x 5 x 4 = 100. Therefore, there are 100 different ways to order a fixed-price special dinner.Rate this question:
-
- 19.
A menu offers 4 choices for the first course, 5 choices for the second course, and 2 courses for dessert. How many different meals, consisting of a first course, a second course, and a dessert, can one choose from this menu?
- A.
60
- B.
40
- C.
20
- D.
10
Correct Answer
B. 40Explanation
There are 4 choices for the first course, 5 choices for the second course, and 2 choices for dessert. To find the total number of different meals, we multiply the number of choices for each course together: 4 * 5 * 2 = 40. Therefore, there are 40 different meals that one can choose from this menu.Rate this question:
-
- 20.
A={1}B={2, 3, 4}C={10, 12}
- A.
{1, 2, 3, 4, 10, 12}
- B.
{1, 2, 3, 4, 5, 6, 7, 8, 9, 10, 11, 12}
- C.
Empty set
- D.
{10, 12}
Correct Answer
A. {1, 2, 3, 4, 10, 12}Explanation
The correct answer is the union of sets A, B, and C. It includes all the elements from each set without any repetition. Therefore, the correct answer is {1, 2, 3, 4, 10, 12}.Rate this question:
-
- 21.
A certain bank issues 4-digit identification codes to its customers using numbers 0, 1, 2, ..., 9. How many different codes are possible?
- A.
40
- B.
100
- C.
1000
- D.
10,000
Correct Answer
D. 10,000Explanation
The bank can issue a 4-digit identification code using any of the numbers 0, 1, 2, ..., 9. Since there are 10 possible choices for each digit, there are a total of 10 choices for the first digit, 10 choices for the second digit, 10 choices for the third digit, and 10 choices for the fourth digit. Therefore, the total number of different codes possible is 10 x 10 x 10 x 10 = 10,000.Rate this question:
-
- 22.
John needs to pick up his clothes for the day. He can choose from 6 different shirts, 4 different pairs of pants, and 8 different socks. If an outfit consists of 1 shirt, 1 pair of pants, and 2 socks, how many different outfits could he choose?
- A.
192
- B.
672
- C.
6,720
- D.
10,000
Correct Answer
B. 672Explanation
John can choose one shirt from 6 options, one pair of pants from 4 options, and two socks from 8 options. The number of different outfits he could choose can be calculated by multiplying the number of options for each item together: 6 * 4 * (8 choose 2) = 6 * 4 * 28 = 672.Rate this question:
-
- 23.
At the school cafeteria, 4 boys and 3 girls are forming a lunch line. If the boys must stand in the first two and last two places in line, how many different lines can be formed?
- A.
24
- B.
6
- C.
144
- D.
12
Correct Answer
C. 144Explanation
There are 4 boys and 3 girls forming the lunch line. The boys must stand in the first two and last two places in line. Therefore, the first two places can be filled with the 4 boys in 4P2 ways, and the last two places can also be filled with the 4 boys in 4P2 ways. The remaining 3 places in the middle can be filled with the 3 girls in 3P3 ways. Therefore, the total number of different lines that can be formed is 4P2 * 4P2 * 3P3 = 144.Rate this question:
-
- 24.
There are 10 different cereals at the grocery store. How many different ways can you choose 3 boxes of cereal (you cannot pick two of the same type)?
- A.
30
- B.
120
- C.
720
- D.
100
Correct Answer
B. 120Explanation
To find the number of different ways to choose 3 boxes of cereal out of 10 different options, we can use the concept of combinations. Since the order in which the boxes are chosen does not matter, we can use the formula for combinations. The formula is nCr = n! / (r!(n-r)!), where n is the total number of options and r is the number of choices. In this case, n = 10 and r = 3. Plugging in the values, we get 10! / (3!(10-3)!) = 10! / (3!7!) = (10 * 9 * 8) / (3 * 2 * 1) = 120. Therefore, there are 120 different ways to choose 3 boxes of cereal.Rate this question:
-
- 25.
There are 10 different cereals at the grocery store. 5 of the 10 cereals are made by General Mills. What is the probability of randomly choosing 3 boxes and having all three be General MIlls brand?
- A.
1/10
- B.
1/120
- C.
1/12
- D.
1500
Correct Answer
C. 1/12Explanation
The probability of randomly choosing a General Mills cereal from the 10 different cereals at the grocery store is 5/10, or 1/2. Since we want to choose 3 boxes and have all three be General Mills brand, we need to multiply the probability of choosing a General Mills cereal three times. Therefore, the probability is (1/2) * (1/2) * (1/2) = 1/8. However, the question asks for the probability of randomly choosing 3 boxes and having all three be General Mills brand out of the total 10 cereals, not just the General Mills cereals. So, we need to divide the previous probability by the total number of cereals, which is 10. Therefore, the correct answer is 1/8 divided by 10, which simplifies to 1/80.Rate this question:
-
- 26.
There are 10 different cereals at the grocery store. 5 of the 10 cereals are made by General Mills. What is the probability of randomly choosing 3 boxes and having none of the three boxes be General Mills?
- A.
1/10
- B.
1/120
- C.
1/12
- D.
1500
Correct Answer
C. 1/12Explanation
The probability of randomly choosing a box that is not made by General Mills is 5/10, since there are 5 cereals made by General Mills out of a total of 10 cereals. To find the probability of choosing 3 boxes that are not made by General Mills, we multiply the probabilities together: (5/10) * (4/9) * (3/8). Simplifying this expression gives us 1/12, which is the probability of randomly choosing 3 boxes and having none of them be General Mills.Rate this question:
-
- 27.
A phone number has 10 digits (0, 1, 2, ..., 9). How many phone numbers consist of 10 digits where none of the digits repeat?
- A.
1,000,000,000
- B.
3,628,800
- C.
5,000,000
- D.
1,000
Correct Answer
B. 3,628,800Explanation
To find the number of phone numbers consisting of 10 digits where none of the digits repeat, we can use the concept of permutations. The first digit can be any of the 10 available digits (0-9). After choosing the first digit, the second digit can be any of the remaining 9 digits. Similarly, the third digit can be chosen from the remaining 8 digits, and so on. Therefore, the total number of phone numbers is 10 * 9 * 8 * 7 * 6 * 5 * 4 * 3 * 2 * 1, which is equal to 3,628,800.Rate this question:
-
- 28.
A phone number has 10 digits (0, 1, 2, ..., 9). How different phone numbers are there?
- A.
1x10^10
- B.
1,000
- C.
1x10^100
- D.
3,628,800
Correct Answer
A. 1x10^10Explanation
There are 10 digits available (0, 1, 2, ..., 9) and each digit can be chosen independently for each position in the phone number. Therefore, the total number of different phone numbers that can be formed is equal to the number of choices for each position multiplied together. Since there are 10 positions in a phone number, the total number of different phone numbers is 1x10^10.Rate this question:
-
- 29.
A jar contains 4 green marbles, 5 red marbles, and 11 white marbles. If one marble is chosen at random, what is the probability that it will be green?
- A.
1/5
- B.
1/4
- C.
20
- D.
1
Correct Answer
A. 1/5Explanation
The probability of choosing a green marble can be calculated by dividing the number of green marbles by the total number of marbles. In this case, there are 4 green marbles out of a total of 20 marbles (4 + 5 + 11). Therefore, the probability of choosing a green marble is 4/20, which simplifies to 1/5.Rate this question:
-
- 30.
A jar contains 4 green marbles, 5 red marbles, and 11 white marbles. If one marble is chosen at random, what is the probability that it will NOT be green?
- A.
4/5
- B.
1/5
- C.
20
- D.
3/4
Correct Answer
A. 4/5Explanation
The probability of an event occurring is equal to the number of favorable outcomes divided by the total number of possible outcomes. In this case, there are 4 green marbles out of a total of 4 green marbles + 5 red marbles + 11 white marbles = 20 marbles. Therefore, the probability of not choosing a green marble is equal to 1 - (4/20) = 16/20 = 4/5.Rate this question:
-
Quiz Review Timeline +
Our quizzes are rigorously reviewed, monitored and continuously updated by our expert board to maintain accuracy, relevance, and timeliness.
-
Current Version
-
Sep 03, 2023Quiz Edited by
ProProfs Editorial Team -
Dec 07, 2010Quiz Created by
Ichihana
- Algebra Quizzes
- Arithmetic Quizzes
- Calculus Quizzes
- Data Handling Quizzes
- Decimal Quizzes
- Discrete Mathematics Quizzes
- Equation Quizzes
- Fraction Quizzes
- Function Quizzes
- Geometry Quizzes
- Graph Quizzes
- Measurement Quizzes
- Number Quizzes
- Percentage Quizzes
- Place Value Quizzes
- Problem Solving Quizzes
- Ratio Quizzes
- Shape Quizzes
- Trigonometry Quizzes
Back to top