Midterm Review - Definitions, Theorems And Postulates

Reviewed by Editorial Team
The ProProfs editorial team is comprised of experienced subject matter experts. They've collectively created over 10,000 quizzes and lessons, serving over 100 million users. Our team includes in-house content moderators and subject matter experts, as well as a global network of rigorously trained contributors. All adhere to our comprehensive editorial guidelines, ensuring the delivery of high-quality content.
Learn about Our Editorial Process
| By Annacabral
A
Annacabral
Community Contributor
Quizzes Created: 29 | Total Attempts: 21,108
| Attempts: 485
Quiz Flashcard
SettingsSettings
Please wait...
  • 1/12 Questions
    Which congruency theorem/postulate proves that the triangles are congruent? - ProProfs

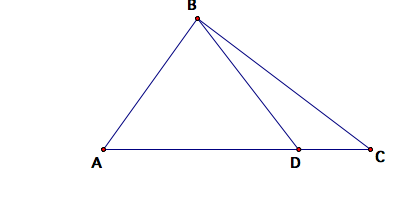
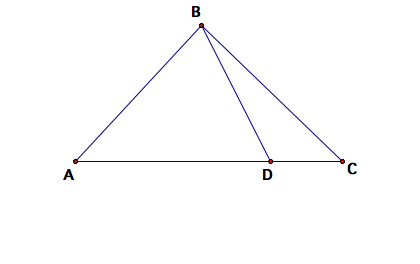
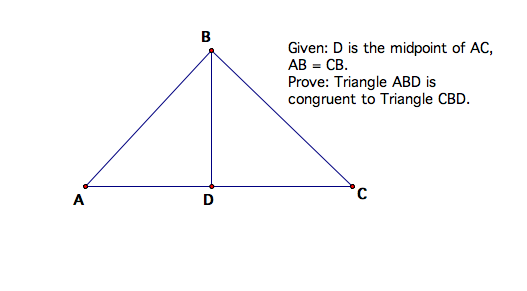
    Which congruency theorem/postulate proves that the triangles are congruent?

    • ASA
    • SAS or SSS
    • Not possible
Please wait...
Midterm Review - Definitions, Theorems And Postulates - Quiz
About This Quiz

This Midterm Review assesses understanding of geometry through definitions, theorems, and postulates. It tests knowledge on triangle congruency, angle relationships, and geometric proofs, enhancing learners' skills in logical reasoning and spatial visualization.

Quiz Preview

  • 2. 
    What series of reasons will give you DBC < BDC if you are given ABD > DBC? - ProProfs

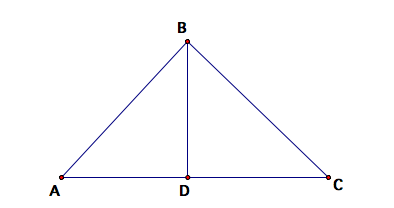
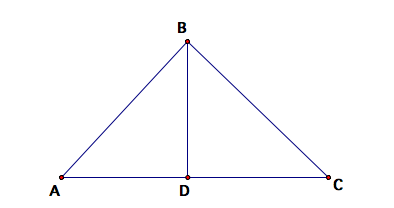
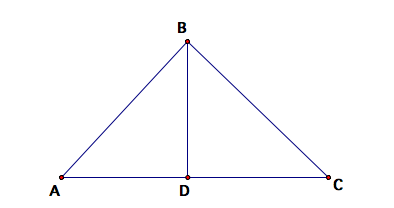
    What series of reasons will give you DBC < BDC if you are given ABD > DBC?

    • GAGS, Exterior Angle Theorem

    • Exterior Angle Theorem, Transitive Property

    • Triangle Inequality Theorem, Substitution

    Correct Answer
    A. Exterior Angle Theorem, Transitive Property
    Explanation
    If we are given ABD > DBC, it means that the angle ABD is greater than the angle DBC. According to the Exterior Angle Theorem, the exterior angle of a triangle is equal to the sum of its two remote interior angles. Therefore, the exterior angle ABD is equal to the sum of angles DBC and BDC. Since ABD is greater than DBC, it implies that the sum of DBC and BDC (which is the exterior angle ABD) is greater than DBC alone. This can be represented as DBC < BDC. The Transitive Property allows us to conclude that if ABD > DBC and DBC < BDC, then ABD > BDC.

    Rate this question:

  • 3. 
    If Triangle ABC is isosceles with AB = CB, what angles can you say are congruent by Isosceles Triangle Theorem? - ProProfs

    If Triangle ABC is isosceles with AB = CB, what angles can you say are congruent by Isosceles Triangle Theorem?

    • Angle A = Angle C

    • Angle ABD = Angle CBD

    • Angle ADB = Angle CDB

    Correct Answer
    A. Angle A = Angle C
    Explanation
    By the Isosceles Triangle Theorem, if a triangle is isosceles with two sides congruent, then the angles opposite those sides are also congruent. Therefore, in triangle ABC, since AB = CB, we can conclude that Angle A is congruent to Angle C.

    Rate this question:

  • 4. 
    Why is angle CDB > Angle A? - ProProfs

    Why is angle CDB > Angle A?

    • Whole > Part

    • GAGS

    • Transitive Property

    • Exterior Angle > Remote Interior Angle

    • Triangle Inequality Theorem

    Correct Answer
    A. Exterior Angle > Remote Interior Angle
    Explanation
    The given answer suggests that the reason angle CDB is greater than angle A is because of the property that an exterior angle of a triangle is always greater than its corresponding remote interior angle. In this case, angle CDB is an exterior angle of triangle CDB, while angle A is a remote interior angle of triangle CDB. Therefore, angle CDB must be greater than angle A.

    Rate this question:

  • 5. 
    If you are given that G is the midpoint of FH and EI, what reason tells you that Triangle EFG is congruent to Triangle IHG? - ProProfs

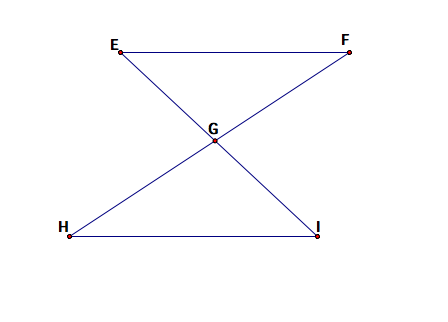
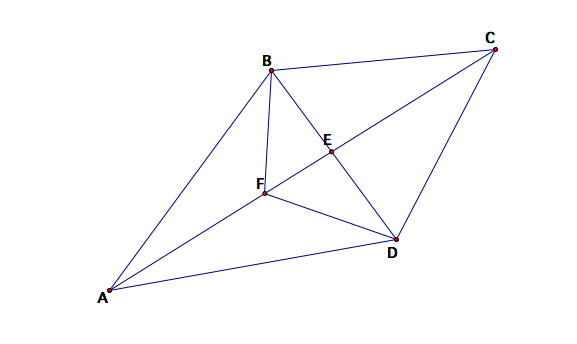
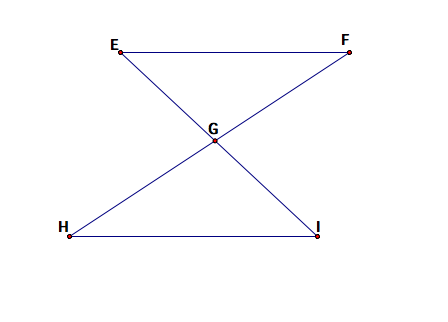
    If you are given that G is the midpoint of FH and EI, what reason tells you that Triangle EFG is congruent to Triangle IHG?

    • SSS

    • ASA

    • SAS

    Correct Answer
    A. SAS
    Explanation
    Given that G is the midpoint of FH and EI, we can conclude that FG is congruent to GH and EG is congruent to GI. This is because the midpoint of a line segment divides it into two congruent segments. Additionally, we know that angle EGF is congruent to angle IHG because they are vertical angles. Therefore, we have two pairs of corresponding sides and one pair of corresponding angles that are congruent, satisfying the SAS (Side-Angle-Side) congruence criterion. Hence, Triangle EFG is congruent to Triangle IHG.

    Rate this question:

  • 6. 
    Why is angle ABC > angle ABD? - ProProfs

    Why is angle ABC > angle ABD?

    • Whole > Part

    • GAGS

    • Transitive Property

    • Exterior Angle > Remote Interior angle

    • Triangle Inequality Theorem

    Correct Answer
    A. Whole > Part
    Explanation
    The given answer "Whole > Part" suggests that angle ABC is greater than angle ABD because in a triangle, the whole angle (angle ABC) is always greater than any of its parts or interior angles (angle ABD). This is a basic concept in geometry where the sum of the interior angles of a triangle is always 180 degrees. Therefore, angle ABC, being a part of the triangle, must be less than 180 degrees and greater than angle ABD.

    Rate this question:

  • 7. 
    If AF = CE, what reason tells you that AE = CF? - ProProfs

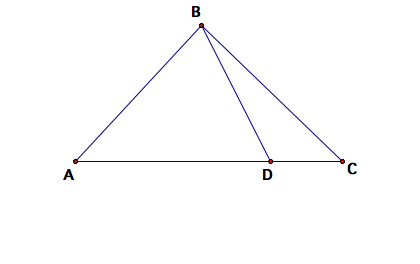
    If AF = CE, what reason tells you that AE = CF?

    • Whole > Part

    • Isosceles Triangle Theorem

    • Segment Addition Theorem

    • Angle Addition Theorem

    • CPCTC

    Correct Answer
    A. Segment Addition Theorem
    Explanation
    The Segment Addition Theorem states that if three points A, B, and C are collinear and B is between A and C, then AB + BC = AC. In this case, AF = CE implies that AB + BF = AC. Since AE is a part of AC and CF is a part of BF, the Segment Addition Theorem can be applied to conclude that AE + EF = AF, which implies that AE = CF.

    Rate this question:

  • 8. 
    If BD is the perpendicular bisector of side AC, what reason tells you that triangle ADB is congruent to triangle CDB? - ProProfs

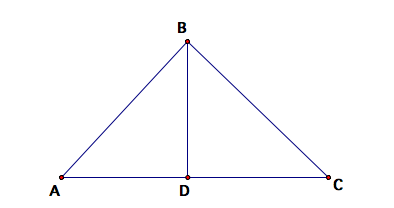
    If BD is the perpendicular bisector of side AC, what reason tells you that triangle ADB is congruent to triangle CDB?

    • SSS

    • ASA

    • SAS

    Correct Answer
    A. SAS
    Explanation
    The reason that triangle ADB is congruent to triangle CDB is the SAS (Side-Angle-Side) congruence criterion. This criterion states that if two sides and the included angle of one triangle are congruent to the corresponding sides and included angle of another triangle, then the triangles are congruent. In this case, the sides AD and CD are congruent because BD is the perpendicular bisector of AC, and the included angle at D is congruent because BD is the perpendicular bisector. Therefore, by the SAS criterion, triangle ADB is congruent to triangle CDB.

    Rate this question:

  • 9. 
    If you were given Triangle AFB congruent to Triangle AFD, what reason would tell you that angle ABF = Angle AD? - ProProfs

    If you were given Triangle AFB congruent to Triangle AFD, what reason would tell you that angle ABF = Angle AD?

    • Isosceles Triangle Theorem

    • Angle Addition Theorem

    • CPCTC

    Correct Answer
    A. CPCTC
    Explanation
    CPCTC stands for "Corresponding Parts of Congruent Triangles are Congruent." This theorem states that if two triangles are congruent, then their corresponding parts, such as angles and sides, are also congruent. In this case, since Triangle AFB is congruent to Triangle AFD, CPCTC tells us that angle ABF is congruent to angle AD. Therefore, the reason that angle ABF = Angle AD is CPCTC.

    Rate this question:

  • 10. 
    Name the reason for the following conclusion.If AC is perpendicular to BD, why is angle ADB = to angle CDB? - ProProfs

    Name the reason for the following conclusion.If AC is perpendicular to BD, why is angle ADB = to angle CDB?

    • Perpendicular lines form right angles

    • Perpendicular lines form congruent angles.

    • Angles ADB and CDB form a linear pair

    • Angles ADB and CDB are supplementary

    Correct Answer
    A. Perpendicular lines form congruent angles.
    Explanation
    While all the above reasons are valid, the second one is the most direct. You could also say perpendicular lines form right angles and then all right angles are equal.

    Rate this question:

  • 11. 
    If BD is perpendicular to AC and bisects angle ABC, what reason says triangle ADB is congruent to triangle CDB? - ProProfs

    If BD is perpendicular to AC and bisects angle ABC, what reason says triangle ADB is congruent to triangle CDB?

    • SSS

    • ASA

    • SAS

    Correct Answer
    A. ASA
    Explanation
    The given information states that BD is perpendicular to AC and also bisects angle ABC. This means that angle ABD is congruent to angle CBD. Additionally, we know that BD is common to both triangles ADB and CDB. Therefore, we have angle ABD congruent to angle CBD, side BD shared by both triangles, and angle B congruent to itself (reflexive property). These conditions satisfy the ASA (Angle-Side-Angle) congruence criterion, which states that if two angles and the included side of one triangle are congruent to two angles and the included side of another triangle, then the triangles are congruent. Hence, triangle ADB is congruent to triangle CDB.

    Rate this question:

  • 12. 
    What additional information would need to be given to prove Triangle EGF is congruent to Triangle IGH by ASA if you are given G is the midpoint of HF - ProProfs

    What additional information would need to be given to prove Triangle EGF is congruent to Triangle IGH by ASA if you are given G is the midpoint of HF

    • G is also the midpoint of HI

    • EF = IH

    • Angle F = Angle H

    • Angle E = Angle I

    • EI is perpendicular to FH

    Correct Answer
    A. Angle F = Angle H
    Explanation
    Angle E = Angle I gives you AAS, not ASA. Don't miss vertical angles are equal!

    Rate this question:

Quiz Review Timeline (Updated): Mar 20, 2023 +

Our quizzes are rigorously reviewed, monitored and continuously updated by our expert board to maintain accuracy, relevance, and timeliness.

  • Current Version
  • Mar 20, 2023
    Quiz Edited by
    ProProfs Editorial Team
  • Dec 04, 2010
    Quiz Created by
    Annacabral
Back to Top Back to top
Advertisement
×

Wait!
Here's an interesting quiz for you.

We have other quizzes matching your interest.