Act Quiz 1 (Alg II+)
-
If 3x + 7 = 15x - 2, then x = ?
-
5/18
-
5/12
-
1/2
-
3/4
-
No solution
-
ACT Quiz 1 (Alg II+) assesses advanced algebra skills, focusing on solving equations, understanding ratios, and working with complex numbers. It prepares students for the ACT, enhancing their problem-solving abilities and readiness for college-level math.
(136).webp)
Quiz Preview
- 2.
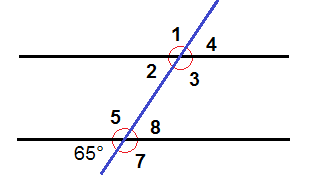
Two parallel lines are cut by a transversal as shown at the left. What is the sum of angles 2, 5 and 8?
-
65
-
115
-
180
-
245
-
360
Correct Answer
A. 245Explanation
When two parallel lines are cut by a transversal, corresponding angles are congruent. In the given diagram, angles 2 and 8 are corresponding angles, so they are congruent. Therefore, angle 2 + angle 8 = angle 5. Thus, the sum of angles 2, 5, and 8 is 2(angle 5). Since angle 5 is a straight angle, it measures 180 degrees. Therefore, 2(angle 5) = 2(180) = 360 degrees.Rate this question:
-
- 3.
The ratio of girls to boys in a club is 6:7. If the total number of students is 39, how many more boys are there than girls?
-
1
-
2
-
3
-
13
-
21
Correct Answer
A. 3Explanation
The ratio of girls to boys in the club is 6:7, which means that for every 6 girls, there are 7 boys. If the total number of students is 39, we can set up the equation 6x + 7x = 39, where x represents the common ratio. Solving for x, we find that x = 3. Therefore, there are 6x = 6 * 3 = 18 girls and 7x = 7 * 3 = 21 boys. The difference between the number of boys and girls is 21 - 18 = 3.Rate this question:
-
- 4.
When 91/333 is expressed as a decimal number what is the 60th digit after the decimal point?
-
0
-
1
-
2
-
3
-
7
Correct Answer
A. 3Explanation
To find the 60th digit after the decimal point when expressing 91/333 as a decimal number, we need to divide 91 by 333 using long division. The remainder after each division will give us the digits after the decimal point. After performing the long division, we find that the remainder for the 60th digit is 3. Therefore, the answer is 3.Rate this question:
-
- 5.
If f(x) = x2 + 3x, then f(b - 3) = ?
-
B2 + 9
-
B2 + 9 + 3x
-
B2 - b - 6
-
B2 + 9b + 18
-
B2 - 3b
Correct Answer
A. B2 - 3bExplanation
The given function f(x) = x^2 + 3x. To find f(b - 3), we substitute (b - 3) in place of x in the function. So, f(b - 3) = (b - 3)^2 + 3(b - 3). Simplifying this expression gives b^2 - 6b + 9 + 3b - 9, which further simplifies to b^2 - 3b. Therefore, the correct answer is b^2 - 3b.Rate this question:
-
- 6.
Which of the following is the correct simplification of the expression (4 + 3i) - (6 - 5i)?
-
-2 + 8i
-
-2 - 2i
-
-2 - 8i
-
2 + 8i
-
2 - 8i
Correct Answer
A. -2 + 8iExplanation
To simplify the expression (4 + 3i) - (6 - 5i), we need to distribute the negative sign to both terms inside the parentheses. This gives us 4 + 3i - 6 + 5i. Combining like terms, we get -2 + 8i. Therefore, the correct simplification of the expression is -2 + 8i.Rate this question:
-
- 7.
At the Farmer's Market, I can buy a coffee for $3 and loaves of bread for $5. Over the summer, if I buy 26 items and spend $90, how many coffees did I buy?
-
5
-
10
-
15
-
20
-
25
Correct Answer
A. 20Explanation
If the person bought 26 items and spent $90, and each coffee costs $3, then the person must have bought 20 coffees in order to reach a total of $90.Rate this question:
-
- 8.
What is the y - intercept of the line that passes through the points (2, 35) and (7, 50)?
-
3
-
10
-
12
-
27
-
29
Correct Answer
A. 29Explanation
The y-intercept of a line is the value of y when x is equal to 0. To find the equation of the line that passes through the points (2, 35) and (7, 50), we can use the slope-intercept form of a line, which is y = mx + b. We first need to find the slope (m) of the line using the formula (y2 - y1)/(x2 - x1), which gives us (50 - 35)/(7 - 2) = 15/5 = 3. Now we can substitute one of the points into the equation to solve for the y-intercept (b). Using the point (2, 35), we get 35 = 3(2) + b, which simplifies to b = 35 - 6 = 29. Therefore, the y-intercept of the line is 29.Rate this question:
-
- 9.
Point P (1, 3) and Point Q (6, -9) are points on the (x, y) coordinate plane. Find the distance between the two points.
-
3
-
9
-
11
-
12
-
13
Correct Answer
A. 13Explanation
To find the distance between two points on a coordinate plane, we can use the distance formula, which is derived from the Pythagorean theorem. The formula is: distance = √((x2 - x1)^2 + (y2 - y1)^2). In this case, the coordinates of point P are (1, 3) and the coordinates of point Q are (6, -9). Plugging these values into the formula, we get: distance = √((6 - 1)^2 + (-9 - 3)^2) = √(5^2 + (-12)^2) = √(25 + 144) = √169 = 13. Therefore, the distance between point P and point Q is 13.Rate this question:
-
- 10.
There are five pig weights (in pounds) from the fair: 250, 270, 260, 275, 300. Approximate the standard deviation for this data set.
-
17
-
21
-
24
-
49
-
271
Correct Answer
A. 17Explanation
The given data set consists of five pig weights: 250, 270, 260, 275, and 300 pounds. To approximate the standard deviation, we can calculate the mean of the data set, which is 271 pounds. Then, we subtract each value from the mean, square the result, and calculate the average of these squared differences. Taking the square root of this average gives us the standard deviation. In this case, the calculated standard deviation is approximately 17 pounds.Rate this question:
-
Quiz Review Timeline (Updated): Feb 22, 2024 +
Our quizzes are rigorously reviewed, monitored and continuously updated by our expert board to maintain accuracy, relevance, and timeliness.
-
Current Version
-
Feb 22, 2024Quiz Edited by
ProProfs Editorial Team -
Sep 25, 2018Quiz Created by
CorvallisMath
Formerly American College Testing Quiz!
Explore the Formerly American College Testing Quiz! This quiz covers basics about the ACT, such as its format, frequency, origin, and skills assessed like reading. Ideal for...
Questions:
10 |
Attempts:
123 |
Last updated:
Mar 20, 2023
|
ACT Vocabulary Quiz 1
Are you almost sitting for your ACT's? Well, if you are, I am sure you are nervous and anxious of the test. ACT vocabulary quiz below will help you prepare for the...
Questions:
12 |
Attempts:
135 |
Last updated:
Mar 13, 2024
|
ACT Practice Test 2014-2015 (1-30)
This ACT Practice Test for 2014-2015 includes 30 questions covering various subjects to help prepare students for the ACT exam.
Questions:
30 |
Attempts:
130 |
Last updated:
May 08, 2024
|
What We Should Study For Act?
Would you ,like to know if what you should study for act? Let's find out!
Questions:
8 |
Attempts:
120 |
Last updated:
Mar 20, 2022
|
ACT 5-in-5 #6-10 Recap
This ACT 5-in-5 #6-10 Recap quiz challenges learners with math-related problems including geometry, algebra, and arithmetic calculations. It assesses skills in problem-solving,...
Questions:
12 |
Attempts:
481 |
Last updated:
Mar 21, 2023
|
ACT 5-in-5 Trivia Quiz
The ACT 5-in-5 Trivia Quiz challenges learners with questions typical of the ACT exam, focusing on algebra and practical math applications. It assesses key skills necessary for...
Questions:
5 |
Attempts:
851 |
Last updated:
Mar 20, 2023
|
 Back to top
Back to top