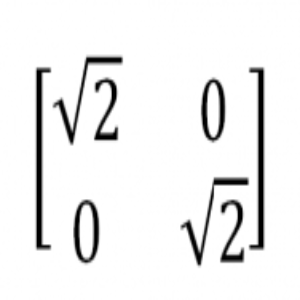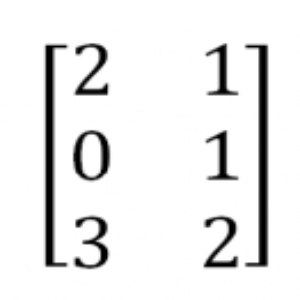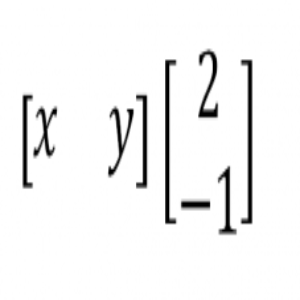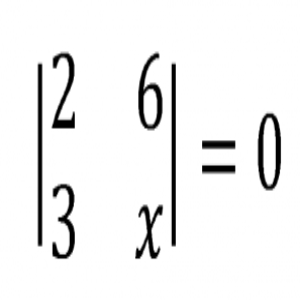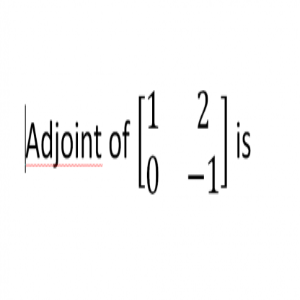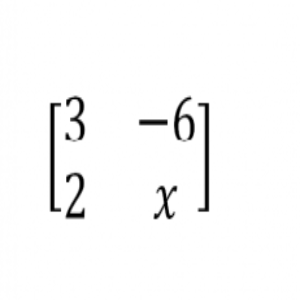Math Quiz For Class 9th
-
The idea of Matrices was given by
-
Arthur Cayley
-
Briggs
-
Al-Khawarzmi
-
John Napier
-
This Math Quiz for 9th grade covers fundamental concepts of matrices including their order, properties, and operations. It is designed to test and reinforce students' understanding of matrix theory.

- 2.
The order of matrix [ 2 1] is __________
-
2-by-1
-
1-by-2
-
1-by-1
-
2-by-2
Correct Answer
A. 1-by-2Explanation
The order of a matrix is determined by the number of rows and columns it has. In this case, the matrix is given as [2 1], which means it has 1 row and 2 columns. Therefore, the order of the matrix is 1-by-2.Rate this question:
-
- 3.
It is called ____________ matrix:
-
Zero
-
Unit
-
Scalar
-
Singular
Correct Answer
A. ScalarExplanation
A scalar matrix is a special type of square matrix where all the elements on the main diagonal are equal and all other elements are zero. In this case, the given matrix is a scalar matrix because all the elements are zero except for the main diagonal, which contains the same value.Rate this question:
-
- 4.
Order of transpose is _____________:
-
3-by-2
-
2-by-3
-
3-by-1
-
1-by-3
Correct Answer
A. 2-by-3Explanation
The order of transpose is determined by the dimensions of the original matrix. In this case, the original matrix is 2-by-3, which means it has 2 rows and 3 columns. When we take the transpose of this matrix, the rows become columns and the columns become rows, resulting in a matrix with dimensions 3-by-2.Rate this question:
-
- 5.
Product of
-
[2x+y]
-
[x-2y]
-
[2x-y]
-
[x+2y]
Correct Answer
A. [2x-y]Explanation
The given expression is a product of four terms: [2x+y], [x-2y], [2x-y], and [x+2y]. The correct answer, [2x-y], is obtained by multiplying the terms together. When multiplying the terms, we use the distributive property and combine like terms. The resulting expression simplifies to 4x^2 - 9y^2. Therefore, the correct answer is [2x-y].Rate this question:
-
- 6.
X is equal to _______________ if :
-
9
-
-6
-
6
-
-9
Correct Answer
A. 9Explanation
If x is equal to 9, then it satisfies the given conditions because 9 is the only number in the list that is equal to itself. The other numbers (-6, 6, -9) are not equal to 9, so they do not satisfy the condition. Therefore, the correct answer is 9.Rate this question:
-
- 7.
Adjoint of
-
Option
-
Option
-
Option
-
Option
Correct Answer
A. Option -
- 8.
For ______ value of x, will be singular matrix.
-
3
-
-4
-
3
-
4
Correct Answer
A. -4 -
- 9.
Arthur Cayley introduced the "Theory of Matrices" in ____________
-
1854
-
1856
-
1858
-
1860
Correct Answer
A. 1858 -
- 10.
Order of square matrix is:
-
2-by-2
-
1-by-2
-
2-by-1
-
3-by-2
Correct Answer
A. 2-by-2Explanation
The order of a square matrix refers to the number of rows and columns it has. In this case, the correct answer is 2-by-2, which means the matrix has 2 rows and 2 columns. This is determined by the given options, where the other options have either different numbers of rows or columns, making them not square matrices.Rate this question:
-
- 11.
A square matrix M is called to be skew symmetric, if:
-
Mt=Mt
-
Mt=1/M
-
Mt=-M
-
Mt = M
Correct Answer
A. Mt=-MExplanation
A square matrix M is called skew symmetric if its transpose is equal to the negative of the matrix itself. In other words, if Mt = -M. This means that the elements above the main diagonal of M are equal to the corresponding elements below the main diagonal, but with opposite signs. The correct answer states that Mt=-M, which is the correct condition for a matrix to be skew symmetric.Rate this question:
-
- 12.
When the number of rows is not equal to the number of columns, the matrix is called____________:
-
Square matrix
-
Rectangular matrix
-
Symmetric matrix
-
Row matrix
Correct Answer
A. Rectangular matrixExplanation
A rectangular matrix is a matrix where the number of rows is not equal to the number of columns. In other words, it has a different number of rows and columns, creating a rectangular shape. This is in contrast to a square matrix, which has an equal number of rows and columns. A symmetric matrix is a square matrix that is equal to its transpose, and a row matrix is a matrix with only one row.Rate this question:
-
- 13.
x is equal to, If
-
Option
-
Option
-
Option
-
Option
Correct Answer
A. Option -
- 14.
Real part of 2ab(i+i2) is ______________
-
-2ab
-
2ab
-
3ab
-
-3ab
Correct Answer
A. -2abExplanation
The expression 2ab(i+i^2) can be simplified as 2ab(i-1), since i^2 is equal to -1. The real part of a complex number is the part without the imaginary unit, so the real part of 2ab(i-1) is -2ab.Rate this question:
-
- 15.
Ifx,y,z ∈ R, z<0 then, x<y⇒ _____________:
-
Xz
-
Xz>yz
-
Xz=yz
-
None of these
Correct Answer
A. Xz>yzExplanation
If x, y, and z are real numbers and z is less than 0, then x < y implies that xz > yz. This can be explained by considering that when z is negative, multiplying both sides of the inequality x < y by z will result in a reversal of the inequality sign. Therefore, xz will be greater than yz.Rate this question:
-
- 16.
The value of i9 is ____________
-
1
-
-1
-
I
-
-i
Correct Answer
A. IExplanation
The value of i9 is i because i is the imaginary unit, which represents the square root of -1. When i is raised to the power of 9, it cycles through a pattern where the powers of i repeat every 4 terms: i, -1, -i, 1. Since 9 is equivalent to 8 + 1, the power of i can be simplified to i8 * i1. i8 is equal to 1, so the value of i9 is i.Rate this question:
-
- 17.
Imaginary part of -i(3i+2) is ___________
-
-2
-
2
-
3
-
-3
Correct Answer
A. -2Explanation
To find the imaginary part of -i(3i+2), we need to simplify the expression. Distributing -i to both terms inside the parentheses, we get -i(3i) - i(2). Simplifying further, -i(3i) becomes 3i^2, and since i^2 is equal to -1, 3i^2 becomes -3. -i(2) simplifies to -2i. Combining the two terms, we have -3 - 2i. The imaginary part of this expression is the coefficient of i, which is -2. Therefore, the correct answer is -2.Rate this question:
-
- 18.
(3+√ 2)(3-√ 2) is equal to
-
7
-
-7
-
-1
-
1
Correct Answer
A. 7Explanation
The given expression can be simplified using the formula (a+b)(a-b) = a^2 - b^2. In this case, a = 3 and b = √2. Substituting these values into the formula, we get (3^2 - (√2)^2), which simplifies to (9 - 2), resulting in 7. Therefore, the correct answer is 7.Rate this question:
-
- 19.
(27x-1)-2/3
-
3√x2/9
-
√x3/9
-
3√x2/8
-
√x3/8
Correct Answer
A. 3√x2/9Explanation
The given expression can be simplified by first simplifying the expression inside the parentheses. The expression (27x - 1) can be simplified further by distributing the -2/3 to both terms inside the parentheses. This gives us -54x/3 + 2/3. Simplifying this further, we get -18x + 2/3. Next, we need to simplify the expression outside the parentheses. Taking the cube root of x^2/9 gives us (x^2/9)^(1/3) which simplifies to (x^2)^(1/3)/(9)^(1/3). Simplifying this further, we get x^(2/3)/3. Therefore, the correct answer is 3√x^2/9.Rate this question:
-
- 20.
Write 42/3 with radical sign:
-
3√42
-
√43
-
3√43
-
√46
Correct Answer
A. 3√42Explanation
The correct answer is 3√42. This is because the radical sign (√) represents the square root of a number. In this case, 3√42 means the cube root of 42. Cube root is the number that when multiplied by itself three times gives the original number. Therefore, the cube root of 42 is 3√42.Rate this question:
-
- 21.
In 3√35 the radicand is
-
3
-
1/3
-
35
-
(35)1/3
Correct Answer
A. 35Explanation
The radicand is the number under the radical symbol. In this case, the radical symbol is the cube root (∛) and the number under it is 35. Therefore, the radicand is 35.Rate this question:
-
- 22.
The logarithm of unity to any base is:
-
1
-
10
-
E
-
0
Correct Answer
A. 0Explanation
The logarithm of unity to any base is 0 because any number raised to the power of 0 is always equal to 1. This means that the logarithm of 1 to any base will always be 0.Rate this question:
-
- 23.
The relation y=logzx implies
-
Xy=z
-
Zy=x
-
Xz=y
-
Yz=x
Correct Answer
A. Zy=xExplanation
The relation y=logzx implies that taking the logarithm of z to the base x will give us y. In other words, if we raise x to the power of y, we will get z. Therefore, zy=x is the correct answer.Rate this question:
-
- 24.
Log e = __________, where e ≈ 2.718
-
0
-
0.4343
-
∞
-
1
Correct Answer
A. 0.4343Explanation
The value of the natural logarithm of e is approximately 0.4343. The natural logarithm is the logarithm to the base e, where e is a mathematical constant approximately equal to 2.718. Therefore, log e = 0.4343.Rate this question:
-
- 25.
If ax=n, then:
-
A=logxa
-
X=logna
-
X=logan
-
A=lognx
Correct Answer
A. X=loganExplanation
The equation ax=n can be rewritten as x=logan. This is because the logarithm of a number to a base is the exponent to which the base must be raised to obtain that number. In this case, "a" is the base, "x" is the exponent, and "n" is the result of raising "a" to the power of "x". Therefore, x=logan is the correct answer.Rate this question:
-
- 26.
The logaritm of any number to itself as base is ___________
-
1
-
0
-
-1
-
10
Correct Answer
A. 1Explanation
The logarithm of any number to itself as a base is always 1. This is because the logarithm of a number to a base is the exponent to which the base must be raised to obtain the number. When the number and the base are the same, the exponent is always 1.Rate this question:
-
- 27.
Antilogarithm table was prepared by
-
John Napier
-
Henry Briggs
-
Jobst Burgi
-
Arthur Cayley
Correct Answer
A. Jobst BurgiExplanation
Jobst Burgi is the correct answer because he was a Swiss mathematician who worked closely with John Napier and Henry Briggs in the development of logarithms. While Napier is credited with the invention of logarithms, Burgi played a significant role in their practical application and calculation. He collaborated with Napier and Briggs to create logarithm tables, which were used for various mathematical calculations. Therefore, Burgi's contribution to the preparation of antilogarithm tables makes him the correct answer for this question.Rate this question:
-
- 28.
Log p - log q is same as _____________
-
Log(p/q)
-
Log(p-q)
-
Log p/log q
-
Log(q/p)
Correct Answer
A. Log(p/q)Explanation
The given expression, log p - log q, is equivalent to log(p/q). This is because when subtracting logarithms with the same base, the result is the logarithm of the division of the corresponding values. Therefore, log p - log q simplifies to log(p/q).Rate this question:
-
- 29.
Log (mn) can be written as ________________
-
(log m)n
-
M log n
-
N log m
-
Log (mn)
Correct Answer
A. N log mExplanation
The given expression, log (mn), can be written as n log m. This is because when we take the logarithm of a product, it can be split into the sum of the logarithms of the individual factors. So, log (mn) is equal to log m + log n. By rearranging the terms, we get n log m.Rate this question:
-
- 30.
4x+3y-2 is an algebraic
-
Expression
-
Sentence
-
Equation
-
Inequation
Correct Answer
A. ExpressionExplanation
The given expression, 4x+3y-2, is a combination of variables (x and y) and constants (4, 3, and 2) connected by mathematical operations (+ and -). It does not have an equal sign, so it is not an equation. It also does not have any inequality symbols (such as > orRate this question:
-
- 31.
The degree of polynomial 4x4+2x2y is ____________
-
1
-
2
-
3
-
4
Correct Answer
A. 4Explanation
The degree of a polynomial is determined by the highest power of the variable in the polynomial. In this case, the polynomial is 4x^4 + 2x^2y. The highest power of x is 4, which means the degree of the polynomial is 4.Rate this question:
-
- 32.
(√a+√b)(√a-√b) is equal to
-
A2+b2
-
A2-b2
-
A-b
-
A+b
Correct Answer
A. A-bExplanation
The expression (√a+√b)(√a-√b) can be simplified using the difference of squares formula, which states that (x+y)(x-y) is equal to x^2 - y^2. In this case, we can let x = √a and y = √b. Therefore, the expression simplifies to (√a)^2 - (√b)^2, which is equal to a - b.Rate this question:
-
- 33.
A2-b2 /a+b
-
(a-b)2
-
(a+b)2
-
(a-b)
-
(a+b)
Correct Answer
A. (a-b)Explanation
The correct answer is (a-b) because when we simplify the expression (a2-b2)/(a+b), we can factor the numerator as (a-b)(a+b). Then, we can cancel out the (a+b) terms in the numerator and denominator, leaving us with (a-b) as the final answer.Rate this question:
-
- 34.
1/a-b - 1/a+b
-
2a/a2-b2
-
2b/a2-b2
-
-2a/a2-b2
-
-2b/a2-b2
Correct Answer
A. 2b/a2-b2Explanation
The given expression can be simplified by finding the common denominator, which is (a^2 - b^2). By multiplying the first term (1/a - b) by (a + b)/(a + b), we get (a + b)/(a^2 - b^2). Simplifying further, we have (2b)/(a^2 - b^2), which matches the given answer 2b/a^2 - b^2.Rate this question:
-
- 35.
If x=3 and y=-1 then the value of x3y is
-
27
-
-27
-
9
-
-9
Correct Answer
A. -27Explanation
The given expression x3y means x cubed multiplied by y. Substituting the given values, we have 3 cubed multiplied by -1, which equals -27.Rate this question:
-
- 36.
The factors of x2-5x+6 are
-
X+1, x-6
-
X-2, x-3
-
X+6, x-1
-
X+2, x+3
Correct Answer
A. X-2, x-3Explanation
The given quadratic equation is x^2 - 5x + 6. To find the factors of this equation, we need to determine two binomials that multiply together to give the original equation. By using the method of factorization, we can determine that the factors are (x-2) and (x-3).Rate this question:
-
- 37.
Factors of a4-4b4 are ____________
-
(a-b),(a+b),(a2+4b2)
-
(a2-2b2), (a2+2b2)
-
(a-b),(a+b),(a2-4b2)
-
(a-2b),(a2+2b2)
Correct Answer
A. (a2-2b2), (a2+2b2)Explanation
The given expression is a^4 - 4b^4. This can be factored using the difference of squares formula, which states that a^2 - b^2 = (a - b)(a + b). In this case, we can rewrite the expression as (a^2)^2 - (2b^2)^2, which can be factored as (a^2 - 2b^2)(a^2 + 2b^2). Therefore, the factors of a^4 - 4b^4 are (a^2 - 2b^2) and (a^2 + 2b^2).Rate this question:
-
- 38.
What will be added to complete the square of 9a2-12ab?
-
-16b2
-
16b2
-
4b2
-
-4b2
Correct Answer
A. 4b2Explanation
To complete the square of 9a^2 - 12ab, we need to add a term that will make the expression a perfect square trinomial. The coefficient of the middle term is -12ab, so we want to find a term that, when squared, will give us (12ab/2)^2 = (6ab)^2 = 36a^2b^2. This term is 36a^2b^2. However, since we want to add this term to the expression, we need to subtract it to maintain the equality. Therefore, the term that needs to be added to complete the square is -36a^2b^2. Simplifying, we get 9a^2 - 12ab - 36a^2b^2. Factoring out a common factor, we have (3a - 6ab)^2. Notice that the middle term is -12ab, which matches the original expression. Therefore, the correct answer is 4b^2.Rate this question:
-
- 39.
Factors of 3x2-x-2 are ____________
-
(x+1),(3x-2)
-
(x+1),(3x+2)
-
(x-1),(3x-2)
-
(x-1),(3x+2)
Correct Answer
A. (x-1),(3x+2)Explanation
The factors of a quadratic expression can be found by factoring it into two binomials. In this case, we need to find two binomials that when multiplied together, result in 3x^2-x-2. By trial and error or using the quadratic formula, we can determine that the correct factors are (x-1) and (3x+2). Multiplying these two binomials together will give us the original quadratic expression.Rate this question:
-
- 40.
Factors of 5x2-17xy-12y2 are ______________
-
(x+4y),(5x+3y)
-
(x-4y),(5x-3y)
-
(x-4y),(5x+3y)
-
(5x-4y),(x+3y)
Correct Answer
A. (x-4y),(5x+3y)Explanation
The given expression is a quadratic expression in two variables, x and y. To find the factors, we can use the factoring method. The expression can be factored as (x-4y)(5x+3y). This means that when we multiply these two factors, we will get the original expression. Therefore, the correct answer is (x-4y),(5x+3y).Rate this question:
-
- 41.
Find m so that x2+4x+m is a complete square _____________
-
8
-
-8
-
4
-
16
Correct Answer
A. 4Explanation
To find m so that x^2 + 4x + m is a complete square, we can use the formula for completing the square. The formula states that if we have a quadratic equation in the form ax^2 + bx + c, then we can rewrite it as (x + (b/2a))^2 + (c - b^2/4a). In this case, a = 1, b = 4, and c = m. Plugging these values into the formula, we get (x + 2)^2 + (m - 4)/4. To make this a complete square, we want the second term to be equal to zero. Therefore, (m - 4)/4 = 0, which implies m = 4.Rate this question:
-
- 42.
If (x-2) is a factor of P(x) = x2+2kx+8, then the value of k is _____________
-
3
-
-3
-
2
-
-2
Correct Answer
A. -3Explanation
If (x-2) is a factor of P(x), it means that when we substitute x=2 into P(x), the result will be zero. Therefore, we can substitute x=2 into P(x) and solve for k. By substituting x=2 into P(x)=x^2+2kx+8, we get 2^2+2k(2)+8=0. Simplifying this equation, we get 4+4k+8=0. Combining like terms, we have 4k+12=0. Subtracting 12 from both sides, we get 4k=-12. Dividing both sides by 4, we find that k=-3.Rate this question:
-
- 43.
L.C.M of 15x2, 45xy and 30xyz is ____________
-
90xyz
-
90x2yz
-
15xyz
-
15x2yz
Correct Answer
A. 90x2yzExplanation
The L.C.M (Least Common Multiple) of three numbers is the smallest number that is divisible by each of the given numbers. To find the L.C.M of 15x^2, 45xy, and 30xyz, we need to find the highest power of each variable (x, y, and z) that appears in any of the given numbers. In this case, the highest power of x is 2, the highest power of y is 1, and the highest power of z is 1. Therefore, the L.C.M is 90x^2yz.Rate this question:
-
- 44.
H.C.F of a3+b3 and a2-ab+b2 is _____________
-
A+b
-
A2-ab+b2
-
(a-b)2
-
A2+b2
Correct Answer
A. A2-ab+b2Explanation
The given question asks for the highest common factor (H.C.F) of two expressions, a^3 + b^3 and a^2 - ab + b^2. The correct answer, a^2 - ab + b^2, is obtained by factoring the expressions and finding the common factors. The expressions can be factored as (a + b)(a^2 - ab + b^2) and (a + b)(a^2 - ab + b^2) respectively. The common factor is (a + b), and when simplified, we get a^2 - ab + b^2. Therefore, a^2 - ab + b^2 is the H.C.F of the given expressions.Rate this question:
-
- 45.
H.C.F of 5x2y2 and 20x3y3 is _____________
-
5x2y2
-
20x3y3
-
100x5y5
-
5xy
Correct Answer
A. 5x2y2Explanation
The H.C.F (Highest Common Factor) of two numbers is the largest number that divides both of them without leaving a remainder. In this case, the given numbers are 5x^2y^2 and 20x^3y^3. To find the H.C.F, we need to find the highest power of each variable that is common to both numbers. The highest power of x that is common to both numbers is x^2, and the highest power of y that is common to both numbers is y^2. Therefore, the H.C.F is 5x^2y^2.Rate this question:
-
- 46.
The square root of a2-2a+1 is
-
±(a+1)
-
±(a-1)
-
A-1
-
A+1
Correct Answer
A. ±(a-1)Explanation
The square root of a^2 - 2a + 1 can be written as √(a-1)^2. Taking the square root of a perfect square gives us the positive and negative value of the square root. Therefore, the answer is ±(a-1).Rate this question:
-
- 47.
The square root of x2-1+1/4x2 is __________
-
±(x-1/2x)
-
±(x+1/2x)
-
(x-1/2x)
-
√(x-1/2x)
Correct Answer
A. ±(x-1/2x)Explanation
The given expression is the square root of x^2 - 1 + 1/4x^2. By factoring out a common factor of 1/4 from the two terms inside the square root, we get (1/4)(4x^2 - 1 + x^2). Simplifying further, we have (1/4)(5x^2 - 1). Taking the square root of this expression gives us ±√(1/4)(5x^2 - 1), which simplifies to ±√(5x^2 - 1)/2. Rearranging the terms, we get ±(x - 1/2x), which matches the given answer.Rate this question:
-
- 48.
L.C.M of a2+b2 and a4-b4 is :
-
A2+b2
-
A2-b2
-
A4-b4
-
A-b
Correct Answer
A. A4-b4Explanation
The L.C.M (Least Common Multiple) of a2+b2 and a4-b4 can be found by factoring both expressions. When we factor a2+b2, it cannot be further simplified. However, when we factor a4-b4, we can use the difference of squares formula to simplify it as (a2+b2)(a2-b2). Therefore, the L.C.M of a2+b2 and a4-b4 is a4-b4.Rate this question:
-
- 49.
H.C.F of x-2 and x2+x-6 is ________________
-
X2+x-6
-
X+3
-
X-2
-
X+2
Correct Answer
A. X-2Explanation
The given question asks for the highest common factor (H.C.F) of the expressions x-2 and x^2+x-6. To find the H.C.F, we need to factorize both expressions. The expression x^2+x-6 can be factored as (x+3)(x-2). The common factor between x-2 and (x+3)(x-2) is x-2. Therefore, the correct answer is x-2.Rate this question:
-
Quiz Review Timeline (Updated): Mar 20, 2023 +
Our quizzes are rigorously reviewed, monitored and continuously updated by our expert board to maintain accuracy, relevance, and timeliness.
-
Current Version
-
Mar 20, 2023Quiz Edited by
ProProfs Editorial Team -
Mar 29, 2020Quiz Created by
Waqar Hussain
 Back to top
Back to top