Test Algebra Mid-Chapter 4 Sections 1-4
(187).webp "Test Algebra Mid-Chapter 4 Sections 1-4 - Quiz")
.
Questions and Answers
- 1.
Write an equation of a line in slope-intercept form with the given slope and y-intercept.Slope 1/4; y-intercept 3
- A.
Y = 1/4x
- B.
Y = 1/4x + 3
- C.
Y = 3x + 1/4
- D.
X = 1/4y + 3
Correct Answer
B. Y = 1/4x + 3Explanation
The equation of a line in slope-intercept form is y = mx + b, where m is the slope and b is the y-intercept. In this case, the slope is 1/4 and the y-intercept is 3. Therefore, the equation of the line is y = 1/4x + 3.Rate this question:
-
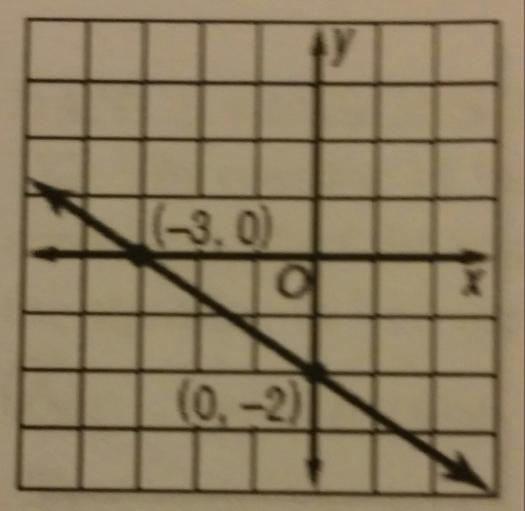
- 2.
Write an equation in slope-intercept form for the graph given.
- A.
![Test Algebra Mid-Chapter 4 Sections 1-4 - Quiz]()
- B.
![Test Algebra Mid-Chapter 4 Sections 1-4 - Quiz]()
- C.
![Test Algebra Mid-Chapter 4 Sections 1-4 - Quiz]()
- D.
![Test Algebra Mid-Chapter 4 Sections 1-4 - Quiz]()
Correct Answer
C. -
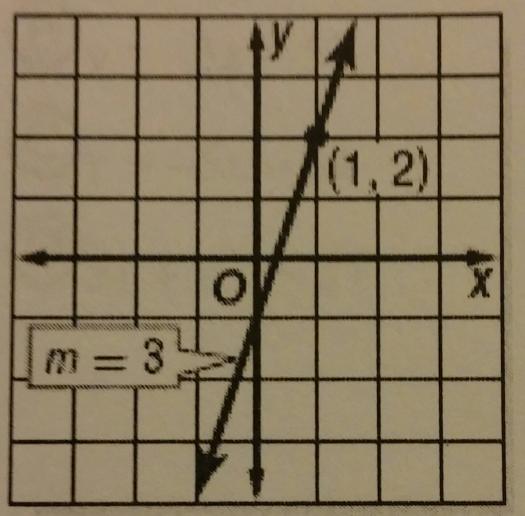
- 3.
Write an equation in slope intercept for the graph given.
- A.
![Test Algebra Mid-Chapter 4 Sections 1-4 - Quiz]()
- B.
![Test Algebra Mid-Chapter 4 Sections 1-4 - Quiz]()
- C.
![Test Algebra Mid-Chapter 4 Sections 1-4 - Quiz]()
- D.
![Test Algebra Mid-Chapter 4 Sections 1-4 - Quiz]()
Correct Answer
D. -
- 4.
Write an equation of the line that passes through the given point with the given slope.
- A.
Y = 3x - 1
- B.
Y = 3x + 1
- C.
Y = -x + 3
- D.
Y = -3x + 1
Correct Answer
A. Y = 3x - 1Explanation
The equation of a line can be written in the form y = mx + b, where m is the slope of the line and b is the y-intercept. In the given equation, y = 3x - 1, the slope is 3. Therefore, the equation y = 3x - 1 represents a line that passes through a given point with a slope of 3.Rate this question:
-
- 5.
Write an equation of the line that passes through the given point with the given slope.(-5, 4); slope -3
- A.
Y = -3x + 11
- B.
Y = -3x - 11
- C.
Y = -3x + 19
- D.
Y = -11x - 3
Correct Answer
B. Y = -3x - 11Explanation
The equation of a line can be written in the form y = mx + b, where m is the slope and b is the y-intercept. In this case, the given point is (-5, 4) and the slope is -3. Plugging these values into the equation, we get y = -3x - 11. This equation represents a line that passes through the point (-5, 4) and has a slope of -3.Rate this question:
-
- 6.
Write an equation of the line that passes through each pair of points.(0, -4), (5, -4)
- A.
Y = -4
- B.
Y = 4
- C.
Y = -4x
- D.
Y = 0
Correct Answer
A. Y = -4Explanation
The equation y = -4 represents a horizontal line passing through the y-coordinate -4. Since both points (0, -4) and (5, -4) have the same y-coordinate, the line that passes through them will be a horizontal line at y = -4.Rate this question:
-
- 7.
The cost for 7 dance lessons is $82. The cost or 11 lessons is $122. Write a linear equation to find the total cost C for n lessons. Then use the equation to find the cost of 4 lessons.
- A.
C = 12n + 10; $58
- B.
C = 10n + 12; $52
- C.
C = 12n ; $48
- D.
C = 10n; $40
Correct Answer
B. C = 10n + 12; $52Explanation
The linear equation to find the total cost C for n lessons is C = 10n + 12. This equation is derived by observing that the cost increases by $10 for each additional lesson, and there is an additional fixed cost of $12.
To find the cost of 4 lessons, we substitute n = 4 into the equation: C = 10(4) + 12 = $40 + $12 = $52. Therefore, the cost of 4 lessons is $52.Rate this question:
-
- 8.
Write an equation in point slope form, , for the line that passes through the point (2, 2) and has the slope -3.
- A.
Y - 2 = 3(x - 2)
- B.
Y + 2 = -3(x + 2)
- C.
Y - 2 = -3(x - 2)
- D.
Y = -3x + 8
Correct Answer
C. Y - 2 = -3(x - 2)Explanation
The equation y - 2 = -3(x - 2) is the correct answer. It is in point-slope form, which is y - y1 = m(x - x1), where (x1, y1) is a point on the line and m is the slope. In this case, the point (2, 2) is on the line and the slope is -3. Plugging in these values into the equation gives y - 2 = -3(x - 2).Rate this question:
-
- 9.
Write an equation in point-slope form for the line that passes through the point (-3, -4) with the slope of 0.
- A.
Y = 4
- B.
Y - 4 = 0
- C.
Y + 4 = 0
- D.
Y + 3 = 0
Correct Answer
C. Y + 4 = 0Explanation
The equation y + 4 = 0 is the correct answer. In point-slope form, the equation is y - y₁ = m(x - x₁), where (x₁, y₁) is the given point and m is the slope. In this case, the given point is (-3, -4) and the slope is 0. Plugging in these values into the equation gives us y - (-4) = 0(x - (-3)), which simplifies to y + 4 = 0.Rate this question:
-
- 10.
Write the equation in standard form, Ax + By = C.
- A.
3x - y = -5
- B.
Y = 3x + 5
- C.
-3x + y = 5
- D.
3x + y = -5
Correct Answer
A. 3x - y = -5Explanation
The given equation is 3x - y = -5. To write it in standard form, we need to rearrange the terms so that the x and y variables are on the left side and the constant term is on the right side. Therefore, the equation can be written as 3x - y + 5 = 0. This is the standard form, Ax + By = C, where A = 3, B = -1, and C = 5.Rate this question:
-
- 11.
Write the equation in slope-intercept form, y = mx + b.
- A.
Y = -5x
- B.
Y = -5x - 57
- C.
Y = -5x - 63
- D.
5x + y = - 57
Correct Answer
B. Y = -5x - 57Explanation
The equation y = -5x - 57 is in slope-intercept form, y = mx + b, where m is the slope (-5) and b is the y-intercept (-57). This means that the graph of this equation is a straight line with a slope of -5 and crosses the y-axis at -57.Rate this question:
-
- 12.
Write an equation in slope intercept form for the line that passes through the given point and is parallel to the graph of the given equation.(3, 2), y = x + 5
- A.
Y = -x - 1
- B.
Y = x - 1
- C.
Y = x + 1
- D.
Y = x - 5
Correct Answer
B. Y = x - 1Explanation
To find an equation in slope-intercept form for a line parallel to y = x + 5, we need to use the same slope. The slope of y = x + 5 is 1. Therefore, the equation of the parallel line will also have a slope of 1. Since the line passes through the point (3, 2), we can use the point-slope form of a linear equation to write the equation as y - 2 = 1(x - 3). Simplifying this equation gives us y - 2 = x - 3. Rearranging the equation to the slope-intercept form, we get y = x - 1.Rate this question:
-
- 13.
Write an equation in slope intercept form for the line that passes through the given point and is parallel to the graph of the given equation.(-3, 4), 3y = 2x - 3
- A.
![Test Algebra Mid-Chapter 4 Sections 1-4 - Quiz]()
- B.
![Test Algebra Mid-Chapter 4 Sections 1-4 - Quiz]()
- C.
![Test Algebra Mid-Chapter 4 Sections 1-4 - Quiz]()
- D.
![Test Algebra Mid-Chapter 4 Sections 1-4 - Quiz]()
Correct Answer
D.Explanation
To find the equation of a line that is parallel to the given equation, we need to determine the slope of the given line. By rearranging the equation, we can see that the slope of the given line is 2/3. Since the parallel line has the same slope, we can plug in the coordinates of the given point (-3, 4) and the slope (2/3) into the slope-intercept form equation y = mx + b. Solving for b, we find that b = 10/3. Therefore, the equation of the line that passes through (-3, 4) and is parallel to 3y = 2x - 3 is y = (2/3)x + 10/3.Rate this question:
-
- 14.
Quadrilateral ABCD has diagonals and . Determine whether is perpendicular to . Explain.
- A.
Yes; they are perpendicular because their slopes are 7 and , which are negative reciprocals.
![Test Algebra Mid-Chapter 4 Sections 1-4 - Quiz]()
- B.
Yes; they are perpendicular because their slopes are 7 and -7 which are negative reciprocals.
- C.
No; they are parallel because their slopes are 7 and , which are negative reciprocals.
![Test Algebra Mid-Chapter 4 Sections 1-4 - Quiz]()
- D.
No; they are parallel because the slopes are both 7.
Correct Answer
A. Yes; they are perpendicular because their slopes are 7 and , which are negative reciprocals.Explanation
The given answer is correct. The slopes of the diagonals are 7 and -7, which are negative reciprocals of each other. In geometry, two lines are perpendicular if and only if their slopes are negative reciprocals of each other. Therefore, the diagonals in quadrilateral ABCD are perpendicular to each other.Rate this question:
-
- 15.
Write an equation in slope-intercept form for the line that passes through the given point and is perpendicular to the graph of the given equation.(-6, -5), 4x + 3y = -6
- A.
![Test Algebra Mid-Chapter 4 Sections 1-4 - Quiz]()
- B.
![Test Algebra Mid-Chapter 4 Sections 1-4 - Quiz]()
- C.
![Test Algebra Mid-Chapter 4 Sections 1-4 - Quiz]()
- D.
![Test Algebra Mid-Chapter 4 Sections 1-4 - Quiz]()
Correct Answer
C.Explanation
The given equation is in standard form, so we need to rearrange it to slope-intercept form (y = mx + b) to find the slope. We can do this by isolating y: 3y = -4x - 6, y = (-4/3)x - 2. The slope of the given equation is -4/3. Since the line we want to find is perpendicular to this line, the slope of the new line will be the negative reciprocal of -4/3, which is 3/4. Using the point-slope form (y - y1 = m(x - x1)), we can substitute the point (-6, -5) and the slope 3/4 into the equation to get y + 5 = (3/4)(x + 6). Simplifying further, we get y = (3/4)x + 9/2.Rate this question:
-
- 16.
Carla has already written 10 pages of a novel. She plans to write 15 additional pages per month until she is finished.________ A) Write an equation to find the total number of pages P written after any number of months m. (answer should be in the form P = mm + b)________ B) Graph the equation on a separate grid. (Type the word GRID in all caps for the answer)________ C) Find the total number of pages written after 5 months. (Enter the number only)
Correct Answer
P = 15m + 10
GRID
85Explanation
The equation P = 15m + 10 represents the total number of pages written after any number of months. The variable m represents the number of months, and the constant 10 represents the initial 10 pages that Carla has already written. Each month, Carla writes an additional 15 pages, which is represented by the coefficient 15.
The word "GRID" indicates that a graph should be created to represent the equation.
To find the total number of pages written after 5 months, we can substitute m = 5 into the equation: P = 15(5) + 10 = 85. Therefore, the total number of pages written after 5 months is 85.Rate this question:
- 17.
A construction company charges $15 per hour for debris removal, plus a one-time fee for the use of a trash dumpster. The total fee for 9 hours of service is $195.________ A) Write the point slope form of an equation to find the total fee y for any number of hours x.________ B) Write the equation in slope intercept form. y = mx + b________ C) What is the fee for the use of a trash dumpster. (Enter ONLY a number)
Correct Answer
y - 195 = 15(x - 9)
y = 15x + 60
60Explanation
The given equation y - 195 = 15(x - 9) represents the point-slope form of an equation to find the total fee y for any number of hours x. It shows that the total fee y is equal to $15 per hour multiplied by the number of hours x, minus the one-time fee for the use of a trash dumpster, which is $195. The equation y = 15x + 60 is the slope-intercept form of the same equation, where the slope (m) is 15 and the y-intercept (b) is 60. Therefore, the fee for the use of a trash dumpster is $60.Rate this question:
Quiz Review Timeline +
Our quizzes are rigorously reviewed, monitored and continuously updated by our expert board to maintain accuracy, relevance, and timeliness.
-
Current Version
-
Jan 22, 2024Quiz Edited by
ProProfs Editorial Team -
Dec 06, 2015Quiz Created by
Pcrumpton
- Calculus Quizzes
- Data Handling Quizzes
- Decimal Quizzes
- Discrete Mathematics Quizzes
- Function Quizzes
- Geometry Quizzes
- Graph Quizzes
- Measurement Quizzes
- Multiplication Quizzes
- Number Quizzes
- Percentage Quizzes
- Place Value Quizzes
- Probability Quizzes
- Problem Solving Quizzes
- Ratio Quizzes
- Shape Quizzes
- Statistics Quizzes
- Trigonometry Quizzes
Back to top