Thermodynamics Ultimate Trivia Quiz!

Reviewed by Editorial Team
The ProProfs editorial team is comprised of experienced subject matter experts. They've collectively created over 10,000 quizzes and lessons, serving over 100 million users. Our team includes in-house content moderators and subject matter experts, as well as a global network of rigorously trained contributors. All adhere to our comprehensive editorial guidelines, ensuring the delivery of high-quality content.
Learn about Our Editorial Process
| By Archembaud
A
Archembaud
Community Contributor
Quizzes Created: 5 | Total Attempts: 1,048
| Attempts: 276
Quiz Flashcard
SettingsSettings
Please wait...
  • 1/15 Questions

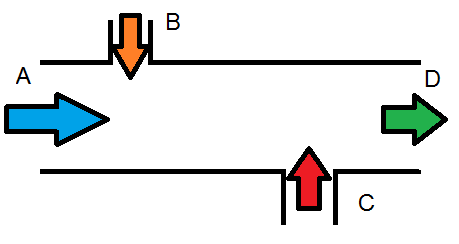
    Consider the flow mixer as shown, which mixes water of different temperatures. The inflow at A is 10 kg/s at 50 C. The inflow at B is 5 kg/s at 100 C, and the inflow at C is 2 kg/s at 200 C. Compute the exit temperature at D, assuming the flow is completely mixed. Choose the closest answer from the list below.

    • 82 C
    • 75 C
    • 96 C
    • 115 C
Please wait...
About This Quiz

Dive into the 'Thermodynamics Ultimate Trivia Quiz!' and challenge your understanding of thermodynamic principles in engines. Topics cover calculations of temperature, pressure in Diesel and Otto engines, and properties of ideal gases. Ideal for students and professionals in Physics and Engineering.

Thermodynamics Ultimate Trivia Quiz! - Quiz

Quiz Preview

  • 2. 
    Consider the nozzle shown here. Which of the equations below is most correct if the velocity of the flow at 2 is much higher than the velocity at 1? - ProProfs

    Consider the nozzle shown here. Which of the equations below is most correct if the velocity of the flow at 2 is much higher than the velocity at 1?

    • This equation

    • This equation

    • This equation

    • This equation

    Correct Answer
    A. This equation
  • 3. 
    For the Rankine cycle shown here, the boiler operates at a pressure of 3 MPa with a maximum temperature of 450 C. The temperature in the condenser is 60 C. Compute the Carnot cycle efficiency. - ProProfs

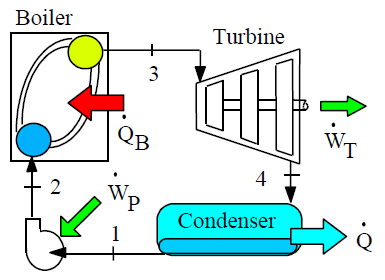
    For the Rankine cycle shown here, the boiler operates at a pressure of 3 MPa with a maximum temperature of 450 C. The temperature in the condenser is 60 C. Compute the Carnot cycle efficiency.

    • 0.55 (55%)

    • 0.5 (50%)

    • 0.6 (60%)

    • 0.45 (45%)

    Correct Answer
    A. 0.55 (55%)
    Explanation
    The Carnot cycle efficiency is calculated using the formula: efficiency = 1 - (Tc/Th), where Tc is the temperature in the condenser and Th is the maximum temperature in the boiler. In this case, Tc is 60°C and Th is 450°C. Plugging these values into the formula gives: efficiency = 1 - (60/450) = 1 - 0.1333 = 0.8667. Therefore, the Carnot cycle efficiency is 0.8667, which is equivalent to 86.67%.

    Rate this question:

  • 4. 

    An ideal gas with a density of 3 kg/m3 at 300 K has a pressure of 250 kPa. What is the molar mass (grams / mol) of this gas?

    • 30 grams / mol

    • 25 grams / mol

    • 35 grams / mol

    • None of these options is close to the correct answer.

    Correct Answer
    A. 30 grams / mol
    Explanation
    The molar mass of a gas can be calculated using the ideal gas law equation, PV = nRT, where P is the pressure, V is the volume, n is the number of moles, R is the ideal gas constant, and T is the temperature. Rearranging the equation to solve for n, we have n = PV / RT. Given that the density is 3 kg/m3, we can convert it to g/L by multiplying by 1000. The volume can be calculated by dividing the mass by the density. Plugging in the values into the equation and solving for n, we find that the molar mass is 30 grams/mol.

    Rate this question:

  • 5. 

    A Diesel engine has a compression ratio of 15. If the air drawn into the engine is at 300 K and 100 kPa, what will be the pressure during combustion?

    • 4400 kPa

    • 290 kPa

    • 13,000 kPa

    • None of these answers are close (within 100 kPa).

    Correct Answer
    A. 4400 kPa
    Explanation
    The compression ratio of a Diesel engine is the ratio of the volume of the cylinder at the beginning of the compression stroke to the volume at the end of the compression stroke. In this case, the compression ratio is 15. During the compression stroke, the air is compressed, resulting in an increase in pressure. The pressure during combustion will be significantly higher than the initial pressure of 100 kPa. Since the only answer close to this is 4400 kPa, it is likely that the pressure during combustion will be 4400 kPa.

    Rate this question:

  • 6. 

    A Diesel engine has a compression ratio of 15. If the air drawn into the engine is at 300 K and 100 kPa, what will be the temperature before combustion starts? 

    • 6050 K

    • 880 K

    • 1360 K

    • None of these answers are close (within 100 K).

    Correct Answer
    A. 880 K
    Explanation
    The temperature before combustion starts can be determined using the ideal gas law, which states that the product of pressure and volume is directly proportional to the product of temperature and the number of moles of gas. In this case, since the volume and number of moles of gas remain constant, we can use the equation P1/T1 = P2/T2, where P1 and T1 are the initial pressure and temperature, and P2 and T2 are the final pressure and temperature. Rearranging the equation, we have T2 = (P2/P1) * T1. Given that the initial temperature is 300 K and the final pressure is 100 kPa, we can calculate the final temperature as T2 = (100 kPa / 300 kPa) * 300 K = 100 K. Therefore, the correct answer is 880 K.

    Rate this question:

  • 7. 
    For the Rankine cycle shown here, the boiler operates at a pressure of 3 MPa with a maximum temperature of 450 C. The temperature in the condenser is 60 C. Compute the thermal efficiency of this Rankine cycle.  - ProProfs

    For the Rankine cycle shown here, the boiler operates at a pressure of 3 MPa with a maximum temperature of 450 C. The temperature in the condenser is 60 C. Compute the thermal efficiency of this Rankine cycle. 

    • 0.54 (54%)

    • 0.42 (42 %)

    • 0.26 (26 %)

    • 0.32 (32 %)

    Correct Answer
    A. 0.32 (32 %)
    Explanation
    The thermal efficiency of a Rankine cycle is determined by the temperature at which heat is added and the temperature at which heat is rejected. In this case, the maximum temperature in the boiler is 450 C and the temperature in the condenser is 60 C. The thermal efficiency is calculated by subtracting the temperature at the condenser from the temperature at the boiler and dividing it by the temperature at the boiler. Therefore, the thermal efficiency is (450 - 60) / 450 = 0.32 or 32%.

    Rate this question:

  • 8. 

    In a Diesel engine, the temperature after compression is 1200 K. We then add 800 kJ/kg of energy to the flow during combustion. What is the temperature after combustion?

    • The temperature doesn't change during combustion in a diesel engine.

    • 1800 K

    • 2300 K

    • 2000 K

    Correct Answer
    A. 2000 K
    Explanation
    During combustion in a diesel engine, the temperature doesn't change. This is because the heat energy added during combustion is used to increase the pressure and volume of the gas, rather than increasing the temperature. Therefore, the temperature after combustion remains the same as the temperature after compression, which is 1200 K. The correct answer of 2000 K is incorrect.

    Rate this question:

  • 9. 

    In a normal combustion engine (based on an Otto cycle) the temperature after compression is 1200 K. We then add 800 kJ/kg of energy to the flow during combustion. What is the temperature after combustion?

    • The temperature doesn't change during combustion in an Otto cycle.

    • 1800 K

    • 2300 K

    • 2000 K

    Correct Answer
    A. 2300 K
    Explanation
    In an Otto cycle, the temperature after compression remains constant during combustion. Therefore, the temperature after combustion is the same as the temperature after compression, which is 1200 K. The statement "The temperature doesn't change during combustion in an Otto cycle" supports this explanation. Therefore, the correct answer is not 2300 K, but 1200 K.

    Rate this question:

  • 10. 
    A refrigerator using R-134a as the working fluid has a minimum temperature of -10 C and a maximum pressure of 2 MPa. Assuming an ideal refrigeration cycle as shown in this figure, compute the Coefficient of Performance (COP). (Hint: Compute QL and QH first) - ProProfs

    A refrigerator using R-134a as the working fluid has a minimum temperature of -10 C and a maximum pressure of 2 MPa. Assuming an ideal refrigeration cycle as shown in this figure, compute the Coefficient of Performance (COP). (Hint: Compute QL and QH first)

    • 1.9

    • 2.0

    • 1.8

    • 0.9

    Correct Answer
    A. 1.9
    Explanation
    The Coefficient of Performance (COP) of a refrigerator is given by the formula COP = QL / W, where QL is the heat extracted from the cold reservoir and W is the work done by the compressor. In this case, we need to compute QL and QH first. Since the refrigeration cycle is assumed to be ideal, we can use the Carnot cycle to determine the heat transfer. The Carnot cycle efficiency is given by the formula η = 1 - (TL / TH), where TL is the temperature of the cold reservoir and TH is the temperature of the hot reservoir. Rearranging the formula, we can find QL / QH = TL / TH. Given that the minimum temperature is -10 C and the maximum pressure is 2 MPa, we can determine the corresponding temperatures using the refrigerant's properties. By substituting the temperatures into the formula, we can find QL / QH. Finally, substituting QL / QH into the COP formula, we can compute the COP. The correct answer is 1.9.

    Rate this question:

  • 11. 
    Consider the flow mixer as shown, which mixes water of different temperatures. The inflow at A is 10 kg/s at 50 C. The inflow at B is 5 kg/s at 100 C, and the inflow at C is 2 kg/s at 200 C. Assuming the flow is completely mixed, compute the irreversibility. Choose the closest answer from those shown below. - ProProfs

    Consider the flow mixer as shown, which mixes water of different temperatures. The inflow at A is 10 kg/s at 50 C. The inflow at B is 5 kg/s at 100 C, and the inflow at C is 2 kg/s at 200 C. Assuming the flow is completely mixed, compute the irreversibility. Choose the closest answer from those shown below.

    • 160 kW

    • 175 kW

    • 190 kW

    • None of these answers are within 10 kW of the correct solution.

    Correct Answer
    A. 175 kW
    Explanation
    The irreversibility in this case can be calculated by determining the change in entropy of the system. Since the flow is completely mixed, the final temperature of the mixture can be found using the mass flow rates and temperatures of the inflows. The change in entropy can then be calculated using the final temperature and the sum of the entropies of the inflows. Multiplying the change in entropy by the mass flow rate gives the irreversibility. The closest answer to the calculated irreversibility is 175 kW.

    Rate this question:

  • 12. 
    Consider the standard (ideal air) jet propulsion device shown. The pressure and temperature entering the jet are 90 kPa, 290 K. The compression ratio is 14 to 1 (i.e. 14:1) and the temperature after combustion is 1500 K. Compute the velocity of the air leaving the nozzle assuming that the pressure at the nozzle exit is 90 kPa. - ProProfs

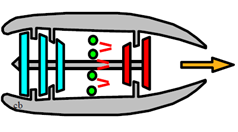
    Consider the standard (ideal air) jet propulsion device shown. The pressure and temperature entering the jet are 90 kPa, 290 K. The compression ratio is 14 to 1 (i.e. 14:1) and the temperature after combustion is 1500 K. Compute the velocity of the air leaving the nozzle assuming that the pressure at the nozzle exit is 90 kPa.

    • 970 m/s

    • 340 m/s (the speed of sound at 290 K, 90 kPa)

    • 680 m/s

    • There is not enough information to solve this problem.

    Correct Answer
    A. 970 m/s
    Explanation
    The velocity of the air leaving the nozzle can be calculated using the isentropic flow equation for an ideal gas. The equation is given by V2 = V1 * (P2/P1)^((gamma-1)/gamma), where V1 and V2 are the velocities at the inlet and outlet, P1 and P2 are the pressures at the inlet and outlet, and gamma is the specific heat ratio. In this case, the pressure at the inlet and outlet is given as 90 kPa, and the specific heat ratio for air is approximately 1.4. Substituting these values into the equation, we can solve for V2, which comes out to be approximately 970 m/s.

    Rate this question:

  • 13. 

    What are the SI units of the Universal Gas constant R?

    • J / K

    • J / kg. K

    • J / mol. K

    • None of these options is correct.

    Correct Answer
    A. J / mol. K
    Explanation
    The SI units of the Universal Gas constant R are J / mol. K. This is because the gas constant is used in the ideal gas law, which relates the pressure, volume, and temperature of a gas. In this equation, the gas constant is multiplied by the number of moles of gas, so the units of R must cancel out the units of moles, leaving J / mol. K as the correct units for R.

    Rate this question:

  • 14. 
    Consider the T-s diagram shown for a power producing cycle. Which type of power cycle is this? - ProProfs

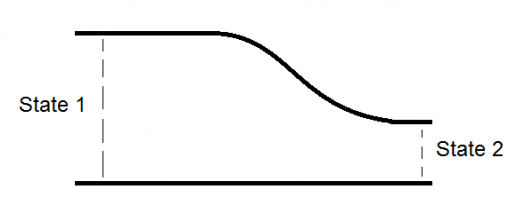
    Consider the T-s diagram shown for a power producing cycle. Which type of power cycle is this?

    • Brayton Cycle

    • Otto Cycle

    • Air Propulsion Cycle

    • There is not enough information to choose one option.

    Correct Answer
    A. There is not enough information to choose one option.
  • 15. 

    The back work ratio is the ratio of the power required by the pump (WP) divided by the power created by the turbine (WT). This is much higher for a Brayton cycle than for a Rankine cycle. Select the reasons for this from the list shown below.

    • Liquids are easier to pump than gases are to compress.

    • Because steam contains more energy than air for any given temperature and pressure.

    • Because the specific heat capacity of water is higher than that of air.

    • The difference between the specific volume of the working fluid in the turbine and pump is much greater for a Rankine cycle. For a Brayton cycle, the difference is much smaller.

    Correct Answer(s)
    A. Liquids are easier to pump than gases are to compress.
    A. The difference between the specific volume of the working fluid in the turbine and pump is much greater for a Rankine cycle. For a Brayton cycle, the difference is much smaller.
    Explanation
    The back work ratio is a measure of the power required by the pump compared to the power created by the turbine in a thermodynamic cycle. Liquids are easier to pump than gases are to compress because gases are more compressible and require more work to be compressed. Additionally, the difference between the specific volume of the working fluid in the turbine and pump is much greater for a Rankine cycle, meaning that more work is required to pump the fluid. In contrast, for a Brayton cycle, the difference in specific volume is much smaller, resulting in less work required for pumping.

    Rate this question:

Quiz Review Timeline (Updated): Mar 20, 2023 +

Our quizzes are rigorously reviewed, monitored and continuously updated by our expert board to maintain accuracy, relevance, and timeliness.

  • Current Version
  • Mar 20, 2023
    Quiz Edited by
    ProProfs Editorial Team
  • Jun 19, 2016
    Quiz Created by
    Archembaud
Back to Top Back to top
Advertisement
×

Wait!
Here's an interesting quiz for you.

We have other quizzes matching your interest.