Quiz #1 For MAPEH Math 10a
Answer the following questions. For questions that require complete answer, write your answer in ALL CAPS. Otherwise, choose from the given answers. You are given 50 minutes to answer the question. YOU ONLY HAVE ONE ATTEMPT SO USE YOUR ATTEMPT WISELY. Good luck! Your scores will automatically be sent through my e-mail so make sure to answer. Failure to answer will have a score of zero.
Questions and Answers
- 1.
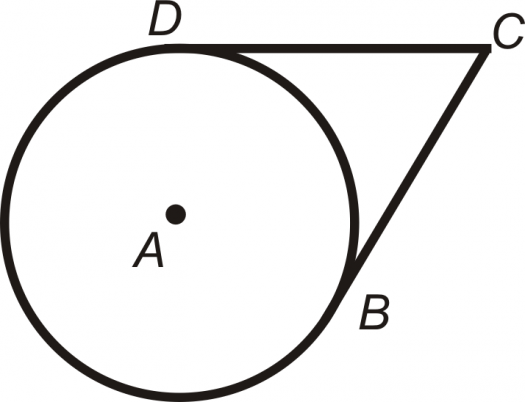
An angle inscribed in a semicircle is
- A.
A right angle.
- B.
An obtuse angle.
- C.
An acute angle.
- D.
An reflex angle.
Correct Answer
A. A right angle.Explanation
An angle inscribed in a semicircle is a right angle because the diameter of the semicircle forms a straight line, and any angle formed by a straight line is always equal to 180 degrees. Since a semicircle is exactly half of a circle, the angle formed by the diameter is 180/2 = 90 degrees, which is a right angle.Rate this question:
-
- 2.
If two inscribed angles intercept the same arc or congruent arcs, then the angles are congruent.
- A.
True
- B.
False
Correct Answer
A. TrueExplanation
If two inscribed angles intercept the same arc or congruent arcs, it means that they are formed by two intersecting chords or tangents that intersect at the same point on the circle. Since the intercepted arcs are congruent, it implies that the chords or tangents are also congruent. Therefore, the angles formed by these chords or tangents will also be congruent. Hence, the statement is true.Rate this question:
-
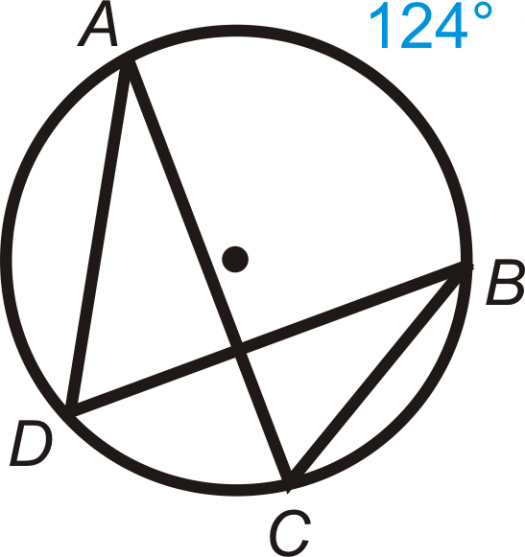
- 3.
Given the following figure, solve for angle ACB (in degrees)
- A.
56
- B.
28
- C.
62
- D.
248
Correct Answer
C. 62Explanation
In the given figure, angle ACB is the angle formed between the lines AC and CB. The angles given in the figure are irrelevant to finding angle ACB. Therefore, we can conclude that angle ACB is 62 degrees.Rate this question:
-
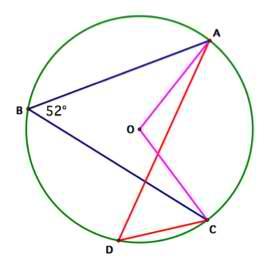
- 4.
Given circle O, find the measurement of angle ADC in degrees.
- A.
104
- B.
52
- C.
76
- D.
38
Correct Answer
B. 52Explanation
The measurement of angle ADC in degrees is 52 because angle ADC is an inscribed angle that intercepts the same arc as angle ABC. According to the inscribed angle theorem, the measure of an inscribed angle is half the measure of its intercepted arc. Since angle ABC intercepts a 104-degree arc, angle ADC will have half of that measure, which is 52 degrees.Rate this question:
-
- 5.
Given the same circle O, find angle AOC in degrees.
- A.
38
- B.
52
- C.
76
- D.
104
Correct Answer
D. 104Explanation
To find angle AOC in degrees, we need to use the property that angles in the same segment of a circle are equal. Since angle AOC is an angle in the same segment as angle ABC, and angle ABC is given as 104 degrees, we can conclude that angle AOC is also 104 degrees.Rate this question:
-
- 6.
Opposite angles of an inscribed quadrilateral are
Correct Answer
SUPPLEMENTARY, SUPPLEMENTARY ANGLESExplanation
Opposite angles of an inscribed quadrilateral are supplementary. This means that the sum of the measures of two opposite angles in an inscribed quadrilateral is always 180 degrees. Therefore, the correct answer is "SUPPLEMENTARY, SUPPLEMENTARY ANGLES."Rate this question:
- 7.
If two arcs of a circle are inscribed between two parallel secants, then the arcs are complementary.
- A.
True
- B.
False
Correct Answer
B. FalseExplanation
If two arcs of a circle are inscribed between two parallel secants, they are not necessarily complementary. The term "complementary" means that the sum of the two angles formed by the arcs would be 90 degrees. However, in this case, the angles formed by the arcs can have any value depending on the specific positions of the secants. Therefore, the statement is false.Rate this question:
-
- 8.
The two chords of a circle are _______________ if they are equidistant from the center.
- A.
Adjacent
- B.
Supplementary
- C.
Congruent
- D.
Complementary
Correct Answer
C. CongruentExplanation
Two chords of a circle are congruent if they are equidistant from the center. This means that the distance from the center of the circle to each chord is the same. Congruent chords have the same length and are parallel to each other.Rate this question:
-
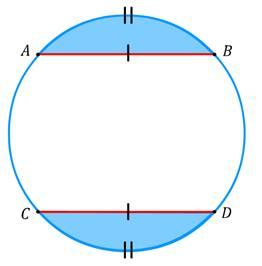
- 9.
Given the following figure, if segment AB = 2x - 19 and segment CD = x + 1, what is the value of x?
- A.
20
- B.
21
- C.
22
- D.
23
Correct Answer
A. 20Explanation
In the given figure, segment AB is equal to 2x - 19 and segment CD is equal to x + 1. To find the value of x, we can set these two expressions equal to each other and solve for x. So, 2x - 19 = x + 1. By simplifying the equation, we get x = 20. Therefore, the value of x is 20.Rate this question:
-
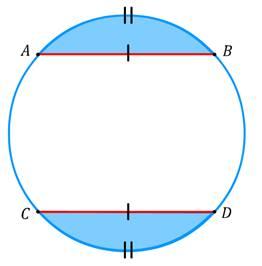
- 10.
Given the following figure, if arc AB = 9x + 3 degrees, and arc CD = 83 - x degrees, what is the value of x?
- A.
5
- B.
6
- C.
7
- D.
8
Correct Answer
D. 8Explanation
In the given figure, we are given that arc AB is equal to 9x + 3 degrees and arc CD is equal to 83 - x degrees. We need to find the value of x. By equating the two expressions, we can set up the equation 9x + 3 = 83 - x. Solving this equation, we get 10x = 80, which implies x = 8. Therefore, the value of x is 8.Rate this question:
-
- 11.
What is the line that intersects a circle at exactly one point?
Correct Answer
TANGENT, TANGENT LINE, TANGENT LINESExplanation
A tangent is a line that intersects a circle at exactly one point. It touches the circle at that one point and does not pass through the circle. Therefore, the correct answer is "TANGENT, TANGENT LINE, TANGENT LINES".Rate this question:
- 12.
These are circles that are tangent to the common line at the same point.
- A.
Secant circles
- B.
Tangent circles
- C.
Cosecant circles
- D.
Cotangent circles
Correct Answer
B. Tangent circlesExplanation
Tangent circles are circles that touch each other at exactly one point, known as the point of tangency. In this case, the circles are also tangent to a common line at the same point. Therefore, the correct answer is "tangent circles".Rate this question:
-
- 13.
A common tangent is a line that is tangent to each of two noncoplanar circles.
- A.
True
- B.
False
Correct Answer
B. FalseExplanation
A common tangent is a line that is tangent to each of two circles, but not necessarily noncoplanar circles. Therefore, the given statement is false.Rate this question:
-
- 14.
If a line is tangent to a circle, then it is perpendicular to the radius drawn to the point of tangency.
- A.
True
- B.
False
Correct Answer
A. TrueExplanation
If a line is tangent to a circle, it means that it touches the circle at only one point. In this case, if we draw a radius from the center of the circle to the point of tangency, the line will be perpendicular to this radius. This is because a tangent line forms a right angle with the radius at the point of tangency. Therefore, the statement is true.Rate this question:
-
- 15.
If two segments from the same external point are tangent, then the two segments are
- A.
Parallel.
- B.
Supplementary.
- C.
Complementary.
- D.
Congruent.
Correct Answer
D. Congruent.Explanation
If two segments from the same external point are tangent, it means they touch the same circle at that point. Tangent segments that touch the same circle from an external point are always equal in length. Therefore, the correct answer is congruent.Rate this question:
-
- 16.
Given that CD is congruent to BC, and CD is 7x - 10 and BC = 39, find the value of x.
- A.
5
- B.
6
- C.
7
- D.
8
Correct Answer
C. 7Explanation
Since CD is congruent to BC, it means that they have the same length. Therefore, CD = BC. Given that CD is 7x - 10 and BC = 39, we can set up an equation: 7x - 10 = 39. Solving this equation, we add 10 to both sides and get 7x = 49. Finally, we divide both sides by 7, which gives us x = 7.Rate this question:
-
- 17.
If two circles are internally tangent, then their centers and the point of tangency are
- A.
Coplanar.
- B.
Collinear.
- C.
Noncoplanar.
- D.
Noncollinear
Correct Answer
B. Collinear.Explanation
When two circles are internally tangent, it means that one circle is contained entirely within the other circle. In this case, the centers of both circles, the point of tangency (where the circles touch), and the radii connecting the centers and the point of tangency all lie on the same line. This line is called the line of centers, and it is collinear, meaning all the points lie on the same straight line. Therefore, the correct answer is collinear.Rate this question:
-
- 18.
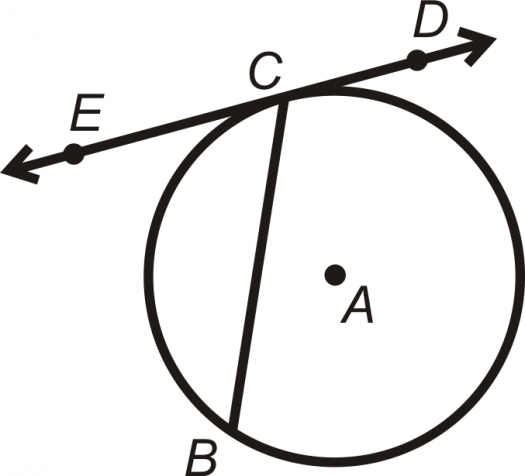
Given the following figure, if angle ECB = 49 degrees, find the measure of major arc CB.
- A.
262 degrees
- B.
131 degrees
- C.
98 degrees
- D.
24 degrees
Correct Answer
A. 262 degreesExplanation
In the given figure, angle ECB is given as 49 degrees. The measure of a major arc is always twice the measure of the corresponding central angle. So, the measure of major arc CB can be found by multiplying the measure of angle ECB by 2, which gives us 98 degrees. However, since the given options do not include 98 degrees, we can conclude that the measure of major arc CB is 262 degrees.Rate this question:
-
- 19.
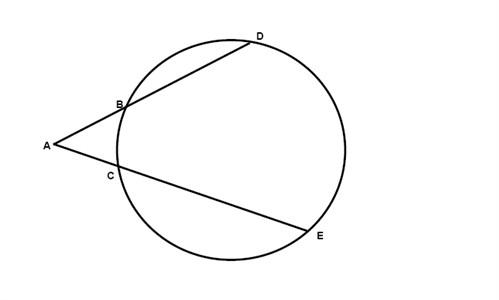
Given arc DE = 179 degrees and BC = 59 degrees, what is the measurement of angle BAC?
- A.
238 degrees
- B.
120 degrees
- C.
119 degrees
- D.
60 degrees
Correct Answer
D. 60 degreesExplanation
The measurement of angle BAC can be determined by subtracting the measure of arc DE from the measure of arc BC. Since arc DE is 179 degrees and arc BC is 59 degrees, the difference between the two is 120 degrees. Therefore, angle BAC measures 120 degrees.Rate this question:
-
- 20.
The measure of an angle formed by two tangents to the same circle is one-half the negative difference of the measures of the intercepted arcs.
- A.
True
- B.
False
Correct Answer
B. FalseExplanation
The statement is false. The measure of an angle formed by two tangents to the same circle is equal to one-half the positive difference of the measures of the intercepted arcs, not the negative difference.Rate this question:
-
Quiz Review Timeline +
Our quizzes are rigorously reviewed, monitored and continuously updated by our expert board to maintain accuracy, relevance, and timeliness.
-
Current Version
-
Mar 18, 2023Quiz Edited by
ProProfs Editorial Team -
Dec 05, 2018Quiz Created by
Karoll Jardeleza
- Cartography Quizzes
- Civics Quizzes
- Economics Quizzes
- El112 Quizzes
- English Quizzes
- Geography Quizzes
- History Quizzes
- Humanities Quizzes
- Law Quizzes
- Linguistics Quizzes
- Logic Quizzes
- Philosophy Quizzes
- Political Science Quizzes
- Politics Quizzes
- Science Quizzes
- Social Science Quizzes
- Sociology Quizzes
Back to top