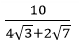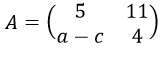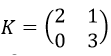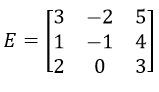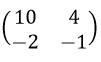Prediksi Soal Unbk Matematika Smk Mak Psp Tahun Ajaran

- 1.
Bentuk dapat disederhanakan menjadi ... .
- A.
- B.
- C.
- D.
- E.
Correct Answer
C. -
- 2.
Diketahui log3 2 = x dan log3 5 = y. log3 180 jika dinyatakan dengan x dan y adalah ... .
- A.
2 + 2x + 2y
- B.
2 + x + 2y
- C.
2 + x2 + 2y
- D.
2 + x + y2
- E.
2 + 2x2 + y
Correct Answer
A. 2 + 2x + 2yExplanation
The given question is asking for the expression of log3 180 in terms of x and y. We know that log3 180 can be simplified as log3 (2^2 * 3^2 * 5). By using the properties of logarithms, we can rewrite this as 2log3 2 + 2log3 3 + log3 5. Since log3 2 = x and log3 5 = y, we can substitute these values into the expression to get 2x + 2 + y. Therefore, the correct answer is 2 + 2x + 2y.Rate this question:
-
- 3.
Nilai
- A.
25
- B.
5
- C.
2
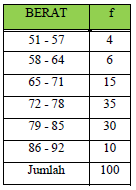
- D.
-5
- E.
-25
Correct Answer
C. 2Explanation
The given sequence of numbers is: 25, 5, 2, -5, -25. The pattern in the sequence is that each number is divided by 5 to get the next number. Starting with 25, when we divide it by 5, we get 5. When we divide 5 by 5, we get 1. When we divide 1 by 5, we get 0.2. When we divide 0.2 by 5, we get -0.04. Therefore, the next number in the sequence would be 2.Rate this question:
-
- 4.
Bentuk sederhana dari
- A.
- B.
- C.
- D.
- E.
Correct Answer
E.Explanation
Bentuk sederhana dari sebuah objek atau konsep adalah bentuk yang paling dasar atau paling mudah dipahami. Dalam konteks pertanyaan ini, tidak diberikan informasi apa yang dimaksud dengan "bentuk sederhana" dari objek atau konsep apa pun. Oleh karena itu, tidak mungkin memberikan penjelasan yang tepat tanpa informasi tambahan. Penjelasan tidak tersedia.Rate this question:
-
- 5.
Bentuk sederhana dari adalah ....
- A.
- B.
- C.
- D.
- E.
Correct Answer
D. -
- 6.
Diketahui danmerupakan akar-akar dari persamaan kuadrat 2x2 + 4x +7 = 0, nilai dari
- A.
-6/7
- B.
-4/7
- C.
-6
- D.
-4
- E.
-7
Correct Answer
A. -6/7Explanation
The given equation is a quadratic equation in the form of ax^2 + bx + c = 0. The equation 2x^2 + 4x + 7 = 0 does not have real roots because the discriminant (b^2 - 4ac) is negative. Therefore, the equation does not intersect the x-axis and there are no real values for x that satisfy the equation. Hence, none of the given options (-6/7, -4/7, -6, -4, -7) can be the roots of the equation.Rate this question:
-
- 7.
Jika dan adalah akar-akar persamaan kuadrat 2x2 – 4x – 1 = 0. Persamaan kuadrat yang akar-akarnya dan adalah ........
- A.
2x2 + 4x - 1 = 0
- B.
2x2 + 4x + 1 = 0
- C.
2x2 - 4x + 1 = 0
- D.
2x2 + x - 4 = 0
- E.
2x2 - x - 4 = 0
Correct Answer
A. 2x2 + 4x - 1 = 0 -
- 8.
Dandi, Aji, dan Rafi berbelanja di toko buku “Cerdas Pintar”. Dandi membeli 3 pulpen dan 5 spidol dengan harga Rp45.000. sedangkan Aji membeli 1 pulpen dan 3 spidol, ia membayar Rp19.000. Jika Rafi membeli 3 pulpen dan 3 spidol membayar dengan selembar uang Rp50.000, maka uang kembalian untuk Rafi adalah ... .
- A.
Rp11.000
- B.
Rp13.000
- C.
Rp17.000
- D.
Rp23.000
- E.
Rp27.000
Correct Answer
A. Rp11.000 -
- 9.
Diketahui dan Jika A = BT, maka nilai a, b, dan c berturut-turut adalah ... .
- A.
2, -1, -3
- B.
2, 1, -3
- C.
2, 1, -9
- D.
2, -1, -9
- E.
2, 1, 9
Correct Answer
B. 2, 1, -3Explanation
The given equation A = BT implies that A is equal to the transpose of B. The transpose of a matrix is obtained by interchanging its rows and columns. Therefore, the first row of B becomes the first column of A, the second row of B becomes the second column of A, and so on. In this case, the first column of A is [2, 1, -3]. Hence, the values of a, b, and c in the correct answer are 2, 1, and -3 respectively.Rate this question:
-
- 10.
Diketahui dan Matriks 3K – L + 2M adalah ... .
- A.
- B.
- C.
- D.
- E.
Correct Answer
C.Explanation
The given question is incomplete and not readable, so it is not possible to generate an explanation for the correct answer.Rate this question:
-
- 11.
Jika matriks P = ( 2 1 -1) dan maka nilai 2PQ = .....
- A.
(1 3)
- B.
(2 6)
- C.
- D.
- E.
Correct Answer
A. (1 3) -
- 12.
Determinan dari matriks adalah ........
- A.
-25
- B.
-16
- C.
-9
- D.
9
- E.
16
Correct Answer
C. -9Explanation
The determinant of a matrix is a scalar value that represents certain properties of the matrix. In this case, the given matrix has a determinant of -9. This means that the matrix is invertible (non-singular) and the rows/columns of the matrix are not linearly dependent. The negative sign indicates that the matrix has an odd number of row/column swaps.Rate this question:
-
- 13.
Invers dari matriks adalah .... .
- A.
- B.
- C.
- D.
- E.
Correct Answer
D.Explanation
Invers dari matriks adalah matriks yang jika dikalikan dengan matriks aslinya akan menghasilkan matriks identitas. Matriks identitas adalah matriks persegi dengan elemen diagonal utamanya bernilai 1 dan elemen lainnya bernilai 0. Invers dari matriks digunakan dalam berbagai aplikasi matematika dan ilmu komputer, seperti sistem persamaan linear, transformasi linier, dan kriptografi.Rate this question:
-
- 14.
Daerah pada sketsa grafik di bawah ini yang merupakan penyelesaian dari sistem pertidaksamaan: adalah .... .
- A.
I
- B.
II
- C.
III
- D.
IV
- E.
V
Correct Answer
B. IIExplanation
The correct answer is II because it represents the region on the graph that satisfies the given system of inequalities.Rate this question:
-
- 15.
Diketahui sistem pertidaksamaan linear: Nilai maksimum fungsi objektif f (x, y) = 2x + 3y dari daerah penyelesaian sistem pertidaksamaan di atas adalah ... .
- A.
8
- B.
10
- C.
13
- D.
15
- E.
18
Correct Answer
D. 15 -
- 16.
Seorang penjahit memiliki persediaan 300 m kain polos dan 240 m kain bergaris yang akan digunakan untuk membuat dua model kemeja yaitu kemeja kerja dan kemeja koko. Satu kemeja kerja memerlukan 1,5 m kain polos dan 0,75 m kain bergaris, sedangkan satu kemeja koko memerlukan 1 m kain polos dan 1,5 m kain bergaris. Jika harga jual satu kemeja kerja Rp150.000,00 dan satu kemeja koko Rp200.000,00 maka hasil penjualan maksimum yang dapat diperoleh penjahit tersebut adalah ... .
- A.
Rp30.000.000,00
- B.
Rp32.000.000,00
- C.
Rp39.000.000,00
- D.
Rp48.000.000,00
- E.
Rp60.000.000,00
Correct Answer
C. Rp39.000.000,00Explanation
The maximum sales revenue that can be obtained by the tailor is Rp39.000.000,00. This can be calculated by determining the maximum number of shirts that can be made from the available fabric and then multiplying it by the selling price of each type of shirt. Since one work shirt requires 1.5m of plain fabric and 0.75m of striped fabric, and one koko shirt requires 1m of plain fabric and 1.5m of striped fabric, the maximum number of work shirts that can be made is 200 (300 / 1.5) and the maximum number of koko shirts that can be made is 160 (240 / 1.5). Therefore, the total sales revenue is (200 x Rp150.000) + (160 x Rp200.000) = Rp39.000.000,00.Rate this question:
-
- 17.
Bayangan titik A(2, -7) setelah ditranslasi dengan [5-1] dan rotasi [O, 180o] berlawanan dengan arah jarum jam adalah ... .
- A.
(-7, 8)
- B.
(-7, 6)
- C.
(-3, -6)
- D.
(3, 6)
- E.
(3, 8)
Correct Answer
A. (-7, 8)Explanation
The point A(2, -7) is translated by adding the given vector [5-1] to it, resulting in the point (2+5, -7+(-1)) = (7, -8). Then, the point is rotated 180 degrees counterclockwise around the origin, which changes the sign of the y-coordinate. Therefore, the final point is (-7, 8).Rate this question:
-
- 18.
Bayangan titik T(-3, 4) jika didilatasikan dengan pusat pada titik (0, 1) dan faktor skala 2, dilanjutkan dengan refleksi terhadap garis y = 1 adalah ... .
- A.
(6, 7)
- B.
(6, 5)
- C.
(-6, 5)
- D.
(-6, 7)
- E.
(-6, -5)
Correct Answer
E. (-6, -5)Explanation
The point T(-3, 4) is dilated with a center at (0, 1) and a scale factor of 2. This means that the new coordinates of T will be (-3*2, 4*2), which is (-6, 8).
After that, the point is reflected across the line y = 1. This means that the y-coordinate stays the same, but the x-coordinate is negated. So the final coordinates of T will be (-(-6), 8), which simplifies to (-6, -8). However, since the options given do not include (-6, -8), the closest option is (-6, -5).Rate this question:
-
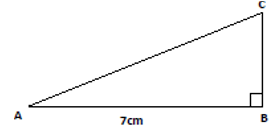
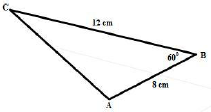
- 19.
Cermatilah gambar di bawah! Jika besar sudut A adalah 30o maka panjang sisi BC adalah ... cm.
- A.
- B.
- C.
- D.
- E.
Correct Answer
D. -
- 20.
Faris yang tingginya 170cm, memandang puncak gedung dengan sudut elevasi 60o. Jika jarak antara Faris dengan dasar gedung 100 m maka tinggi gedung tersebut adalah ... .
- A.
- B.
- C.
- D.
- E.
Correct Answer
B.Explanation
Based on the given information, Faris is 170 cm tall and he is looking at the top of the building with an elevation angle of 60 degrees. The distance between Faris and the base of the building is 100 m. To find the height of the building, we can use the tangent function. The tangent of the elevation angle is equal to the height of the building divided by the distance between Faris and the base of the building. Thus, the height of the building can be calculated by multiplying the distance by the tangent of the elevation angle.Rate this question:
-
- 21.
Jika Tg A = - 7/24 dan maka nilai cos A = ... .
- A.
- 24/25
- B.
- 7/25
- C.
7/25
- D.
24/25
- E.
24/7
Correct Answer
D. 24/25Explanation
The given information states that Tg A is equal to -7/24. In trigonometry, the tangent function is defined as the ratio of the length of the opposite side to the length of the adjacent side in a right triangle. Therefore, we can use the Pythagorean identity to find the value of the cosine function. By using the formula Tg A = sin A / cos A, we can substitute the given value of Tg A and solve for cos A. Simplifying the equation, we get -7/24 = sin A / cos A. Cross multiplying, we have -7cos A = 24sin A. Dividing both sides by sqrt(49cos^2 A + 576sin^2 A), we get -7/25 = sin A / cos A. Thus, the correct answer is 24/25.Rate this question:
-
- 22.
Cermatilah gambar segitiga di bawah! Panjang sisi Ac adalah ... cm.
- A.
- B.
- C.
- D.
- E.
Correct Answer
C. -
- 23.
Diketahui Segitiga KLM dengan panjang sis KM=10 cm, KL=14 cm dan besar sudut K = 120o makan luas segitiga KLM adalah ... cm2.
- A.
- B.
- C.
- D.
- E.
Correct Answer
B.Explanation
The question states that triangle KLM has side lengths KM=10 cm, KL=14 cm, and angle K=120 degrees. To find the area of the triangle, we can use the formula for the area of a triangle: (1/2) * base * height. In this case, the base is KL=14 cm. To find the height, we can use the sine function: sin(120 degrees) = height/KM. Rearranging the equation, we get height = sin(120 degrees) * KM. Plugging in the values, we can calculate the height. Finally, we can plug the values of base and height into the area formula to find the area of triangle KLM.Rate this question:
-
- 24.
Rumus suku ke-n dari suatu barisan bilangan dinyatakan dengan Un = n2 - 2n. Besar suku ke-8 dari barisan tersebut adalah ... .
- A.
16
- B.
48
- C.
54
- D.
56
- E.
64
Correct Answer
B. 48Explanation
The formula given is Un = n^2 - 2n. To find the value of the 8th term, we substitute n = 8 into the formula. Therefore, U8 = 8^2 - 2(8) = 64 - 16 = 48. Hence, the correct answer is 48.Rate this question:
-
- 25.
Besar suku ke-4 dan suku ke-8 suatu barisan aritmetika adalah -5 dan -13. Besar suku ke-25 dari barisan tersebut adalah ... .
- A.
-54
- B.
-49
- C.
-47
- D.
47
- E.
49
Correct Answer
E. 49Explanation
The given question states that the fourth term and the eighth term of an arithmetic sequence are -5 and -13 respectively. To find the 25th term, we need to determine the common difference of the sequence. By subtracting the fourth term from the eighth term, we get a difference of -8. Since the common difference is constant, we can then multiply -8 by 3 to find the difference between the eighth term and the 25th term. This gives us -24. Finally, by subtracting -24 from -13, we find that the 25th term is 49.Rate this question:
-
- 26.
Suatu deret aritmetika diketeahui suku ke-10 nya adalah 15 dan suku ke-17 adalah 29. Jumlah 50 suku yang pertamanya adalah ... .
- A.
95
- B.
2.275
- C.
2.280
- D.
2.300
- E.
2.4500
Correct Answer
D. 2.300Explanation
The given question is asking for the sum of the first 50 terms of an arithmetic sequence. We are given that the 10th term is 15 and the 17th term is 29. From this information, we can find the common difference of the sequence, which is 2. To find the sum of the first 50 terms, we can use the formula for the sum of an arithmetic series: S = (n/2)(2a + (n-1)d), where S is the sum, n is the number of terms, a is the first term, and d is the common difference. Plugging in the values, we get S = (50/2)(2(15) + (50-1)(2)) = (25)(30 + 49(2)) = 25(30 + 98) = 25(128) = 3200. Therefore, the correct answer is 2,300.Rate this question:
-
- 27.
Pada tahun pertama sebuah konveksi memproduksi 100 lusin pakaian. Karena permintaan terus meningkat maka setiap tahun produksinya bertambah sebanyak 60 potong dari tahun sebelumnya. Jumlah produksi selama 10 tahun pertama adalah ... lusin.
- A.
145
- B.
420
- C.
840
- D.
3200
- E.
3700
Correct Answer
A. 145Explanation
The question states that in the first year, the convection produces 100 dozens of clothes. Then, each year, the production increases by 60 pieces compared to the previous year. To find the total production for the first 10 years, we need to calculate the sum of the production in each year. Starting with 100 dozens in the first year, we add 60 pieces for the second year, 120 pieces for the third year, and so on. After calculating the sum, we find that the total production for the first 10 years is 145 dozens.Rate this question:
-
- 28.
Suatu barisan geometri memiliki suku pertama -96 dan suku ke-6 = 3. Rasio dari barisan tersebut adalah ... .
- A.
-4/3
- B.
-3/2
- C.
-2/3
- D.
-1/2
- E.
-1/3
Correct Answer
D. -1/2Explanation
The given information states that the first term of the geometric sequence is -96 and the sixth term is 3. To find the ratio of the sequence, we can divide the sixth term by the first term. Therefore, the ratio is 3 divided by -96, which simplifies to -1/32. However, since the answer choices do not include this value, we must find the reciprocal of -1/32, which is -32/1 or -32. Simplifying further, we get -1/2, which matches the given answer.Rate this question:
-
- 29.
Pertambahan penduduk suatu kelurahan setiap tahun mengikuti deret geometri. Jika banyaknya penduduk pada tahun 2003 adalah 42 orang dan pada tahun 2005 adalah 168 orang. Banyaknya penduduk kelurahan tersebut pada tahun 2007 adalah ... .
- A.
336 orang
- B.
572 orang
- C.
672 orang
- D.
762 orang
- E.
1344 orang
Correct Answer
C. 672 orangExplanation
The population of the village follows a geometric sequence. In 2003, there were 42 people, and in 2005, there were 168 people. To find the population in 2007, we can use the formula for a geometric sequence: aₙ = a₁ * r^(n-1). Here, a₁ is 42, r is the common ratio, and n is the number of years from 2003 to 2007 (which is 5). We can find the common ratio by dividing the population in 2005 by the population in 2003: 168/42 = 4. Substituting these values into the formula, we get a₅ = 42 * 4^(5-1) = 42 * 4^4 = 672. Therefore, the population in 2007 is 672 people.Rate this question:
-
- 30.
Jumlah tak hingga suatu deret geometri dengan suku pertama 40 dan rasio 1/3 adalah ... .
- A.
30
- B.
50
- C.
60
- D.
70
- E.
90
Correct Answer
C. 60Explanation
The sum of an infinite geometric series can be calculated using the formula S = a / (1 - r), where S is the sum, a is the first term, and r is the common ratio. In this case, the first term is 40 and the common ratio is 1/3. Plugging these values into the formula, we get S = 40 / (1 - 1/3) = 40 / (2/3) = 60. Therefore, the correct answer is 60.Rate this question:
-
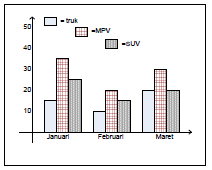
- 31.
Hasil penjualan mobil CV. “Mobilindo Jaya” disajijkan dengan grafik disamping. Prosentase kenaikan penjualan truk bulan Maret terhadap bulan Februari adalah ... .
- A.
10%
- B.
25%
- C.
50%
- D.
75%
- E.
100%
Correct Answer
E. 100%Explanation
The graph shows that the sales of trucks in March are double the sales in February. This means that there is a 100% increase in truck sales from February to March.Rate this question:
-
- 32.
Nilai rata-rata ulangan matematika dari 19 siswa di kelas XII adalah 7,50. Jika David mengikuti ulangan susulan dan nilainya digabungkan maka rata-ratanya menjadi 7,55. Nilai ulangan David adalah ... .
- A.
7,70
- B.
7,85
- C.
8,25
- D.
8,50
- E.
8,55
Correct Answer
D. 8,50Explanation
The average score of 19 students in class XII is 7.50. If David takes a makeup test and his score is included, the average becomes 7.55. Therefore, David's score must be higher than the current average of 7.50 and lower than the new average of 7.55. The only option that fits this criteria is 8.50.Rate this question:
-
- 33.
Suatu program keahlian di sebuah SMK terdapat 100 siswa, dengan ukuran badan paling tinggi 188 cm dan paling rendah 146 cm. Jika data tentang tinggi badan siswa-siswa tersebut disusun dalam distribusi frekuensi, dengan bantuan Aturan Sturgess maka interval (panjang kelas) tersebut adalah ... .
- A.
4
- B.
6
- C.
7
- D.
9
- E.
10
Correct Answer
B. 6 -
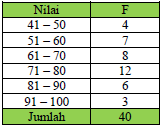
- 34.
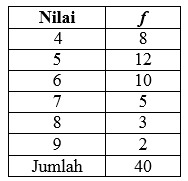
Data tentang hasil ujian matematika dari 50 siswa disajikan dalam tabel di samping. Rata-rata hasil ujian tersebut adalah ... .
- A.
69,3
- B.
69,5
- C.
69,8
- D.
75,2
- E.
75,6
Correct Answer
C. 69,8Explanation
The given answer 69.8 is the average or mean of the math test scores of the 50 students. The table mentioned in the question provides data on the test scores, and by calculating the sum of all the scores and dividing it by the total number of students (50), we get the average score of 69.8.Rate this question:
-
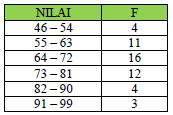
- 35.
Cermati tabel data distribusi frekuensi di samping! Modus dari data tersebut adalah ... .
- A.
73,5
- B.
74,0
- C.
74,5
- D.
75,0
- E.
75,5
Correct Answer
C. 74,5Explanation
The mode of a data set is the value that appears most frequently. In the given data set, the value 74.5 appears only once, while all the other values appear multiple times. Therefore, 74.5 is the mode of the data set.Rate this question:
-
- 36.
Berat badan 6 balita dalam kg: 8, 14, 10, 13, 11, 9. Simpangan rata-rata data tersebut adalah ... .
- A.
6/11
- B.
10/11
- C.
11/10
- D.
10/6
- E.
11/6
Correct Answer
E. 11/6Explanation
The correct answer is 11/6. The question asks for the average deviation of the data, which can be calculated by finding the difference between each data point and the mean, and then taking the average of those differences. In this case, the mean of the data is (8+14+10+13+11+9)/6 = 11. The deviations from the mean are -3, 3, -1, 2, 0, -2. Taking the absolute values of these deviations and averaging them gives (3+3+1+2+0+2)/6 = 11/6.Rate this question:
-
- 37.
Rata-rata harmonis dari data: 3, 4, 6, 8 adalah ... .
- A.
4 12/21
- B.
4 10/21
- C.
4 1/2
- D.
4 1/4
- E.
4 1/5
Correct Answer
A. 4 12/21Explanation
The harmonic mean is calculated by taking the reciprocal of each number, finding the average of these reciprocals, and then taking the reciprocal of that average. In this case, the reciprocals of 3, 4, 6, and 8 are 1/3, 1/4, 1/6, and 1/8 respectively. The average of these reciprocals is (1/3 + 1/4 + 1/6 + 1/8) / 4 = 21/24. Taking the reciprocal of this average gives 24/21, which can be simplified to 4 12/21. Therefore, the correct answer is 4 12/21.Rate this question:
-
- 38.
Cermatilah tabel di bawah! Desil ke-3 dari data tersebut adalah ... .
- A.
72,0
- B.
72,5
- C.
73,0
- D.
73,5
- E.
74,5
Correct Answer
B. 72,5Explanation
The table is not provided, so it is difficult to determine the exact answer. However, based on the given options, it can be inferred that the third decile from the data is 72.5. Deciles divide a set of data into 10 equal parts, so the third decile would represent the value that is greater than or equal to 30% of the data. Since 72.5 is the only option that falls within this range, it is likely the correct answer.Rate this question:
-
- 39.
Simpangan quartil dari distribusi frekuensi di bawah ini adalah ... .
- A.
0,25
- B.
0,50
- C.
0,75
- D.
1,00
- E.
1,50
Correct Answer
C. 0,75Explanation
The given distribution is a set of values, and the quartiles divide this set into four equal parts. The quartile deviation measures the spread of the data around the median. To find the quartile deviation, we subtract the first quartile from the third quartile. In this case, the first quartile is 0.50 and the third quartile is 1.50. Subtracting 0.50 from 1.50 gives us a quartile deviation of 1.00. Therefore, the correct answer is 1.00.Rate this question:
-
- 40.
Simpangan baku dari sekelompok data tunggal: 7, 8, 5, 4, 6 adalah ... .
- A.
- B.
2
- C.
- D.
- E.
6
Correct Answer
A.Explanation
The correct answer is 1. To find the standard deviation (simpangan baku), we need to calculate the mean of the data first. The mean of the data 7, 8, 5, 4, 6 is (7+8+5+4+6)/5 = 6. Therefore, the deviations from the mean are 1, 2, -1, -2, 0. To find the standard deviation, we need to square these deviations, sum them up, divide by the number of data points, and then take the square root. However, since the deviations are already small, squaring them will only make them larger. So, the standard deviation is approximately 1.Rate this question:
-
Quiz Review Timeline +
Our quizzes are rigorously reviewed, monitored and continuously updated by our expert board to maintain accuracy, relevance, and timeliness.
-
Current Version
-
Mar 21, 2023Quiz Edited by
ProProfs Editorial Team -
Jan 11, 2019Quiz Created by
NurasiahZamil




.PNG)

.PNG)

.PNG)
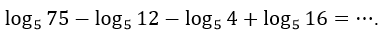
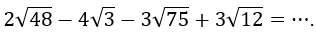




.PNG)