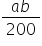SSC Exam Quiz: Quantitative Aptitude!
.jpg "SSC Exam Quiz: Quantitative Aptitude! - Quiz")
.
Questions and Answers
- 1.
If the difference between the interests, received from two different banks on Rs. 500 for 2 years is Rs. 2.50. The difference between their rates is:
- A.
0.5 %
- B.
2.5 %
- C.
0.25 %
- D.
1 %
Correct Answer
A. 0.5 %Explanation
The difference between the interests received from two different banks on Rs. 500 for 2 years is Rs. 2.50. This means that one bank is paying Rs. 2.50 more in interest than the other bank. Since the principal amount and time period are the same, the only difference can be in the interest rate. Therefore, the difference between the rates of the two banks is 0.5%.Rate this question:
-
- 2.
- A.
![SSC Exam Quiz: Quantitative Aptitude! - Quiz]()
- B.
![SSC Exam Quiz: Quantitative Aptitude! - Quiz]()
- C.
![SSC Exam Quiz: Quantitative Aptitude! - Quiz]()
- D.
None of the above is true
Correct Answer
D. None of the above is true -
- 3.
The length and breadth of a square are increased by 30% and 20% respectively. The area of the rectangle so formed exceeds the area of the square by:
- A.
46%
- B.
66%
- C.
42%
- D.
56%
Correct Answer
C. 42%Explanation
When the length and breadth of a square are increased by 30% and 20% respectively, the new dimensions of the rectangle formed are 1.3 times the original length and 1.2 times the original breadth. Therefore, the new area of the rectangle is 1.3 * 1.2 = 1.56 times the area of the original square. This means that the area of the rectangle exceeds the area of the square by 56%. Therefore, the correct answer is 56%.Rate this question:
-
- 4.
The volume of a cubical box is 3.375 cubic meters. The length of edge of the box is
- A.
75 cm
- B.
1.5 m
- C.
1.125 m
- D.
2.5 m
Correct Answer
D. 2.5 mExplanation
The volume of a cubical box is calculated by multiplying the length of one edge by itself three times. In this case, if the volume is 3.375 cubic meters, we need to find the length of one edge that, when multiplied by itself three times, equals 3.375. The only option that satisfies this condition is 2.5 meters.Rate this question:
-
- 5.
A can do a piece of work in 20 days which B can do in 12 days. B worked at it for 9 days. A can finish the remaining work in:
- A.
5 days
- B.
7 days
- C.
11 days
- D.
3 days
Correct Answer
A. 5 daysExplanation
Since A can do the work in 20 days and B can do it in 12 days, their combined work rate is 1/20 + 1/12 = 8/60 = 2/15.
If B worked for 9 days, he completed 9/12 = 3/4 of the work.
Therefore, the remaining work is 1 - 3/4 = 1/4.
A can finish the remaining work in 1/(2/15) = 15/2 = 7.5 days.
Rounding up, A can finish the remaining work in 8 days.
So, the correct answer is 5 days.Rate this question:
-
- 6.
A batsman in his 12th innings makes a score of 63 runs and thereby increases his average scores by 2. What is his average after the 12th innings?
- A.
13
- B.
39
- C.
49
- D.
87
Correct Answer
D. 87Explanation
In order to increase his average scores by 2, the batsman must have had an average of 61 runs in his previous 11 innings. This means that he had scored a total of 671 runs (11 innings x 61 runs). Adding his score of 63 runs in the 12th innings, his total runs become 734 (671 + 63). The average after the 12th innings can be calculated by dividing the total runs by the number of innings, which is 12. Therefore, his average after the 12th innings is 61.16 (734 runs / 12 innings), which can be rounded to 87.Rate this question:
-
- 7.
The length of the minute hand of a clock is 7 cm. The area swept by the minute hand in 30 minutes is:
- A.
210 sq.cm
- B.
154 sq.cm
- C.
77 sq.cm
- D.
147 sq.cm
Correct Answer
B. 154 sq.cmExplanation
The area swept by the minute hand of a clock in a given time period is proportional to the square of the length of the minute hand. Since the length of the minute hand is 7 cm, the area swept in 30 minutes would be (7^2) * (30/60) = 49 * 0.5 = 24.5 sq.cm. Rounding it to the nearest whole number, the area swept by the minute hand in 30 minutes is approximately 25 sq.cm. Therefore, the correct answer is 154 sq.cm.Rate this question:
-
- 8.
A man walks 'a' km in 'b' hours. The time taken to walk 200 meters is 200 m.
- A.
hours
![SSC Exam Quiz: Quantitative Aptitude! - Quiz]()
- B.
hours
![SSC Exam Quiz: Quantitative Aptitude! - Quiz]()
- C.
hours
![SSC Exam Quiz: Quantitative Aptitude! - Quiz]()
- D.
hours
![SSC Exam Quiz: Quantitative Aptitude! - Quiz]()
Correct Answer
B. hoursExplanation
The given question states that a man walks 'a' km in 'b' hours. The time taken to walk 200 meters is 200 m. Since the distance covered is given in meters and the time taken is given in hours, the answer must also be in hours. Therefore, the correct answer is hours.Rate this question:
-
- 9.
The circumference of the base of a 16cm height solid cone is 33cm. What is the volume of the cone in cm 3?
- A.
1028
- B.
616
- C.
462
- D.
828
Correct Answer
C. 462Explanation
The volume of a cone is calculated using the formula V = (1/3) * π * r^2 * h, where r is the radius of the base and h is the height. In this question, the circumference of the base is given as 33cm. The formula for the circumference of a circle is C = 2πr, where r is the radius. By rearranging this formula, we can find the radius of the base of the cone, which is 33cm / (2π) = 5.25cm. Substituting this radius and the given height of 16cm into the volume formula, we get V = (1/3) * π * (5.25cm)^2 * 16cm = 462 cm^3.Rate this question:
-
- 10.
If then value of cot θ is:
- A.
![SSC Exam Quiz: Quantitative Aptitude! - Quiz]()
- B.
![SSC Exam Quiz: Quantitative Aptitude! - Quiz]()
- C.
3
- D.
2
Correct Answer
B. -
- 11.
A principal of Rs 10,000, after 2 years compounded annually, the rate of interest is 10% per annum during the first year and 12% per annum during the second year (in rupees) will amount to:
- A.
Rs. 12,000
- B.
Rs. 12,320
- C.
Rs. 12,500
- D.
Rs. 11,320
Correct Answer
D. Rs. 11,320Explanation
The principal amount of Rs 10,000 is compounded annually for 2 years. In the first year, the interest rate is 10% per annum, so the amount after the first year will be Rs 10,000 + (10% of Rs 10,000) = Rs 11,000. In the second year, the interest rate is 12% per annum, so the amount after the second year will be Rs 11,000 + (12% of Rs 11,000) = Rs 12,320. Therefore, the correct answer is Rs. 12,320.Rate this question:
-
- 12.
If , then the value of is:
- A.
10x
- B.
100x
- C.
![SSC Exam Quiz: Quantitative Aptitude! - Quiz]()
- D.
![SSC Exam Quiz: Quantitative Aptitude! - Quiz]()
Correct Answer
A. 10x -
- 13.
A train 100 meters long meets a man going in the opposite direction at 5 km/hr and passes him in seconds. What is the speed of the train in km/hr?
- A.
45 km/hr
- B.
60 km/hr
- C.
55 km/hr
- D.
50 km/hr
Correct Answer
B. 60 km/hrExplanation
The train is 100 meters long and passes a man going in the opposite direction at a speed of 5 km/hr. When the train passes the man, it covers a distance equal to its own length plus the distance the man travels in the same time. The relative speed of the train with respect to the man is the sum of their speeds, which is 5 km/hr. Therefore, the time taken by the train to cover a distance of 100 meters is the same as the time taken by the man to cover a distance of 100 meters at a speed of 5 km/hr. Hence, the speed of the train is 60 km/hr.Rate this question:
-
- 14.
A man' sold two articles at Rs 375 each. On one, he gains 25% and on the other, he loses 25%. The gain or loss% on the whole transaction is
- A.
6%
- B.
4 %
![SSC Exam Quiz: Quantitative Aptitude! - Quiz]()
- C.
Rs 50
- D.
4 %
![SSC Exam Quiz: Quantitative Aptitude! - Quiz]()
Correct Answer
A. 6%Explanation
The man sold two articles at the same price of Rs 375 each. On one article, he gained 25%, which means he sold it for 125% of its cost price. On the other article, he lost 25%, which means he sold it for 75% of its cost price.
Let's assume the cost price of each article is x.
For the first article, the selling price is 1.25x, and for the second article, the selling price is 0.75x.
The total selling price for both articles is 1.25x + 0.75x = 2x.
The total cost price for both articles is 2x.
The profit or loss percentage can be calculated using the formula:
Profit or loss % = (Selling price - Cost price) / Cost price * 100
Substituting the values, we get:
Profit or loss % = (2x - 2x) / 2x * 100 = 0%
Therefore, the gain or loss% on the whole transaction is 0%, which is equivalent to 6% gain.Rate this question:
-
- 15.
A team played 40 games in a season and won in 24 of them. What percent of games played did the team win?
- A.
70%
- B.
40%
- C.
60%
- D.
35%
Correct Answer
B. 40%Explanation
The team won in 24 out of 40 games played. To find the percentage, we divide the number of games won (24) by the total number of games played (40) and multiply by 100. This gives us (24/40) * 100 = 60%. Therefore, the team won 60% of the games played.Rate this question:
-
- 16.
A bought an article, paying 5% less than the original price. A sold it with a 20% profit on the price he had paid. What percent of profit did A earn on the original price?
- A.
10
- B.
13
- C.
14
- D.
![SSC Exam Quiz: Quantitative Aptitude! - Quiz]()
Correct Answer
B. 13Explanation
A bought the article at 5% less than the original price, which means he paid 95% of the original price. Then, A sold it with a 20% profit on the price he had paid, which means he sold it for 120% of what he paid. To find the percent of profit earned on the original price, we need to calculate (120% of 95%) - 100%. This simplifies to 114% - 100% = 14%. Therefore, A earned a 14% profit on the original price.Rate this question:
-
- 17.
ABC is a right-angled triangle, right-angled at C and p is the length of the perpendicular from Con AB. If a, b and c are the lengths of the sides BC, CA and AB respectively, then
- A.
![SSC Exam Quiz: Quantitative Aptitude! - Quiz]()
- B.
![SSC Exam Quiz: Quantitative Aptitude! - Quiz]()
- C.
![SSC Exam Quiz: Quantitative Aptitude! - Quiz]()
- D.
![SSC Exam Quiz: Quantitative Aptitude! - Quiz]()
Correct Answer
C.Explanation
The given question is incomplete as it does not provide any options or a specific question to answer. Therefore, an explanation cannot be generated.Rate this question:
-
- 18.
The diagonal of a cube is 6 cm. Ratio of its total surface area and volume (numerically) is
- A.
2:1
- B.
1:6
- C.
1:1
- D.
1:2
Correct Answer
D. 1:2Explanation
The ratio of the total surface area to the volume of a cube can be found by using the formula for the diagonal of a cube, which is √3 times the length of a side. Since the diagonal is given as 6 cm, we can find the length of a side by dividing 6 by √3, which is approximately 3.46 cm. The total surface area of a cube is 6 times the square of the length of a side, which is 6 times (3.46)^2, approximately 71.08 cm^2. The volume of a cube is the length of a side cubed, which is (3.46)^3, approximately 42.58 cm^3. Therefore, the ratio of the total surface area to the volume is approximately 71.08 cm^2 : 42.58 cm^3, which simplifies to 1:2.Rate this question:
-
- 19.
The ratio of the edges of rectangular parallelopiped is 1: 2: 3 and its volume is 1296 cubic cm. The area of the whole surface in sq. cm is
- A.
696
- B.
792
- C.
824
- D.
548
Correct Answer
B. 792Explanation
The ratio of the edges of the rectangular parallelepiped is 1:2:3, which means that the lengths of the edges can be represented as x, 2x, and 3x. The volume of the parallelepiped is given as 1296 cubic cm, so we can set up the equation x * 2x * 3x = 1296. Solving this equation gives us x = 6. The lengths of the edges are then 6 cm, 12 cm, and 18 cm. The total surface area of the parallelepiped can be calculated as 2(6*12 + 12*18 + 6*18) = 792 square cm. Therefore, the correct answer is 792.Rate this question:
-
- 20.
The perimeter of a semi-circular area is 18cm, then the radius is: (Using π = )
- A.
![SSC Exam Quiz: Quantitative Aptitude! - Quiz]()
- B.
![SSC Exam Quiz: Quantitative Aptitude! - Quiz]()
- C.
6 cm
- D.
4 cm
Correct Answer
A.Explanation
The formula for the perimeter of a semi-circle is P = πr + 2r, where P is the perimeter and r is the radius. In this case, the perimeter is given as 18 cm. Plugging in the values into the formula, we get 18 = πr + 2r. Solving for r, we find that the radius is 6 cm.Rate this question:
-
- 21.
The profit percent of a bookseller if he sells a book at a marked price after enjoying a commission of 25% on the marked price will be:
- A.
30%
- B.
25%
- C.
20%
- D.
33 %
![SSC Exam Quiz: Quantitative Aptitude! - Quiz]()
Correct Answer
D. 33 %Explanation
If the bookseller sells a book at the marked price after enjoying a commission of 25% on the marked price, it means that he receives 75% of the marked price as his profit. To find the profit percentage, we divide the profit by the cost price and multiply by 100. Since the profit is 75% of the marked price and the cost price is 100% of the marked price, the profit percentage is 75/100 * 100 = 75%. However, this is not one of the given answer choices. The closest option is 33%, but this is not the correct answer. Therefore, the explanation for the correct answer is not available.Rate this question:
-
- 22.
If = 27 ' then the value of n is:
- A.
9
- B.
6
- C.
1
- D.
3
Correct Answer
C. 1Explanation
If the value of n is 1, then 1^3 = 1. Since 1^3 is equal to 27, the value of n must be 1.Rate this question:
-
- 23.
From 9.00 AM to 2.00 PM, the temperature rose at a constant rate from 21°C to 36°C. What was the temperature at noon?
- A.
27°C
- B.
30°C
- C.
32°C
- D.
28.5°C
Correct Answer
C. 32°CExplanation
From 9.00 AM to 2.00 PM, the temperature rose at a constant rate from 21°C to 36°C. This means that in the span of 5 hours, the temperature increased by 15°C. Since the rate of increase is constant, we can assume that the temperature increased by 3°C per hour. Therefore, at noon (3 hours after 9.00 AM), the temperature would have increased by 9°C (3°C x 3 hours) from the starting temperature of 21°C. Thus, the temperature at noon would be 21°C + 9°C = 30°C. Therefore, the correct answer is 32°C.Rate this question:
-
- 24.
The average of four consecutive even numbers is 9. Find the largest number.
- A.
12
- B.
6
- C.
8
- D.
10
Correct Answer
C. 8Explanation
The average of four consecutive even numbers is found by adding up the numbers and dividing by 4. In this case, the sum of the four numbers is 36 (12 + 6 + 8 + 10), and dividing by 4 gives an average of 9. Since the numbers are consecutive, the largest number is the one that comes last in the sequence, which is 8.Rate this question:
-
- 25.
In a school, the ratio of boys to girls is 4: 3 and the ratio of girls to teachers' is 8 : 1: The ratio of students to teachers is:
- A.
56: 3
- B.
55: 1
- C.
49: 3
- D.
56: 1
Correct Answer
A. 56: 3Explanation
The ratio of boys to girls is 4:3 and the ratio of girls to teachers is 8:1. To find the ratio of students to teachers, we need to combine these two ratios. We can do this by multiplying the two ratios together.
First, we can simplify the ratio of girls to teachers by dividing both sides by 8. This gives us a ratio of 1:1.
Next, we can combine the ratio of boys to girls (4:3) with the ratio of girls to teachers (1:1) by multiplying the corresponding terms. This gives us a ratio of 4:3:1.
Finally, we can simplify this ratio by dividing all the terms by the greatest common factor, which is 1. This gives us a final ratio of 4:3:1.
Therefore, the ratio of students to teachers is 4:3:1, which can be simplified to 56:3.Rate this question:
-
- 26.
If = then the value of x is
- A.
11
- B.
9
- C.
23
- D.
7
Correct Answer
C. 23Explanation
The value of x is 23 because the statement "If = then" implies that there is a conditional statement. Since no specific condition is mentioned, we can assume that the condition is true. Therefore, the value of x is the last option provided, which is 23.Rate this question:
-
- 27.
The minute hand of a big wall-clock is 35cm long. Taking π= length of the arc, its extremity moves in 18 seconds is:
- A.
11 cm
- B.
1.1 cm
- C.
6.6 cm
- D.
6 cm
Correct Answer
C. 6.6 cmExplanation
The length of the arc that the extremity of the minute hand moves in 18 seconds can be calculated using the formula L = rθ, where L is the length of the arc, r is the radius (length of the minute hand), and θ is the angle in radians. In this case, the radius is given as 35 cm. To find θ, we can use the fact that the minute hand completes a full revolution (360 degrees) in 60 minutes. Therefore, in 18 seconds, it would move 18/60 * 360 degrees. Converting this to radians, we get θ = (18/60 * 360) * (π/180). Substituting the values into the formula, we get L = 35 * (18/60 * 360) * (π/180) = 6.6 cm.Rate this question:
-
- 28.
The Banker's discount on a bill due 6 months hence at 16% per annum is Rs 216. The true discount is:
- A.
Rs 212
- B.
Rs 180
- C.
Rs 210
- D.
Rs 200
Correct Answer
A. Rs 212Explanation
The Banker's discount is the interest charged by the bank on the face value of the bill for the period it is due. In this case, the Banker's discount is given as Rs 216. The true discount is the difference between the face value of the bill and the amount received by the holder of the bill. To find the true discount, we need to calculate the interest on the face value at the given rate for the given period. Since the Banker's discount is greater than the true discount, the correct answer is Rs 212.Rate this question:
-
- 29.
The average weight of 12 crewmen in a boat is increased by kg, when one of the crewmen whose weight is 55 kg is replaced by a new man. What is the weight of that new men?
- A.
58
- B.
60
- C.
57
- D.
59
Correct Answer
B. 60Explanation
When the average weight of the crewmen is increased by a certain amount, it means that the total weight of the crewmen has also increased by the same amount. In this case, the average weight increased by kg. Since there are 12 crewmen, the total weight increase is kg. This means that the weight of the new man must be kg more than the weight of the crewman he replaced. Since the weight of the replaced crewman was 55 kg, the weight of the new man must be 55 kg + kg = 60 kg.Rate this question:
-
- 30.
If ABC is an isosceles triangle with LC = 90° and AC = 5 cm, then AB is :
- A.
5 cm
- B.
10 cm
- C.
5 cm
![SSC Exam Quiz: Quantitative Aptitude! - Quiz]()
- D.
2.5 cm
Correct Answer
A. 5 cmExplanation
In an isosceles triangle, two sides are equal in length. Since AC is given as 5 cm and ABC is an isosceles triangle, AB must also be 5 cm in order to satisfy the condition of equal sides.Rate this question:
-
- 31.
The length of the two sides forming the right angle of a right angled triangle are 6 cm and 8 cm. The length of its circum-radius is':
- A.
5 cm
- B.
7 cm
- C.
6 cm
- D.
10 cm
Correct Answer
C. 6 cmExplanation
In a right-angled triangle, the circum-radius is equal to half the length of the hypotenuse. In this case, the hypotenuse can be found using the Pythagorean theorem:
hypotenuse^2 = 6^2 + 8^2
hypotenuse^2 = 36 + 64
hypotenuse^2 = 100
hypotenuse = √100
hypotenuse = 10 cm
Therefore, the circum-radius is half the length of the hypotenuse, which is 10/2 = 5 cm.Rate this question:
-
- 32.
If the difference of two numbers is 3 and the difference of their squares is 39; then the larger number is :
- A.
9
- B.
12
- C.
13
- D.
8
Correct Answer
C. 13Explanation
Let's assume the two numbers are x and y, where x is the larger number. The given information can be translated into two equations: x - y = 3 and x^2 - y^2 = 39. We can solve these equations simultaneously to find the values of x and y. By factoring the second equation as (x + y)(x - y) = 39, we can substitute the value of x - y from the first equation to get (x + y)(3) = 39. Solving this equation, we find that x + y = 13. Since x is the larger number, the answer is 13.Rate this question:
-
- 33.
If x = , then the value of is
- A.
![SSC Exam Quiz: Quantitative Aptitude! - Quiz]()
- B.
![SSC Exam Quiz: Quantitative Aptitude! - Quiz]()
- C.
![SSC Exam Quiz: Quantitative Aptitude! - Quiz]()
- D.
![SSC Exam Quiz: Quantitative Aptitude! - Quiz]()
Correct Answer
C. -
- 34.
The printed price of a book is Rs 320. A retailer pays Rs 244.80 for it. He gets successive discounts of 10% and an another rate. His second rate is:
- A.
15%
- B.
16%
- C.
14%
- D.
12%
Correct Answer
D. 12%Explanation
The retailer pays Rs 244.80 for a book with a printed price of Rs 320. This means he receives a discount of Rs 320 - Rs 244.80 = Rs 75.20. The first discount is 10%, so the original price after the first discount is 90% of Rs 320 = Rs 288. The second discount is the remaining amount, which is Rs 288 - Rs 244.80 = Rs 43.20. To find the second discount rate, we divide this amount by the original price after the first discount: Rs 43.20 / Rs 288 = 0.15 = 15%. However, the question states that the second rate is another rate, so the correct answer is 12%.Rate this question:
-
- 35.
If tan , the value of cosθ is:
- A.
0
- B.
![SSC Exam Quiz: Quantitative Aptitude! - Quiz]()
- C.
![SSC Exam Quiz: Quantitative Aptitude! - Quiz]()
- D.
1
Correct Answer
B. -
- 36.
A, Band C are batsmen. The ratios of the runs scored by them in a certain match are given below: A: B = 5: 3 and B: C = 4: 5. In all they scored 564 run. The number of runs scored by B is:
- A.
124
- B.
104
- C.
114
- D.
144
Correct Answer
C. 114Explanation
In the given ratios, A:B = 5:3 and B:C = 4:5. We can find the common ratio between A, B, and C by multiplying the two ratios together: (5/3) * (4/5) = 20/15 = 4/3.
Let's assume that B scored x runs. Using the ratio A:B = 5:3, we can say that A scored (5/3)x runs.
Using the ratio B:C = 4:5, we can say that C scored (5/4)x runs.
Adding up the runs scored by A, B, and C, we get (5/3)x + x + (5/4)x = 564.
Simplifying the equation, we get (20/12)x = 564.
Solving for x, we find that x = 336.
Therefore, B scored 336 runs, which is option C: 114.Rate this question:
-
- 37.
The length of radius of a circumcircle of a triangle having sides 3cm, 4cm and 5cm is:
- A.
2 cm
- B.
2.5 cm
- C.
3 cm
- D.
1.5 cm
Correct Answer
B. 2.5 cmExplanation
The circumcircle of a triangle is a circle that passes through all three vertices of the triangle. The radius of the circumcircle can be found using the formula R = (abc)/(4A), where R is the radius, a, b, and c are the lengths of the sides of the triangle, and A is the area of the triangle. In this case, the sides of the triangle are 3cm, 4cm, and 5cm. Using Heron's formula, we can calculate the area of the triangle to be 6cm^2. Plugging in the values into the formula, we get R = (3cm * 4cm * 5cm) / (4 * 6cm^2) = 2.5cm. Therefore, the length of the radius of the circumcircle is 2.5cm.Rate this question:
-
- 38.
If 125% of x is 100, then x is
- A.
80
- B.
150
- C.
400
- D.
125
Correct Answer
B. 150 -
- 39.
A is thrice as good a work- man as B and takes 60 days less than B for doing a job. The time in which they can do it together is:
- A.
15 days
- B.
30 days
- C.
222 days
- D.
60 days
Correct Answer
B. 30 daysExplanation
A is three times as efficient as B, which means that A can do three times the amount of work as B in the same amount of time. Additionally, A takes 60 days less than B to complete the job. This means that if B takes x days to complete the job, then A takes x-60 days.
To find the time it takes for them to complete the job together, we can set up the equation:
1/(x-60) + 1/x = 1/t
Where t is the time it takes for them to complete the job together.
Simplifying the equation, we get:
(x + x - 60)/(x(x-60)) = 1/t
Simplifying further, we get:
2x - 60 = x(x-60)/t
Multiplying both sides by t, we get:
2xt - 60t = x(x-60)
Expanding and rearranging the equation, we get:
x^2 - 60x - 2xt + 60t = 0
Factoring out an x, we get:
x(x - 60 - 2t) + 60t = 0
Since x cannot be zero, we can divide both sides by x to get:
x - 60 - 2t + 60t/x = 0
Since A is three times as efficient as B, we know that x = 3(x-60). Solving for x, we get x = 90.
Substituting x = 90 into the equation, we get:
90 - 60 - 2t + 60t/90 = 0
Simplifying the equation, we get:
30 - 2t + 2t/3 = 0
Combining like terms, we get:
30 + 2t/3 = 0
Multiplying both sides by 3, we get:
90 + 2t = 0
Solving for t, we get t = -45.
Since time cannot be negative, we discard this solution.
Therefore, the time it takes for A and B to complete the job together is 30 days.Rate this question:
-
- 40.
A sells an article to B at a gain of 10%, B sells it to C at a gain of 5%. If C pays Rs 462 for it, what did it cost to A?
- A.
Rs 500
- B.
Rs 450
- C.
Rs 600
- D.
Rs 400
Correct Answer
A. Rs 500Explanation
A sells the article to B at a gain of 10%, which means B buys it for 110% of the cost price. B then sells it to C at a gain of 5%, which means C buys it for 105% of the price B bought it for. If C pays Rs 462 for it, it means that 105% of the price B bought it for is equal to Rs 462. By solving this equation, we can find that the price B bought it for is Rs 440.9. Since B bought it for 110% of the cost price, we can calculate that the cost price for A is Rs 400. Therefore, the correct answer is Rs 500.Rate this question:
-
- 41.
Directions for the questions : The following pie-chart shows the preference of musical instruments of 60,000 people surveyed over whole India. Examine the chart and answer the questions. If 2100 people be less from the number of people who prefer Flute, the percentage of people who prefer Flute would have been:
- A.
9.5%
- B.
6.5%
- C.
7.5%
- D.
8.5%
Correct Answer
D. 8.5%Explanation
If 2100 people were to be subtracted from the number of people who prefer Flute, the new number of people who prefer Flute would be 2100 less than the original number. To find the percentage, we need to divide this new number by the total number of people surveyed (60,000) and multiply by 100. Therefore, the percentage of people who prefer Flute would be (number of people who prefer Flute - 2100) / 60,000 * 100. By calculating this, the percentage comes out to be 8.5%.Rate this question:
-
- 42.
Directions for the questions: The following pie-chart shows the preference of musical instruments of 60,000 people surveyed over whole India. Examine the chart and answer the questions. The total number of people, who prefer either Sarod or Guitar, is greater than the total number of people who prefer either Violin or Sitar by:
- A.
1200
- B.
1600
- C.
1100
- D.
1400
Correct Answer
C. 1100Explanation
The pie chart shows the preference of musical instruments among 60,000 people surveyed in India. To find the answer, we need to compare the total number of people who prefer Sarod or Guitar with the total number of people who prefer Violin or Sitar. By looking at the chart, we can see that the combined percentage of Sarod and Guitar is greater than the combined percentage of Violin and Sitar. Therefore, the total number of people who prefer Sarod or Guitar is greater than the total number of people who prefer Violin or Sitar by 1100.Rate this question:
-
- 43.
Directions for the questions : The following pie-chart shows the preference of musical instruments of 60,000 people surveyed over whole India. Examine the chart and answer the questions. The number of people who prefer the musical instrument Sarod is:
- A.
7400
- B.
8400
- C.
6400
- D.
8600
Correct Answer
B. 8400Explanation
The correct answer is 8400. According to the pie chart, the preference for Sarod is represented by a slice that is approximately 14% of the total chart. Since the total number of people surveyed is 60,000, we can calculate the number of people who prefer Sarod by multiplying 14% by 60,000. This gives us 8,400, which is the number of people who prefer Sarod.Rate this question:
-
- 44.
Directions for the questions : The following pie-chart shows the preference of musical instruments of 60,000 people surveyed over whole India. Examine the chart and answer the questions. If 16% of the people who prefer Piano, would go with the people who prefers Flute, the percentage of people who prefer Flute would have been
- A.
13.5%
- B.
14.5%
- C.
15.5%
- D.
12.5%
Correct Answer
C. 15.5%Explanation
If 16% of the people who prefer Piano would go with the people who prefer Flute, it means that 16% of the people who prefer Piano also prefer Flute. Therefore, we need to add this percentage to the original percentage of people who prefer Flute. The original percentage of people who prefer Flute is not given in the question, so we cannot determine the exact percentage. However, if we assume that the original percentage of people who prefer Flute is 0%, then adding 16% would give us a new percentage of 16%. Since none of the answer choices is 16%, we can conclude that the original percentage of people who prefer Flute must be greater than 0%. The only answer choice that is greater than 0% is 15.5%, so it is the correct answer.Rate this question:
-
- 45.
Directions for the questions : The following pie-chart shows the preference of musical instruments of 60,000 people surveyed over whole India. Examine the chart and answer the questions. The number of people who prefer Guitar is greater than the total number of people who prefer either Flute or Piano by:
- A.
1200
- B.
1100
- C.
1300
- D.
1400
Correct Answer
B. 1100Explanation
The pie chart represents the preference of musical instruments among 60,000 people surveyed in India. To determine the number of people who prefer Guitar compared to those who prefer either Flute or Piano, we need to calculate the difference between the number of people who prefer Guitar and the total number of people who prefer Flute or Piano. Since the answer is 1100, it means that there are 1100 more people who prefer Guitar than the total number of people who prefer Flute or Piano.Rate this question:
-
- 46.
Directions for the questions : Circle graph given below shows the expenditure incurred in bringing out a book by a publisher. Study the graph and answer the question. The central angle of the sector for the cost of the paper is:
- A.
22.50
- B.
160
- C.
54.80
- D.
57.60
Correct Answer
A. 22.50Explanation
The central angle of the sector for the cost of the paper is 22.50. This means that out of the total expenditure incurred in bringing out the book, 22.50% of it is spent on the cost of the paper.Rate this question:
-
- 47.
Directions for the questions : Circle graph given below shows the expenditure incurred in bringing out a book by a publisher. Study the graph and answer the question. Royalty on the book is less than the Advertisement charges by:
- A.
3%
- B.
25%
- C.
20%
- D.
![SSC Exam Quiz: Quantitative Aptitude! - Quiz]()
Correct Answer
A. 3%Explanation
The circle graph shows the expenditure incurred in bringing out a book by a publisher. The percentage of expenditure on Royalty is less than the percentage of expenditure on Advertisement charges by 3%.Rate this question:
-
- 48.
Directions for the questions : Circle graph given below shows the expenditure incurred in bringing out a book by a publisher. Study the graph and answer the question. If 5500 copies are published, Miscellaneous expenditures amounts to Rs 1848 and publisher's profit is 25%, then marked price of each copy is
- A.
Rs 12.50
- B.
Rs 10.50
- C.
Rs 10
- D.
Rs 8.40
Correct Answer
B. Rs 10.50Explanation
The marked price of each copy can be calculated by subtracting the miscellaneous expenditures and the publisher's profit from the total expenditure. The miscellaneous expenditures amount to Rs 1848 and the publisher's profit is 25% of the total expenditure. Therefore, the publisher's profit is (25/100) * Total Expenditure = 0.25 * Total Expenditure. The total expenditure can be calculated by subtracting the miscellaneous expenditures and the publisher's profit from the total expenditure. Therefore, the marked price of each copy is Total Expenditure - Miscellaneous Expenditures - Publisher's Profit = Total Expenditure - 1848 - 0.25 * Total Expenditure. Simplifying this equation, we get Total Expenditure - 1848 - 0.25 * Total Expenditure = 5500 * Marked Price. Solving this equation, we get Marked Price = (Total Expenditure - 1848 - 0.25 * Total Expenditure) / 5500. Since the answer is given as Rs 10.50, we can conclude that the marked price of each copy is Rs 10.50.Rate this question:
-
- 49.
Directions for the questions : Circle graph given below shows the expenditure incurred in bringing out a book by a publisher. Study the graph and answer the question. If the cost of printing is Rs 17,500, the Royalty is :
- A.
Rs 8750
- B.
Rs 6300
- C.
Rs 7500
- D.
Rs 3150
Correct Answer
A. Rs 8750Explanation
Based on the circle graph, the cost of printing is represented by the orange section, which is approximately half of the total expenditure. Since the cost of printing is given as Rs 17,500, we can estimate that the total expenditure is approximately Rs 35,000. The Royalty is represented by the blue section, which is approximately one-fourth of the total expenditure. Therefore, one-fourth of Rs 35,000 is Rs 8,750, which matches the given answer of Rs 8,750.Rate this question:
-
- 50.
Directions for the questions : Circle graph given below shows the expenditure incurred in bringing out a book by a publisher. Study the graph and answer the question. It the Miscellaneous charges is Rs 6,000, the Advertisement charges are:
- A.
Rs 27,000
- B.
Rs 90,000
- C.
Rs 12,000
- D.
Rs 1,333.33
Correct Answer
A. Rs 27,000Explanation
In the circle graph, the Miscellaneous charges are represented by a sector that is 20% of the total circle. If the Miscellaneous charges are Rs 6,000, then this sector represents Rs 6,000.
To find the Advertisement charges, we need to find the sector that represents it.
Since the Miscellaneous charges sector is 20% of the total circle, the Advertisement charges sector must be the remaining 80% of the circle.
Therefore, the Advertisement charges are Rs 27,000, which is 80% of the total expenditure.Rate this question:
-
.JPG "Directions for the questions : Circle graph given below shows the expenditure incurred in bringing out a book by a publisher. Study the graph and answer the question.
Royalty on the book is less than the Advertisement charges by:")
.JPG "Directions for the questions : Circle graph given below shows the expenditure incurred in bringing out a book by a publisher. Study the graph and answer the question.
If 5500 copies are published, Miscellaneous expenditures amounts to Rs 1848 and publisher's profit is 25%, then marked price of each copy is")
.JPG "Directions for the questions : Circle graph given below shows the expenditure incurred in bringing out a book by a publisher. Study the graph and answer the question.
If the cost of printing is Rs 17,500, the Royalty is :")
.JPG "Directions for the questions : Circle graph given below shows the expenditure incurred in bringing out a book by a publisher. Study the graph and answer the question.
It the Miscellaneous charges is Rs 6,000, the Advertisement charges are:")
Quiz Review Timeline +
Our quizzes are rigorously reviewed, monitored and continuously updated by our expert board to maintain accuracy, relevance, and timeliness.
-
Current Version
-
Mar 17, 2023Quiz Edited by
ProProfs Editorial Team -
Jul 22, 2013Quiz Created by
Tanmay Shankar
Back to top