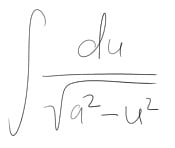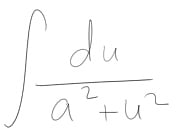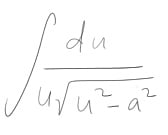Integrals Of Trigonometric Functions Trivia Quiz
(31).jpg "Integrals Of Trigonometric Functions Trivia Quiz - Quiz")
How well do you remember the integrals of the trigonometric functions? For this quiz, you must know how to do integrations using various trigonometric identities, solving some questions for us. This quiz is best to strengthen your basics and prepare for an upcoming exam. All the Best!
.
- 1.
∫sin(u)du
- A.
Ln|secu + tanu)| + C
- B.
Tan(u)+C
- C.
Sec(u)+C
- D.
-cos(u) + C
- E.
Cos(u)+C
Correct Answer
D. -cos(u) + CExplanation
The integral of sin(u) with respect to u is equal to -cos(u) + C, where C is the constant of integration. This can be found using the integral rules for trigonometric functions. The antiderivative of sin(u) is -cos(u), and adding the constant of integration C gives the final result.Rate this question:
-
- 2.
∫cos(u)du
- A.
Sin(u)+C
- B.
Ln|sin(u)| + C
- C.
-cot(u) + C
- D.
(1/a)arcsec(|u|/a) + C
- E.
-csc(u) + C
Correct Answer
A. Sin(u)+CExplanation
The integral of cos(u) with respect to u is equal to sin(u) plus a constant (C). This is because the derivative of sin(u) is equal to cos(u), which means that the integral of cos(u) is sin(u) plus a constant.Rate this question:
-
- 3.
∫tan(u)du
- A.
-ln|cos(u)| + C
- B.
(1/a)arctan(u/a) + C
- C.
-sec^2(u) + C
- D.
Csc(u)*cot(u)
- E.
-cot(u) + C
Correct Answer
A. -ln|cos(u)| + C -
- 4.
∫cot(u)du
- A.
Ln|sin(u)| + C
- B.
(1/a)arctan(u/a) + C
- C.
-cot(u) + C
- D.
Sin(u)+C
- E.
Ln|secu + tanu)| + C
Correct Answer
A. Ln|sin(u)| + CExplanation
The integral of cot(u) can be found by using the formula for the integral of the cotangent function, which is ln|sin(u)| + C. This formula is derived from the derivative of the natural logarithm function and the chain rule. Therefore, the correct answer is ln|sin(u)| + C.Rate this question:
-
- 5.
∫du/(a^2 - u^2)
- A.
Arctan(u/a) + C
- B.
Arcsin(u/a) + C
- C.
(1/a) arcsin (u/a) + C
- D.
(1/u) arcsin (|u|/a) + C
- E.
(1/a) arcsec (|u|/a) + C
Correct Answer
B. Arcsin(u/a) + CExplanation
The integral of du/(a^2 - u^2) can be solved using the substitution method. Let u = a*sin(theta), then du = a*cos(theta)d(theta). Substituting these values into the integral, we get ∫(a*cos(theta)d(theta))/(a^2 - a^2*sin^2(theta)). Simplifying further, we have ∫(a*cos(theta)d(theta))/(a^2*cos^2(theta)). Canceling out the common terms, we are left with ∫(1/a)d(theta). Integrating this, we get (1/a)theta + C. Replacing theta with arcsin(u/a), we get arcsin(u/a) + C as the final answer.Rate this question:
-
- 6.
∫du/(a^2 + u^2)
- A.
(1/u) arcsin (|u|/a) + C
- B.
Arctan(u/a) + C
- C.
Arcsin(u/a) + C
- D.
(1/a) arctan (u/a) + C
- E.
(1/a) arctan (|u|/a) + C
Correct Answer
D. (1/a) arctan (u/a) + CExplanation
The given integral can be solved using the substitution method. Let u = a tanθ. Then, du = a sec^2θ dθ. Substituting these values into the integral, we get ∫(a sec^2θ)/(a^2 + a^2 tan^2θ) dθ. Simplifying this expression gives us ∫(a sec^2θ)/(a^2 sec^2θ) dθ. The sec^2θ terms cancel out, leaving us with ∫(1/a) dθ. Integrating this expression gives us (1/a)θ + C. Substituting back θ = arctan(u/a), we get (1/a) arctan (u/a) + C as the final answer.Rate this question:
-
- 7.
∫du/((u(u^2 - a^2))^.5)
- A.
(1/a) arctan (u/a) + C
- B.
Arcsin(u/a) + C
- C.
(1/a)arcsec(u/a) + C
- D.
(1/a) arctan (|u|/a) + C
- E.
(1/a)arcsec(|u|/a) + C
Correct Answer
E. (1/a)arcsec(|u|/a) + CExplanation
The integral given is in the form of ∫du/((u(u^2 - a^2))^.5). To evaluate this integral, we can use the substitution method. Let's substitute u = a secθ, du = a secθ tanθ dθ.
After substitution, the integral becomes ∫(a secθ tanθ)/(a secθ(a sec^2θ - a^2))^.5 dθ. Simplifying this expression, we get ∫(tanθ)/(secθ(a^2 sec^2θ - a^2))^.5 dθ.
Further simplifying, we have ∫(tanθ)/(a secθ)^.5 dθ. Using the trigonometric identity tanθ = sinθ/cosθ and secθ = 1/cosθ, we can rewrite the integral as ∫(sinθ)/(a cosθ)^1.5 dθ.
Now, we can use the substitution method again, letting t = cosθ, dt = -sinθ dθ. The integral becomes ∫(-1)/(a t^1.5) dt.
Integrating this expression, we get (-1/a)∫t^(-1.5) dt = (-1/a)(-2t^(-0.5)) = (2/a√t) + C.
Substituting back t = cosθ, we have (2/a√cosθ) + C. Finally, using the identity secθ = 1/cosθ, we can rewrite the answer as (1/a)arcsec(|u|/a) + C.Rate this question:
-
- 8.
∫csc(u)cot(u)du
- A.
Csc(u) + C
- B.
-csc^2(u) + C
- C.
Sec(u)tan(u) + C
- D.
Tan(u) + C
- E.
-csc(u) + C
Correct Answer
E. -csc(u) + CExplanation
The given integral is ∫csc(u)cot(u)du. By using the derivative of csc(u) which is -csc(u)cot(u), we can see that the correct answer is -csc(u) + C, where C represents the constant of integration.Rate this question:
-
- 9.
∫csc(u)du
- A.
Ln|csc(u) + cot(u)| + C
- B.
Tan^2(u) + C
- C.
-ln|csc(u) + cot(u)| + C
Correct Answer
C. -ln|csc(u) + cot(u)| + CExplanation
The integral of csc(u)du can be found by using the substitution method. Let's substitute v = csc(u) + cot(u). Then, dv = (-csc(u)cot(u) - csc^2(u))du. Rearranging the terms, we have -dv = (csc(u) + cot(u))du. Substituting back into the integral, we get ∫-dv = -∫(csc(u) + cot(u))du. Integrating both sides, we have -v = -ln|csc(u) + cot(u)| + C. Therefore, the correct answer is -ln|csc(u) + cot(u)| + C.Rate this question:
-
- 10.
Cos^2(x) =
- A.
(1 + cos(2x)) / 2
- B.
(1 - cos (2x)) / 2
Correct Answer
A. (1 + cos(2x)) / 2Explanation
A mnemonic device to remember these: It is the solution with the POSITIVE cos(2x) because we started with cos^2(x), not sin^2(x)--did we start with a sine function? Nope, sine^2(x) gives a NEGATIVE on the cosine(2x) because sine is not cosine. Remember, this is not a mathematical proof, just a way to relate things in your head to remember them.Rate this question:
-
- 11.
Sin^2(x) =
- A.
(1 - cos (2x)) / 2
- B.
(1 + cos(2x)) / 2
Correct Answer
A. (1 - cos (2x)) / 2Explanation
The given equation sin^2(x) = (1 - cos (2x)) / 2 is the correct answer. This can be derived using the trigonometric identity sin^2(x) = 1 - cos^2(x). By substituting cos^2(x) with (1 - cos(2x))/2, we get the given equation.Rate this question:
-
- 12.
If the power of the cosine is odd and positive...
- A.
Save a sine factor
- B.
Save a cosine factor
- C.
Convert the remaining factors into sines
- D.
Convert the remaining factors into cosines
Correct Answer(s)
A. Save a sine factor
D. Convert the remaining factors into cosinesExplanation
If the power of the cosine is odd and positive, it means that there is an odd number of cosine factors in the expression. In this case, we save a sine factor because the sine and cosine are complementary functions. Then, we convert the remaining factors into cosines because an odd number of cosines multiplied together will result in a positive value.Rate this question:
-
- 13.
If the power of the cosine is odd and positive,
- A.
Save a sine factor
- B.
Save a cosine factor
- C.
Convert the remaining factors into sines
- D.
Convert the remaining factors into cosines
Correct Answer(s)
B. Save a cosine factor
C. Convert the remaining factors into sinesExplanation
When the power of the cosine is odd and positive, we save a cosine factor because it cannot be converted into a sine. The remaining factors, which are cosines, can be converted into sines using the identity cos^2(x) = 1 - sin^2(x). Therefore, we convert the remaining factors into sines.Rate this question:
-
- 14.
If the powers of both the sine and cosine are even and positive,
- A.
Save a sine factor
- B.
Save a cosine factor
- C.
Use the sine^2 and cosine^2 identities
- D.
Convert the remaining factors into sines
- E.
Convert the remaining factors into cosines
Correct Answer
C. Use the sine^2 and cosine^2 identitiesExplanation
When the powers of both the sine and cosine are even and positive, we can use the sine^2 and cosine^2 identities. These identities state that sine squared plus cosine squared equals 1. By using these identities, we can simplify the expression and eliminate the need for separate sine and cosine factors.Rate this question:
-
- 15.
If the power of the secant is even and positive,
- A.
Save a secant squared factor
- B.
Convert the remaining factors into tangents
- C.
Save a secant-tangent factor
- D.
Convert the remaining factors into secants
- E.
Expand and integrate
Correct Answer(s)
A. Save a secant squared factor
B. Convert the remaining factors into tangents
E. Expand and integrateExplanation
When the power of the secant is even and positive, we can save a secant squared factor. Then, we can convert the remaining factors into tangents. After that, we save a secant-tangent factor and convert the remaining factors into secants. Finally, we expand and integrate the expression.Rate this question:
-
- 16.
If the power of the tangent is odd and positive,
- A.
Save a secant squared factor
- B.
Save a secant-tangent factor
- C.
Convert the remaining factors into tangents
- D.
Convert the remaining factors into secants
- E.
Expand and integrate
Correct Answer(s)
B. Save a secant-tangent factor
D. Convert the remaining factors into secants
E. Expand and integrateExplanation
When the power of the tangent is odd and positive, we can save a secant-tangent factor and convert the remaining factors into secants. This is because the derivative of tangent is secant squared and the derivative of secant is secant-tangent. By saving a secant-tangent factor, we can use it later to simplify the integral. Converting the remaining factors into secants allows us to use the derivative of secant to simplify the integral further. Finally, we expand and integrate the simplified expression to find the solution.Rate this question:
-
- 17.
If there are no secant factors and the power of the tangent is even and positive,
- A.
Convert a tangent-squared factor into a secant-squared factor
- B.
Save a secant-tangent factor
- C.
Save a secant squared factor
Correct Answer
A. Convert a tangent-squared factor into a secant-squared factorExplanation
When there are no secant factors and the power of the tangent is even and positive, we can convert a tangent-squared factor into a secant-squared factor. This means that if we have an expression with a term like tangent squared, we can rewrite it as secant squared. This conversion allows us to simplify the expression and potentially make it easier to work with or solve.Rate this question:
-
- 18.
For integrals involving √(a^2 - u^2),
- A.
U = asinΘ
- B.
U = tanΘ
- C.
U=secΘ
- D.
√(a^2 - u^2) = acosΘ
- E.
√(u^2 - a^2) = atanΘ
Correct Answer(s)
A. U = asinΘ
D. √(a^2 - u^2) = acosΘExplanation
The given answer, u = asinΘ and √(a^2 - u^2) = acosΘ, is correct because when we substitute u = asinΘ into √(a^2 - u^2), we get √(a^2 - (asinΘ)^2) = √(a^2 - a^2sin^2Θ) = √(a^2(1 - sin^2Θ)) = √(a^2cos^2Θ) = acosΘ. Therefore, the answer is valid and provides the correct relationship between u and √(a^2 - u^2).Rate this question:
-
- 19.
For integrals involving √(a^2 + u^2)
- A.
U = asinΘ
- B.
U = atanΘ
- C.
U=asecΘ
- D.
√(a^2 + u^2) = acosΘ
- E.
√(u^2 + a^2) = asecΘ
Correct Answer(s)
B. U = atanΘ
E. √(u^2 + a^2) = asecΘ -
- 20.
For integrals involving √(u^2 - a^2)
- A.
U = asinΘ
- B.
U = atanΘ
- C.
U=asecΘ
- D.
√(u^2 - a^2) = acosΘ
- E.
√(u^2 - a^2) = atanΘ
Correct Answer(s)
C. U=asecΘ
E. √(u^2 - a^2) = atanΘ -
Quiz Review Timeline +
Our quizzes are rigorously reviewed, monitored and continuously updated by our expert board to maintain accuracy, relevance, and timeliness.
-
Current Version
-
Mar 22, 2023Quiz Edited by
ProProfs Editorial Team -
Apr 20, 2010Quiz Created by
Kirakiwibug
- Algebra Quizzes
- Arithmetic Quizzes
- Calculus Quizzes
- Data Handling Quizzes
- Decimal Quizzes
- Discrete Mathematics Quizzes
- Equation Quizzes
- Fraction Quizzes
- Function Quizzes
- Geometry Quizzes
- Graph Quizzes
- Measurement Quizzes
- Multiplication Quizzes
- Number Quizzes
- Percentage Quizzes
- Place Value Quizzes
- Probability Quizzes
- Problem Solving Quizzes
- Ratio Quizzes
- Shape Quizzes
- Statistics Quizzes
Back to top