Trigonometry Practice Problems! Math Trivia Quiz
(35).jpg)
.
- 1.
A photographer points his camera to the top of a building forming an angle of elevation of 50a. If he stands 70 meters from the building, how tall is the building?
- A.
45 meters
- B.
53.6 meters
- C.
83.4 meters
Correct Answer
C. 83.4 metersExplanation
The photographer is standing 70 meters away from the building and the angle of elevation is 50 degrees. To find the height of the building, we can use trigonometry. The tangent of the angle of elevation is equal to the opposite side (height of the building) divided by the adjacent side (distance from the building). So, tan(50) = height/70. Rearranging the equation, we get height = 70 * tan(50) = 83.4 meters. Therefore, the height of the building is 83.4 meters.Rate this question:
-
- 2.
A man is walking along a straight road. He notices the top of a tower makes an angle of 60o with the ground at the point where he is standing. If the height of the tower is h = 15 m, then what is the distance of the man from the tower?
- A.
8.67 meters
- B.
10.8 meters
- C.
6.9 meters
Correct Answer
A. 8.67 metersExplanation
The man is standing at the base of the tower, and he notices that the angle between the ground and the top of the tower is 60 degrees. This forms a right triangle, with the height of the tower as one of the sides and the distance between the man and the tower as the other side. Since we know the height of the tower is 15 meters, and the angle is 60 degrees, we can use trigonometry to find the distance between the man and the tower. By using the sine function (sin(angle) = opposite/hypotenuse), we can calculate the distance to be approximately 8.67 meters.Rate this question:
-
- 3.
A tree is 200 ft tall. If a man is standing 75 ft away from the tree, what is the angle of elevation to the bird he is looking at?
- A.
98°
- B.
56°
- C.
69.5°
Correct Answer
C. 69.5°Explanation
The angle of elevation to the bird can be found using trigonometry. In this case, the opposite side is the height of the tree (200 ft) and the adjacent side is the distance from the man to the tree (75 ft). The angle of elevation can be found using the tangent function: tan(angle) = opposite/adjacent. Therefore, tan(angle) = 200/75. Taking the inverse tangent of both sides, we find that the angle is approximately 69.5°.Rate this question:
-
- 4.
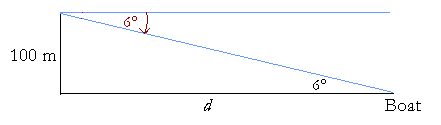
Find the distance of a boat from a lighthouse if the lighthouse is 100 meters tall, and the angle of depression is 6a.
- A.
765
- B.
901
- C.
951.4
Correct Answer
C. 951.4Explanation
The angle of depression is the angle formed between the horizontal line and the line of sight from the observer to the object below. In this case, the boat is below the lighthouse. To find the distance of the boat from the lighthouse, we can use trigonometry. We can use the tangent function, which is opposite over adjacent, to find the distance. The opposite side is the height of the lighthouse (100 meters) and the adjacent side is the distance we want to find. By taking the tangent of the angle of depression (6a), we can solve for the distance. Using this method, we find that the distance is approximately 951.4 meters.Rate this question:
-
- 5.
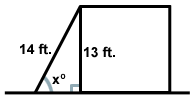
A 14 foot ladder is used to scale a 13 foot wall. At what angle of elevation must the ladder be situated in order to reach the top of the wall?
- A.
68.4 degrees
- B.
21.57 degrees
- C.
42.92 degrees
Correct Answer
A. 68.4 degreesExplanation
Imagine a right triangle formed by the ladder (hypotenuse), the wall (opposite side), and the ground (adjacent side). The angle of elevation is the angle between the ladder and the ground.
We know the opposite side (13 ft) and the hypotenuse (14 ft). The trigonometric function that relates these is the sine function:
sin(angle) = opposite / hypotenuse
sin(x) = 13 / 14
x = arcsin(13/14)
x ≈ 68.2°
Rate this question:
-
- 6.
Find side BC.
- A.
3.92
- B.
4.67
- C.
2.52
Correct Answer
A. 3.92 -
- 7.
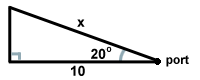
Find the measure of angle θ in the figure. Take h = 15 m and d = 30 m.
- A.
30°
- B.
60°
- C.
25.67°
Correct Answer
C. 25.67°Explanation
The measure of angle θ can be found using the tangent function. Tangent is defined as the ratio of the length of the opposite side to the length of the adjacent side in a right triangle. In this case, the opposite side is h and the adjacent side is d. Therefore, tan(θ) = h/d. Plugging in the given values, tan(θ) = 15/30 = 0.5. To find θ, we can take the inverse tangent (arctan) of 0.5. Using a calculator, we find that θ ≈ 25.67°.Rate this question:
-
- 8.
Find x.
- A.
10.64
- B.
11.9
- C.
9.1
Correct Answer
A. 10.64 -
- 9.
Find the height.
- A.
30.48
- B.
140.55
- C.
173.21
Correct Answer
C. 173.21 -
- 10.
How tall is the flagpole?
- A.
33.32
- B.
19.20
- C.
23.05
Correct Answer
A. 33.32 -
- 11.
A 20-foot ladder is leaning against a wall. The foot of the ladder is 7 feet from the base of the wall. What is the approximate measure of the angle the ladder forms with the ground?
- A.
70.7°
- B.
69.5°
- C.
20.5°
- D.
19.3°
Correct Answer
B. 69.5°Explanation
The approximate measure of the angle the ladder forms with the ground can be determined using trigonometry. In this case, we can use the tangent function, which is defined as the ratio of the length of the opposite side (height of the wall) to the length of the adjacent side (distance from the foot of the ladder to the base of the wall). By taking the arctangent of this ratio, we can find the angle. Using the given values, the tangent of the angle is approximately 0.364, and taking the arctangent of this value gives us an approximate angle of 69.5°.Rate this question:
-
- 12.
Find the missing side.
- A.
20.75
- B.
75.22
- C.
3.32
Correct Answer
B. 75.22 -
- 13.
Find side a.
- A.
8.04
- B.
10.26
- C.
7.50
Correct Answer
B. 10.26 -
- 14.
Find side x if the angle is 45 degrees and the opposite side is 5.
- A.
5
- B.
7
- C.
1/5
Correct Answer
A. 5Explanation
The answer is 5 because in a right triangle, the side opposite to a 45-degree angle is equal to the side adjacent to the angle. Since the opposite side is given as 5, the adjacent side (which is also the value of x) would also be 5.Rate this question:
-
- 15.
Find the angle.
- A.
42.71
- B.
67.38
- C.
22.62
Correct Answer
C. 22.62 -
- 16.
Find the sin A.
- A.
4/5
- B.
3/5
- C.
2/3
- D.
3/4
- E.
5/3
Correct Answer
A. 4/5Explanation
The sine of angle A can be found by dividing the length of the side opposite angle A by the length of the hypotenuse in a right triangle. In this case, the side opposite angle A is 4 and the hypotenuse is 5. Therefore, the sine of angle A is 4/5.Rate this question:
-
- 17.
A wheelchair ramp needs to be pitched at 30o. If the ramp is to access a 25-inch step to a door, what will the length of the ramp be? (Round to the nearest inch.)
- A.
50 inches
- B.
52 inches
- C.
85 inches
- D.
86 inches
Correct Answer
A. 50 inchesExplanation
To determine the length of the ramp, we can use trigonometry. Since the ramp needs to be pitched at 30 degrees, we can use the sine function to find the length. The formula to find the length of the ramp is: length = height / sin(angle). Plugging in the values, we get: length = 25 / sin(30). Using a calculator, we find that sin(30) is 0.5. So, length = 25 / 0.5 = 50 inches. Therefore, the correct answer is 50 inches.Rate this question:
-
Quiz Review Timeline +
Our quizzes are rigorously reviewed, monitored and continuously updated by our expert board to maintain accuracy, relevance, and timeliness.
-
Current Version
-
Nov 05, 2024Quiz Edited by
ProProfs Editorial Team -
Mar 12, 2012Quiz Created by
Tjkim
 Back to top
Back to top