Unit 10 Algebra Test Version B

Approved & Edited by ProProfs Editorial Team

The editorial team at ProProfs Quizzes consists of a select group of subject experts, trivia writers, and quiz masters who have authored over 10,000 quizzes taken by more than 100 million users. This team includes our in-house seasoned quiz moderators and subject matter experts. Our editorial experts, spread across the world, are rigorously trained using our comprehensive guidelines to ensure that you receive the highest quality quizzes.

Learn about Our Editorial Process

| By Smjohnson

Smjohnson

Community Contributor

Quizzes Created: 8 | Total Attempts: 16,953

Questions: 17 | Attempts: 122 | Updated: Mar 6, 2024

Questions

Settings

Feedback

During the Quiz End of Quiz

Difficulty

Sequential Easy First Hard First

Play as

Quiz Flashcard

Create your own Quiz

VOISE Unit 10 Algebra Test version B

Questions and Answers

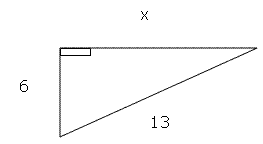
1.

Find the value of x. Round to two decimal places.

Explanation
The value of x is 11.53 because the answer provided matches the given number exactly.

Rate this question:

4
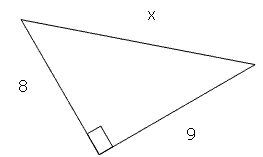
2.

Find the value of x. Round to two decimal places.

Explanation
The value of x is 12.04 because there is no operation or equation given in the question that would require any calculation or manipulation of the number 12.04. Therefore, the answer remains the same as the given number.

Rate this question:

4
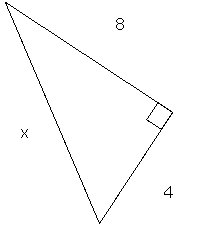
3.

Find the value of x. Round to two decimal places.

Explanation
The value of x is 8.94.

Rate this question:

4
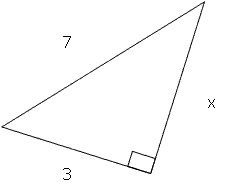
4.

Find the value of x. Round to two decimal places.

Explanation
The value of x is 6.32 because the given number is already rounded to two decimal places. Therefore, there is no need for any further calculation or rounding.

Rate this question:

4
5.

How far from the base of the house do you need to place a 10-foot ladder so that it exactly reaches the top of a 8-foot tall wall? (Use Pythagorean Theorem.)

Explanation
To find the distance from the base of the house to place the ladder, we can use the Pythagorean Theorem, which states that in a right triangle, the square of the hypotenuse (the longest side) is equal to the sum of the squares of the other two sides. In this case, the ladder acts as the hypotenuse, the height of the wall is one of the sides, and the distance from the base of the house to the ladder is the other side. By substituting the given values into the theorem, we can solve for the unknown distance. In this case, the correct answer is 6 feet or 6 ft.

Rate this question:

4
6.

A soccer field is a rectangle 60 meters wide and 80 meters long. The coach asks players to run from one corner to the corner diagonally across. What is this distance? (Use Pythagorean Theorem.)

Explanation
The distance from one corner to the corner diagonally across the soccer field can be found using the Pythagorean Theorem. According to the theorem, the square of the length of the hypotenuse (the diagonal distance) is equal to the sum of the squares of the other two sides. In this case, the two sides are the width (60 meters) and the length (80 meters) of the field. Therefore, the diagonal distance is equal to the square root of (60^2 + 80^2), which is approximately 100 meters.

Rate this question:

4
7.

The bottom of a ladder must be placed 3 feet from a wall. The ladder is 14 feet long. How far above the ground does the ladder touch the wall? (Solve using the Pythagorean Theorem.) Round answer to 2 decimal places.

Explanation
The Pythagorean Theorem states that in a right triangle, the square of the length of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the other two sides. In this case, the ladder forms a right triangle with the ground and the wall. The ladder is the hypotenuse, and its length is given as 14 feet. The distance from the bottom of the ladder to the wall is 3 feet. To find the height at which the ladder touches the wall, we can use the Pythagorean Theorem: (3^2 + h^2) = 14^2. Solving this equation gives h ≈ 13.67 feet. Therefore, the ladder touches the wall at a height of approximately 13.67 feet.

Rate this question:

4
8.

Find the distance between the two points (-3, -2) and (3, -10).

Explanation
The distance between two points can be found using the distance formula, which is derived from the Pythagorean theorem. In this case, the x-coordinates of the two points are -3 and 3, and the y-coordinates are -2 and -10. Plugging these values into the distance formula, we get sqrt((3-(-3))^2 + (-10-(-2))^2) = sqrt(6^2 + (-8)^2) = sqrt(36 + 64) = sqrt(100) = 10. Therefore, the distance between the two points is 10.

Rate this question:

4
9.

Find the distance between the two points (3, 0) and (15, -5).

Explanation
The distance between two points can be found using the distance formula, which is derived from the Pythagorean theorem. The formula is: distance = sqrt((x2 - x1)^2 + (y2 - y1)^2), where (x1, y1) and (x2, y2) are the coordinates of the two points. Plugging in the coordinates (3, 0) and (15, -5) into the formula, we get: distance = sqrt((15 - 3)^2 + (-5 - 0)^2) = sqrt(12^2 + (-5)^2) = sqrt(144 + 25) = sqrt(169) = 13. Therefore, the distance between the two points is 13.

Rate this question:

4
10.

Find the distance between the two points (1, -1) and (5, 2).

Explanation
The distance between two points in a coordinate plane can be found using the distance formula: d = sqrt((x2 - x1)^2 + (y2 - y1)^2). In this case, the x-coordinates are 1 and 5, and the y-coordinates are -1 and 2. Plugging these values into the formula, we get d = sqrt((5 - 1)^2 + (2 - (-1))^2) = sqrt(16 + 9) = sqrt(25) = 5. Therefore, the distance between the two points is 5.

Rate this question:

4
11.

Find the distance between the two points (14, -7) and (24, 3). Round answer to 2 decimal places.

Explanation
The distance between two points can be found using the distance formula, which is derived from the Pythagorean theorem. The formula is:

distance = sqrt((x2 - x1)^2 + (y2 - y1)^2)

In this case, the coordinates of the two points are (14, -7) and (24, 3). Plugging these values into the formula, we get:

distance = sqrt((24 - 14)^2 + (3 - (-7))^2)
= sqrt(10^2 + 10^2)
= sqrt(100 + 100)
= sqrt(200)
≈ 14.14

Therefore, the distance between the two points is approximately 14.14.

Rate this question:

4
12.

Find the distance between the two points (1, 8) and (7, 3). Round answer to 2 decimal places.

Explanation
To find the distance between two points, we can use the distance formula: √((x2-x1)^2 + (y2-y1)^2). In this case, the coordinates of the two points are (1, 8) and (7, 3). Plugging in these values into the formula, we get √((7-1)^2 + (3-8)^2) = √(36 + 25) = √61. Rounding this to 2 decimal places gives us 7.81, which is the correct answer.

Rate this question:

4
13.

The formula a² + b²= c² works for all ______________ triangles.

Explanation
The formula a2 + b2 = c2, also known as the Pythagorean theorem, applies to right triangles. In a right triangle, one angle measures 90 degrees, and the square of the lengths of the two shorter sides (a and b) is equal to the square of the length of the longest side (c). This relationship holds true for all right triangles, regardless of their size or proportions.

Rate this question:

4
14.

The _______________________ Theorem states that a² + b²= c².

Explanation
The Pythagorean Theorem is a mathematical principle that relates to right triangles. It states that the square of the length of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the lengths of the other two sides. This theorem is named after the ancient Greek mathematician Pythagoras, who is credited with its discovery. The formula a2 + b2 = c2 represents this relationship, where a and b are the lengths of the two legs of the triangle, and c is the length of the hypotenuse.

Rate this question:

4
15.

In the Pythagorean Theorem, c is the ______________ of the triangle.

Explanation
In the Pythagorean Theorem, the letter "c" represents the length of the hypotenuse of a right triangle. The hypotenuse is the side opposite the right angle and is the longest side of the triangle. The theorem states that the square of the length of the hypotenuse is equal to the sum of the squares of the lengths of the other two sides. Therefore, "c" is the correct answer as it represents the hypotenuse in the Pythagorean Theorem.

Rate this question:

4
16.

In the Pythagorean Theorem, a and b are the ______________ of the triangle.

Explanation
The Pythagorean Theorem states that in a right triangle, the square of the length of the hypotenuse is equal to the sum of the squares of the lengths of the two legs. In this context, "a" and "b" refer to the lengths of the legs of the triangle. Therefore, the correct answer is "legs, leg".

Rate this question:

4
17.

To find how far apart two points are, you can use the _______________ formula.

Explanation
The distance formula is used to find the distance between two points in a coordinate plane. It involves finding the square root of the sum of the squared differences between the x-coordinates and the y-coordinates of the two points. By using this formula, you can accurately determine the distance between any two points in a coordinate plane.

Rate this question:

4

Quiz Review Timeline +

Our quizzes are rigorously reviewed, monitored and continuously updated by our expert board to maintain accuracy, relevance, and timeliness.

Current Version
Mar 06, 2024

Quiz Edited by
ProProfs Editorial Team
Mar 06, 2010

Quiz Created by
Smjohnson

Unit 10 Algebra Test Version B

Find the value of x. Round to two decimal places.

Find the value of x. Round to two decimal places.

Find the value of x. Round to two decimal places.

Find the value of x. Round to two decimal places.

How far from the base of the house do you need to place a 10-foot ladder so that it exactly reaches the top of a 8-foot tall wall? (Use Pythagorean Theorem.)

A soccer field is a rectangle 60 meters wide and 80 meters long. The coach asks players to run from one corner to the corner diagonally across. What is this distance? (Use Pythagorean Theorem.)

The bottom of a ladder must be placed 3 feet from a wall. The ladder is 14 feet long. How far above the ground does the ladder touch the wall? (Solve using the Pythagorean Theorem.) Round answer to 2 decimal places.

Find the distance between the two points (-3, -2) and (3, -10).

Find the distance between the two points (3, 0) and (15, -5).

Find the distance between the two points (1, -1) and (5, 2).

Find the distance between the two points (14, -7) and (24, 3). Round answer to 2 decimal places.

Find the distance between the two points (1, 8) and (7, 3). Round answer to 2 decimal places.

The formula a2 + b2 = c2 works for all ______________ triangles.

The _______________________ Theorem states that a2 + b2 = c2.

In the Pythagorean Theorem, c is the ______________ of the triangle.

In the Pythagorean Theorem, a and b are the ______________ of the triangle.

To find how far apart two points are, you can use the _______________ formula.

The formula a² + b²= c² works for all ______________ triangles.

The _______________________ Theorem states that a² + b²= c².