Examen De Probabilidad Y Estadística Unidad 3

Reviewed by Editorial Team
The ProProfs editorial team is comprised of experienced subject matter experts. They've collectively created over 10,000 quizzes and lessons, serving over 100 million users. Our team includes in-house content moderators and subject matter experts, as well as a global network of rigorously trained contributors. All adhere to our comprehensive editorial guidelines, ensuring the delivery of high-quality content.
Learn about Our Editorial Process
| By Denisse Blanco
D
Denisse Blanco
Community Contributor
Quizzes Created: 1 | Total Attempts: 233
| Attempts: 233
Quiz Flashcard
SettingsSettings
Please wait...
  • 1/15 Questions

    El conjunto M por extensión es:  M={5,10,15,20,25,30,…} ¿cuál es el conjunto M por comprensión?

    • M= {ð‘¥â”‚ð‘¥ ð‘’ð‘  ð‘¢ð‘› múltiplo de 5}
    • M= {ð‘¥â”‚ð‘¥ ð‘’ð‘  ð‘¢ð‘› número primo}
    • M= {ð‘¥â”‚ð‘¥ ð‘’ð‘  ð‘¢ð‘› número real}
    • M= {ð‘¥â”‚ð‘¥ ð‘’ð‘  ð‘¢ð‘› número racional}
Please wait...
About This Quiz

Examen de Probabilidad y estadística para sexto semestre.

Examen De Probabilidad Y Estadística Unidad 3 - Quiz

Quiz Preview

  • 2. 

    ¿Que diagrama de Venn representa correctamente la operación (A U B) - C?

    • Option 2

    • Option 3

    • Option 1

    • Option 4

    Correct Answer
    A. Option 1
    Explanation
    Option 1 represents the correct Venn diagram for the operation (A U B) - C. In this diagram, the sets A and B are represented by two overlapping circles, and the set C is represented by a separate circle. The shaded region inside A and B but outside of C represents the elements that belong to either A or B but not to C. This corresponds to the operation (A U B) - C, which means taking the union of A and B and then removing the elements in C.

    Rate this question:

  • 3. 
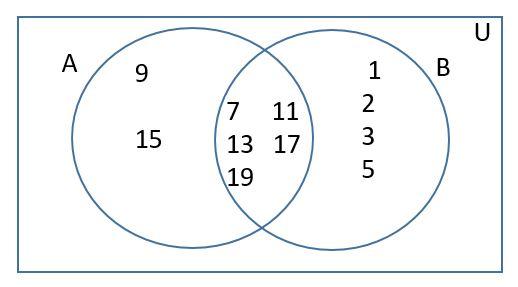
    Los elementos que corresponden a la operación: ð´ ∩ ðµ  en el siguiente diagrama de Venn son: - ProProfs

    Los elementos que corresponden a la operación: ð´ ∩ ðµ  en el siguiente diagrama de Venn son:

    • 7,9,11,13,17,19

    • 7,11,13,17,19

    • 1,2,3,5,7,11,13,17,19

    • 7,11,13,17,19

    Correct Answer
    A. 7,11,13,17,19
    Explanation
    The correct answer is 7, 11, 13, 17, 19 because these elements are the ones that belong to both sets A and B, represented by the intersection of the two circles in the Venn diagram.

    Rate this question:

  • 4. 

    En el jardin de niños campanita, se tienen 3 pelotas rojas, 2 azules, 2 amarillas y 1 verde en una caja de cartón. Rosalía, Elías y Renata toman, cada uno, una pelota roja y juegan con ellas en el recreo. Entonces el conjunto complemento de este caso estaría formado por 2 pelotas amarillas, 1 azul y 1 verde

    • Verdadero

    • Falso

    Correct Answer
    A. Falso
    Explanation
    The statement is false because the complement of the set of red balls that Rosalía, Elías, and Renata took would be the remaining balls in the box, which are 2 yellow balls, 1 blue ball, and 1 green ball.

    Rate this question:

  • 5. 

    Es una operación entre dos conjuntos, de tal modo que se forma otro conjunto con todos los pares ordenados posibles. 

    • Producto Cartesiano

    • Conjunto potencia

    • Unión de conjuntos

    • Conjunto complemento

    Correct Answer
    A. Producto Cartesiano
    Explanation
    The given correct answer is "Producto Cartesiano" because it is a mathematical operation between two sets that creates a new set containing all possible ordered pairs. This operation is also known as the Cartesian product, which is commonly used in set theory and combinatorics.

    Rate this question:

  • 6. 
    ¿Qué operación representa el siguiente diagrama de Venn? - ProProfs

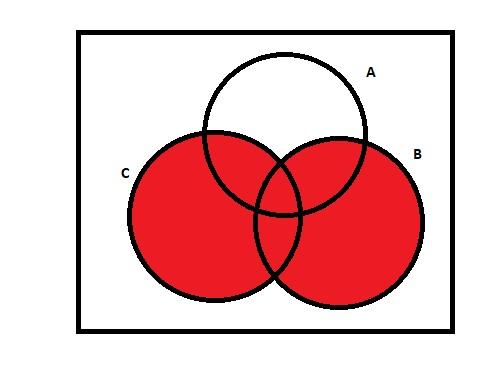
    ¿Qué operación representa el siguiente diagrama de Venn?

    • A ∩ B

    • (A ∩ B) ∪ (A ∩ C)

    • A ∩ (B ∪ C)

    • (A ∩ B) ∪ C

    Correct Answer
    A. A ∩ (B ∪ C)
    Explanation
    The correct answer is A ∩ (B ∪ C) because the Venn diagram shows the intersection of set A with the union of sets B and C. This means that it represents the elements that are common to both set A and either set B or set C, or both.

    Rate this question:

  • 7. 

    En la aerolínea Europa se proporciona a las azafatas un uniforme que consta de 4 faldas, 4 blusas y 3 pares de zapatos distintos. Se considera que utilizarán un uniforme distinto para cada día, y que todas las prendas son de colores coordinados ¿de cuantas formas diferentes puede vestirse una sobrecargo, sin repetir un atuendo?

    • 11

    • 48 

    • 30

    • 19

    Correct Answer
    A. 48 
    Explanation
    There are 4 skirts, 4 blouses, and 3 pairs of different shoes available. To find the number of different outfits a flight attendant can wear without repeating an outfit, we multiply the number of options for each item together. So, the total number of different outfits is 4 (skirts) x 4 (blouses) x 3 (pairs of shoes) = 48. Therefore, a flight attendant can dress in 48 different ways without repeating an outfit.

    Rate this question:

  • 8. 

    Hay 3 dados y 4 monedas ¿Cuál es la cardinalidad del espacio muestral que se forma al tirar una moneda, después un dado, después la otra moneda, otro dado, una moneda, un dado y finalmente una moneda?

    • 1728

    • 3456

    • 10368

    • 3450

    Correct Answer
    A. 3456
    Explanation
    The cardinality of the sample space can be found by multiplying the number of outcomes for each event. In this case, there are 2 outcomes for each coin toss (2 monedas), and 6 outcomes for each dice roll (3 dados). Therefore, the total number of outcomes is 2 * 2 * 6 * 3 * 2 * 6 * 2 = 3456.

    Rate this question:

  • 9. 

    Si se desea acomodar a 6 alumnos en una fila del salón de clases, entonces, los alumnos se pueden sentar de ________ maneras diferentes. 

    Correct Answer
    720
    Explanation
    There are 6 students and they can be arranged in a line. The number of ways to arrange them can be calculated using the formula for permutations, which is n! (n factorial). In this case, n=6, so the number of ways to arrange the students is 6! = 6 x 5 x 4 x 3 x 2 x 1 = 720.

    Rate this question:

  • 10. 

    Una pareja de recién casados desea salir en un viaje de vacaciones, la pareja consultó dos agencias de viajes, las cuales les dieron las siguientes opciones: Agencia 1: Realizar un tour a Europa o un viaje por el Caribe Mexicano. Agencia 2: Conocer Machu Pichu, realizar un viaje por los pueblos mágicos de México o visitar Nueva York. Independientemente de que pueden elegir una de las dos agencias , ¿cuál es la probabilidad de que su viaje sea por algún lugar de México?

    • 0.3333

    • 0.50

    • 0.1666

    • 0.8333

    Correct Answer
    A. 0.8333
    Explanation
    The probability of their trip being in Mexico can be determined by calculating the number of options that involve Mexico and dividing it by the total number of options given by both agencies. In this case, Agency 1 offers a trip to the Caribbean Mexican and Agency 2 offers a trip to the magical towns of Mexico. Therefore, there are two options out of a total of three options that involve Mexico. Dividing 2 by 3 gives us 0.6667, which is equivalent to 0.8333 when rounded to four decimal places.

    Rate this question:

  • 11. 

    Hay 6 personas elegibles para formar un Comité Directivo compuesto de un presidente, un secretario y un tesorero. ¿Cuántas maneras distintas existen para conformar dicho comité?

    • 120

    • 60

    • 720

    • 310

    Correct Answer
    A. 120
    Explanation
    There are 6 eligible people to form a committee with 3 positions: president, secretary, and treasurer. To find the number of ways to form the committee, we use the concept of permutations. The number of ways to select the president is 6 (as any of the 6 people can be chosen), then the number of ways to select the secretary is 5 (as there are 5 remaining people), and finally the number of ways to select the treasurer is 4 (as there are 4 remaining people). Therefore, the total number of ways to form the committee is 6 x 5 x 4 = 120.

    Rate this question:

  • 12. 

    De la ciudad A a la ciudad B, se puede ir mediante 2 buses o 3 trenes. De la ciudad B a la ciudad C se puede ir mediante 2 barcos, 2 trenes o 3 aviones. ¿De cuántas formas se puede ir de la ciudad A a la ciudad C, pasando por B?

    • 18

    • 35

    • 12

    • 72

    Correct Answer
    A. 35
    Explanation
    There are two possible routes from city A to city B (2 buses or 3 trains) and three possible routes from city B to city C (2 boats, 2 trains, or 3 planes). To calculate the total number of ways to go from city A to city C, passing through city B, we multiply the number of routes from A to B (2) with the number of routes from B to C (3), giving us a total of 6. However, we need to subtract the duplicate routes, which are the routes that involve taking both buses and boats or both trains and planes. Since there is only one such duplicate route, the final answer is 6 - 1 = 5.

    Rate this question:

  • 13. 

    En un colegio, la probabilidad de que un alumno consuma mayonesa, es de 65%, la probabilidad de que consuma ketchup es de 70%, y la probabilidad de que consuma mayonesa y ketchup es de 55%. ¿Cuál es la probabilidad de que un alumno consuma mayonesa, dado que consume ketchup?

    • 0.7857

    • 70%

    • .8461

    • 0.9285

    Correct Answer
    A. 0.7857
    Explanation
    The correct answer is 0.7857. This can be calculated using the formula for conditional probability. The probability of consuming mayonnaise given that the student consumes ketchup is equal to the probability of consuming both mayonnaise and ketchup divided by the probability of consuming ketchup. In this case, the probability of consuming both mayonnaise and ketchup is 55%, and the probability of consuming ketchup is 70%. Therefore, the probability of consuming mayonnaise given that the student consumes ketchup is 55%/70% = 0.7857.

    Rate this question:

  • 14. 

    En una urna se encuentran 8 esferas de color azul, 10 amarillas y 6 moradas. Se requiere realizar el experimento de extracciones de la urna sin reemplazo. ¿Cuál es la probabilidad de que al realizar dos extracciones (una a la vez) se obtengan dos esferas de color amarillo?

    • 0.1630

    • 0.1034

    • 0.1635

    • 0.1030

    Correct Answer
    A. 0.1630
    Explanation
    The probability of obtaining two yellow spheres can be calculated by dividing the number of favorable outcomes (choosing 2 yellow spheres out of 10) by the total number of possible outcomes (choosing any 2 spheres out of the total 24 spheres). The calculation is as follows: (10/24) * (9/23) = 0.1630.

    Rate this question:

Quiz Review Timeline (Updated): Jul 22, 2024 +

Our quizzes are rigorously reviewed, monitored and continuously updated by our expert board to maintain accuracy, relevance, and timeliness.

  • Current Version
  • Jul 22, 2024
    Quiz Edited by
    ProProfs Editorial Team
  • May 28, 2020
    Quiz Created by
    Denisse Blanco
Back to Top Back to top
Advertisement