Types of Triangles Lesson: Properties, Theorems, and Formulas

Lesson Overview

• What Is a Triangle?
• How Are Triangles Classified
• What Are the Properties of Different Types of Triangles?
• What Are the Important Triangle Theorems?
• How Do You Calculate the Area and Perimeter of Triangles
• Conclusion

Students often struggle with solving geometric problems because they can't easily identify the type of triangle involved. This confusion affects their understanding of angles, sides, and key theorems. In this types of triangles lesson, you'll explore their properties, classifications, and formulas-building a solid foundation for mastering geometry.

What Is a Triangle?

A triangle is a closed two-dimensional shape with three straight sides and three angles. It is one of the most fundamental geometric figures in mathematics and plays a key role in geometry, trigonometry, and real-world applications like engineering, design, and architecture.

Basic Properties of a Triangle

• It has three vertices (corners).
• The sum of all interior angles in a triangle is always 180 degrees.
• The length of any side must be less than the sum of the other two sides (Triangle Inequality Theorem).
• Triangles are classified based on their sides and angles.

Components of a Triangle

Component	Description	Example
Side	A straight line segment joining two vertices	AB, BC, or AC
Angle	Formed where two sides meet	∠A, ∠B, ∠C
Vertex	A point where two sides intersect	A, B, C
Base	Often the side considered as the foundation	Any one side, by context
Height	Perpendicular distance from base to opposite vertex	Depends on triangle orientation

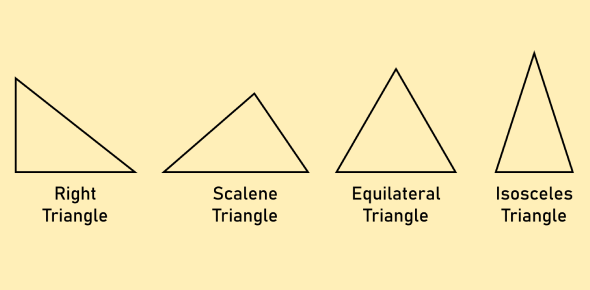
How Are Triangles Classified

Triangles are classified into different types based on the characteristics of their sides and angles. This classification is fundamental in geometry, as it allows us to understand the unique properties and relationships within each type of triangle.

Classification by Sides

1. Equilateral Triangle
   An equilateral triangle has all three sides of equal length, making it perfectly symmetrical. As a result of this equal side length, all three interior angles in an equilateral triangle are also equal, each measuring exactly 60 degrees. This type of triangle is often used in problems involving symmetry and regularity in geometric figures.
   
2. Isosceles Triangle
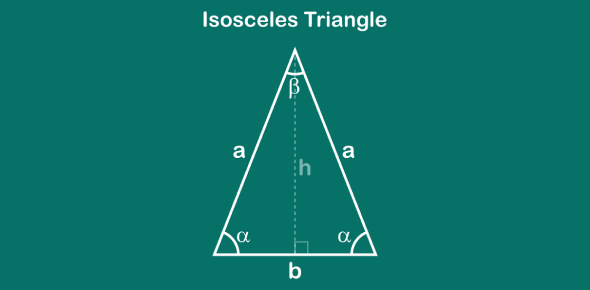
   In an isosceles triangle, two sides are of equal length, and the third side is different. The angles opposite the two equal sides are also equal. This means that if you know one of the angles opposite the equal sides, you can easily find the other. Isosceles triangles are frequently encountered in geometric proofs and constructions because of their properties of symmetry and balance.
   
3. Scalene Triangle
   A scalene triangle is one in which all three sides have different lengths, and consequently, all three angles are different as well. Scalene triangles do not have any lines of symmetry and are often used in problems that require analysis of general properties of triangles without specific constraints of equality in sides or angles.

Classification by Angles

1. Acute Triangle
   An acute triangle is characterized by having all three interior angles measuring less than 90 degrees. This means that the triangle is "sharp" or "pointed," and the sum of its angles still equals 180 degrees. Acute triangles can vary in their side lengths, so they can also be equilateral, isosceles, or scalene.

2. Right Triangle
   A right triangle has one of its angles exactly equal to 90 degrees, making it a key shape in trigonometry and various practical applications, such as construction and navigation. The side opposite the right angle is called the hypotenuse, and the other two sides are referred to as the legs. Right triangles are the basis for the Pythagorean Theorem, which is a fundamental principle in geometry.

3. Obtuse Triangle
   An obtuse triangle contains one angle that is greater than 90 degrees. This type of triangle appears "stretched" in one direction, with one of its angles being wider than a right angle. Obtuse triangles have specific properties that distinguish them from acute and right triangles, particularly in the way they are used in geometric proofs and constructions.

Take These Quizzes

What Are the Properties of Different Types of Triangles?

Triangles can be classified based on their sides or angles, and each type has unique properties. Understanding these properties helps in identifying triangles, solving problems, and applying geometric theorems effectively.

Classification Based on Sides

Equilateral Triangle

• All sides are equal: AB ≅ BC ≅ AC
• All angles are equal: ∠A = ∠B = ∠C = 60°
• Symmetry: Has 3 lines of symmetry
• Properties: Regular polygon; all internal angles measure 60°

Isosceles Triangle

• Two sides are equal: AB ≅ AC
• The angles opposite equal sides are equal: ∠B = ∠C
• Properties: Has at least one line of symmetry
• Special Case: An equilateral triangle is also isosceles

Scalene Triangle

• No sides are equal: AB ≠ BC ≠ AC
• No angles are equal: ∠A ≠ ∠B ≠ ∠C
• Properties: No symmetry; angle and side measures are all different

Classification Based on Angles

Acute Triangle

• All angles are less than 90°: ∠A < 90°, ∠B < 90°, ∠C < 90°
• Properties: Can be scalene, isosceles, or equilateral

Right Triangle

• Has one right angle: ∠C = 90°
• Sides: The side opposite the right angle is the hypotenuse
• Pythagorean relation: a² + b² = c², where c is the hypotenuse
• Properties: Can be scalene or isosceles, but never equilateral

Obtuse Triangle

• Has one angle greater than 90°: ∠A > 90°
• The other two angles are acute: ∠B < 90°, ∠C < 90°
• Properties: Can be scalene or isosceles, but never equilateral

Take These Quizzes

What Are the Important Triangle Theorems?

Triangle theorems define essential rules about angles, sides, and relationships within and between triangles. These theorems are fundamental in geometry and are widely used to solve problems involving measurements, proofs, and constructions.

Triangle Sum Theorem

Statement:
In any triangle, the sum of the three interior angles is always equal to 180°.

Formula:
∠A + ∠B + ∠C = 180°

Application:
If ∠A = 70° and ∠B = 60°, then ∠C = 180° − (70° + 60°) = 50°

Exterior Angle Theorem

Statement:
An exterior angle of a triangle is equal to the sum of its two remote interior angles.

Formula:
∠Exterior = ∠Interior₁ + ∠Interior₂

Example:
If ∠A = 50° and ∠B = 60°, then the exterior angle at ∠C = 50° + 60° = 110°

Isosceles Triangle Theorem

Statement:
If two sides of a triangle are congruent, then the angles opposite those sides are congruent.

Converse:
If two angles are congruent, then the sides opposite them are also congruent.

Symbolically:
If AB ≅ AC, then ∠B ≅ ∠C

Pythagorean Theorem (Right Triangles Only)

Statement:
In a right triangle, the square of the hypotenuse is equal to the sum of the squares of the other two sides.

Formula:
c² = a² + b²

Application:
If a = 3 and b = 4, then c = √(3² + 4²) = √(9 + 16) = √25 = 5

Triangle Inequality Theorem

Statement:
The sum of the lengths of any two sides of a triangle must be greater than the length of the third side.

Inequalities:
AB + BC > AC
AB + AC > BC
AC + BC > AB

Example:
Sides 3, 4, and 5 form a triangle because 3 + 4 > 5, 3 + 5 > 4, and 4 + 5 > 3

Triangle Congruence Theorems

These theorems determine when two triangles are congruent (same shape and size).

SSS (Side–Side–Side)

If AB ≅ DE, BC ≅ EF, and AC ≅ DF, then △ABC ≅ △DEF

SAS (Side–Angle–Side)

If AB ≅ DE, ∠B ≅ ∠E, and BC ≅ EF, then △ABC ≅ △DEF

ASA (Angle–Side–Angle)

If ∠A ≅ ∠D, AB ≅ DE, and ∠B ≅ ∠E, then △ABC ≅ △DEF

AAS (Angle–Angle–Side)

If ∠A ≅ ∠D, ∠B ≅ ∠E, and BC ≅ EF, then △ABC ≅ △DEF

HL (Hypotenuse–Leg)

In right triangles, if hypotenuse and one leg are congruent, then the triangles are congruent.

Triangle Similarity Theorems

These describe when two triangles are similar (same shape, different size).

AA (Angle–Angle)

If two angles of one triangle are equal to two angles of another, then the triangles are similar.
∠A ≅ ∠D and ∠B ≅ ∠E → △ABC ∼ △DEF

SSS (Side–Side–Side) Similarity

If AB/DE = BC/EF = AC/DF, then △ABC ∼ △DEF

SAS (Side–Angle–Side) Similarity

If AB/DE = AC/DF and ∠A ≅ ∠D, then △ABC ∼ △DEF

Take These Quizzes

How Do You Calculate the Area and Perimeter of Triangles

Calculating the area and perimeter of triangles is fundamental in geometry, and it varies depending on the type of triangle and the information provided. Below, we'll explore the methods for calculating both the area and the perimeter of different types of triangles.

1. Calculating the Perimeter of a Triangle

The perimeter of a triangle is the sum of the lengths of its sides. This calculation is straightforward and applies to all types of triangles, whether they are equilateral, isosceles, or scalene.

• Formula
  Perimeter=a+b+c
  where a, b, and c are the lengths of the three sides of the triangle.
  
• Example
  Suppose you have a triangle with side lengths of 5 cm, 7 cm, and 10 cm. The perimeter would be calculated as:
  Perimeter=5 cm+7 cm+10 cm=22 cm
  
  This formula works universally for all triangles, as it simply adds the lengths of the sides together.

2. Calculating the Area of a Triangle

The method for calculating the area of a triangle depends on the information available about the triangle. The most common methods include using the base and height, using Heron's formula, and using the sine function for non-right triangles.

• Area Using Base and Height
  For any triangle, if the base and the corresponding height are known, the area can be calculated using the following formula
  
  • Formula
    Area=1/2×Base×Height
    
  • Explanation
    In this formula, the base is any one side of the triangle, and the height is the perpendicular distance from the base to the opposite vertex. This method works for all types of triangles.
    
  • Example
    Suppose a triangle has a base of 8 cm and a height of 5 cm. The area would be calculated as:
    Area=12 × 8 cm × 5 cm=20 cm²

• Area Using Heron's Formula
  Heron's formula is particularly useful when the lengths of all three sides of the triangle are known, but the height is not. It allows you to calculate the area without needing to know the height.
  
  • Formula:
    Area=s×(s−a)×(s−b)×(s−c)
    where s is the semi-perimeter of the triangle, and a, b, and c are the side lengths.
    
    The semi-perimeter sss is calculated as
    s= a+b+c2
    
  • Explanation
    Heron's formula is useful for any type of triangle, including scalene triangles where the sides are of different lengths.
    
  • Example
    Suppose a triangle has sides of 7 cm, 8 cm, and 9 cm. First, calculate the semi-perimeter
    s= 7 cm + 8 cm + 9 cm2
    s= 12 cm
    
    Then, use Heron's formula to calculate the area
    
    Area= 12cm×(12cm−7cm)×(12cm−8cm)×(12cm−9cm)​
    Area= 12×5×4×3​ = 720 ≈ 26.83 cm²

• Area of a Right Triangle
  For right triangles, the area can be calculated more easily by treating one of the legs as the base and the other leg as the height.
  
  • Formula
    Area=12 × Leg₁ × Leg₂
    where Leg₁​ and Leg₂​ are the lengths of the two legs of the right triangle.
    
  • Example
    If a right triangle has legs of lengths 6 cm and 8 cm, the area is:
    Area=12 × 6 cm × 8 cm=24 cm²

• Area Using Trigonometry (For Non-Right Triangles)
  If you know two sides of a triangle and the included angle (the angle between those two sides), you can calculate the area using trigonometry.
  
  • Formula
    Area=12 × a × b × sin⁡(C)
    where a and b are the lengths of the two sides, and C is the included angle.
    
  • Explanation
    This formula is particularly useful for oblique triangles (triangles that are not right-angled).
    
  • Example
    Suppose a triangle has sides of 7 cm and 10 cm, with an included angle of 30 degrees.
    
    The area is
    Area = 12  × 7cm × 10cm × sin(30^∘)
    Area = 12 × 7 × 10 × 0.5 = 17.5cm²

Take These Quizzes

Conclusion

In this lesson on the types of triangles, we've explored the fundamental geometric properties and classifications that define this essential shape. From understanding what constitutes a triangle to examining the various types-equilateral, isosceles, and scalene, as well as right, acute, and obtuse triangles-we've covered the key characteristics that differentiate each type. We've also learned about the important theorems related to triangles, such as the Pythagorean theorem, and learned how to calculate the area and perimeter of different triangles using various methods.